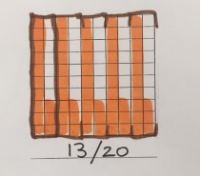
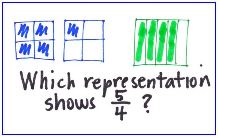
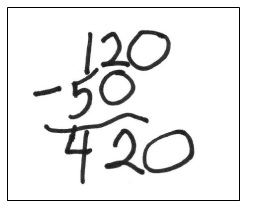
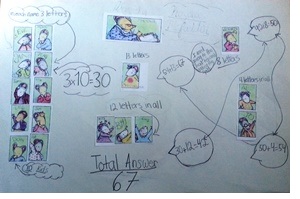
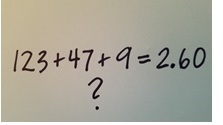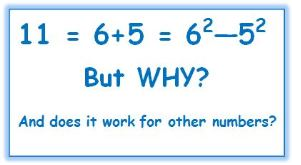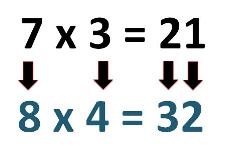When half of the fifth graders selected 0.45 as the smallest of 0.8, 0.45, and 0.125, I had my work cut out for me. I planned a lesson that went wonderfully. Those are the days that remind me of how good it is to be a teacher.
Marilyn BurnsFebruary 2, 2025