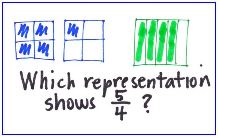
The fourth graders I’ve been working with this year have recently been learning about fractions. Last week I began a lesson by drawing two representations on the board (as shown above) and writing a question.
I gave the students about five minutes to do a “quick write” to answer the question and explain their thinking. I collected their papers without commenting on them, and then I began the discussion.
The conversation lasted for about 45 minutes, and a variety of arguments emerged. It was obvious to some students that the blue representation was 5/4, but others weren’t sure about that.
- Some students explained that each small blue shaded square was one-fourth and there were five of them, so that was 5/4.
- Some reasoned that since the green representation was 4/5, the other had to be 5/4.
- One girl argued vehemently that the representation on the left, the blue one, was 5/8, not 5/4, explaining that there were eight squares and five of them were shaded in.
- A boy countered by arguing that if the two blue squares were touching to make one rectangle, it would be 5/8, but they didn’t.
- Others thought that both of their arguments could make sense.
- It seemed clear to most of the students that the green representation on the right was 4/5, which caused some to think that maybe neither representation showed 5/4.
It was fascinating. I’m not sure the photo below showing the board work is useful, but students came up and gave different ways to explain their thinking and to convince those who disagreed with their position.

Some students changed their thinking during the discussion, a few more than one time. It was an amazing discussion because everyone was involved in asking questions and making comments. The students listened respectfully to one another. The conversation was testimony to the work that their regular teacher, Sara, has done to build a classroom climate that supports this sort of discourse.
Ending the Discussion
Math class is the hour before lunch, and after the conversation had gone on for almost 45 minutes, one boy announced, “I’m tired and I’m hungry.” I could see his point and said that it was a good time to stop.
Then, to get a sense of where the students were in their thinking, I asked them to put their heads down so they could vote privately. More students voted for the blue correct representation (some shifting from their initial written work), some voted for the green representation, a few voted for neither representation showing 5/4, and a few voted that they weren’t sure yet.
My Teaching Dilemma
The students wanted to know what the “real” answer was. And there I was facing one of those teaching decisions for which I’m not sure there’s a right answer. Should I tell the correct answer? Would that nurture or shut down thinking? How do I honor the students’ efforts to make sense and communicate, while also being true to the mathematics?
I didn’t want to foster misconceptions. I saw the need to emphasize the importance of identifying what is the whole. The girl arguing for 5/8 considered the two squares as one whole, as if it were a chocolate bar scored into eight pieces, each 1/8. Others thought (as I did) that each of the two squares was a whole divided into four pieces, each 1/4. Defining the whole and keeping your sight on it is extremely important.
But I made the decision, with Sara’s agreement, not to resolve the issue. I told the students that I’d give them more time to think about the problem and that we’d come back to it.
Revisiting the Lesson
The lesson was on Thursday. On Monday, Sara asked them to revisit the problem. First they revisited the board work, which she had saved. Then Sara asked them to draw their own representations for 5/4, encouraging them to show multiple representations. She organized a silent ten-minute gallery walk for students to look at each other’s work. Before they began to look at the papers, she directed them to think about what they learned, were unsure about, or were wondering. She distributed post-it notes so that students could leave their comments as they read what each other had written.

This representation shows the student’s comfort with using a mixed number for 5/4.

This student struggled with showing 5/4 with triangles. The class would benefit from exploring dividing a triangle into equal parts.
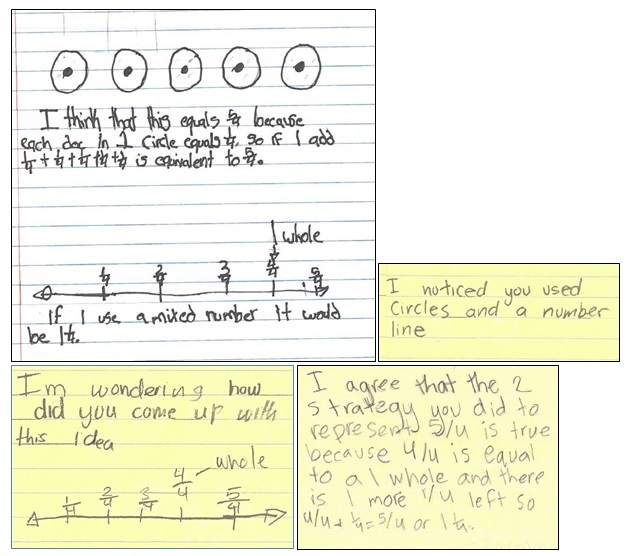
This student shows comfort with using the representation of a number line and with mixed numbers. But I’m not sure why he thinks the dots in the circles show fourths.
P.S. Before teaching the class, I wanted to be prepared in case the question didn’t spark any controversy. My backup was to ask them a question I was introduced to by Phil Daro: Which of the two fractions—5/4 or 4/5—is closer to 1? I’ll save that for another lesson.
P.P.S. Students write reflections at the end of each math class. One boy, who is prone to confrontations with students during recess, made my heart sing by writing the following:

P.P.P.S. I got the idea for this lesson at the NCSM 2016 meeting this April when I attended Susan Jo Russell’s session titled Productive Lingering: Elementary Students Learn About Structure of the Operations Through Representation-Based Argument. The description read: “Too often representations are passed over quickly, as if what they illustrate is obvious. What does it look like when grades 2–5 students spend focused time creating, comparing, and analyzing representations in order to understand the structure and behavior of the operations? Video examples from our research will be used to examine these questions.” I’m pleased that I attended Susan Jo’s session (as I always am after hearing her speak).

Thanks for sharing this experience. There’s so much here to think about. I love how this one, accessible question sparked so much debate and inspired such meaningful mathematical thinking. We don’t always need a big dog-and-pony show. Often something much simpler will accomplish the same goals. Also I like how you and the classroom teacher took your time and let this all unfold. Too often we rush on to the next question before we’ve given the last one its full due.
Given what they now know, I’m curious to know how the class will respond to Daro’s question about which is closer to 1. I hope you’ll share those results in a follow-up post.
I’m going to class tomorrow and will ask them Phil Daro’s questions about which is closer to 1, 4/5 or 5/4. I’m not sure how they’ll respond. One of the goals I have when planning instruction is to make lessons accessible to all students while also having the potential to offer a challenge. That’s not always easy. As I think about Phil Daro’s question, I worry that there are a few students who will be mathematically perplexed. At least at first. But that reminds me that students often prove my assumptions wrong, so I’ll see what happens. My goal is to challenge them with kindness and encouragement, and to help them stay open to learning from me and their classmates. When I was a math major in college, preparing to be a math teacher, I remember feeling completely lost at times in classes when a well-meaning professor was filling the board with mathematical whatever. It took a lot of energy to stay engaged, not get discouraged, and not to feel demeaned. I think I learned as much about making classrooms safe places to learn as I did about the mathematics I was expected to learn. That is, I think I’m a better math teacher in many ways from having experienced what it feels like not to know what’s going on. But I’m not a subscriber to the school of hard knocks when teaching kids. So . . . as I ponder the question about whether 4/5 or 5/4 is closer to 1, I’m thinking about how to help them embrace “not understanding” as “not understanding yet.” Stay tuned. And if you try the question with students in your school, I’d love to hear about what happens.
Is this why you are reluctant: for kids who don’t yet feel comfortable with the idea of fractions, asking the next question might seem to be piling on complexity?
That makes me think of a comment from Piper Harris: “we are allowed to use math that we don’t yet understand.” I wonder how elementary teachers can communicate that to kids?
I was reluctant to give the answer in this instance because I didn’t think that the answer would promote understanding. I thought it would shut down the conversation rather than keeping it open. I don’t mean to imply that I never give answers, but in this situation, I didn’t think it would be productive. I may be wrong of course, for some or all of the students — making instructional decisions is never easy or simple.
This post is a good illustration of the question: “what is the reference unit?” Properly specified, any of the choices could be a representation of 5/4.
One thing I found very interesting: in the student work highlighted, none chose to use a rectangle representation. Instead, they used circles with pie wedges and number lines. The number line explicitly identifies the reference unit, while a complete circle seems to provide an obvious and intuitive reference unit.
Yes, circles have the advantage of the whole being defined by its shape and, therefore, many parts of the whole (for sure, halves and fourths) also being recognizable by their shape, even if the whole circle isn’t there. Not so with cutting rectangles where the whole must be clearly referenced to quantify any part. And number lines are a completely different way to represent fractions, sort of a combination of an ordinal model and a length model. All this points to the importance of students having experience with different models for fractions — as parts of wholes and also parts of sets. I love teaching fractions and the wonderful conversations that they can spark. Thanks for your comment.
Dr Cathy Bruce from Trent University in Peterborough, Ontario, Canada recently completed a fraction inquiry research project with the results being published on Edugains.ca from the Ontario Ministry of ED. I was part of the inquiry and one recommendation that came out of it was to avoid using circles as representations in the early years up to around grade 5/6. Some of the reasons being that they are hard to draw accurately by hand (for the whole representation), they are hard to partition accurately for odd number fractions (5ths, 7ths etc) and that if students are shown circles as the first representation (the study stated this is especially true for girls) they will revert to using the circle almost always even after being shown other ways like rectangles, number lines, pattern blocks etc. that may be easier and more efficient to partition. Also when they do use circles it often creates misconceptions with having to have equal area parts because of the difficulty partitioning them. This was research she shared with us but I don’t believe was done by her. Her research concentrated more on how students develop fraction understanding, what tasks should be used and proper terminology etc. Its well worth the read on edugains.ca (Fraction Pathways). Our board has been focusing on having our teachers work with multiply representations and visual models and avoid circles until others have been shown and used. We were also shared info about how in Japan they focus on three visual models: rectangle, number line and volume model. With the number line being there main focus and that they didn’t understand why in Canada we used circles first when in our curriculum we don’t start really working with properties of circles until Grade 6 and on. I know a number line is a specific fraction representation of a linear model or fraction as a measure from 0, but it can be related to the area models. What do you think of this information Marilyn? I still see circles used often, if not as the go to model in many classrooms. Do you have any thoughts?
Thanks for your reply and I look forward to digging in to Dr. Cathy Bruce’s research. I appreciate the link. I’ve thought lots about models that promote understanding of fractions, though I haven’t conducted any formal research. My preference has been, for quite a while, to have students create their own Fraction Kits using paper strips, which is a measurement model. (Even though the strips are rectangles, we focus on comparing their lengths, not their areas.) And I begin with related fractions that have denominators that are multiples of each other — halves, fourths, eighths, and sixteenths. Then they extend their kits to include thirds, sixths, and twelfths. I do introduce circles, but later, and typically in the context of division, where different numbers of children are sharing different numbers of cookies. And I use the strips to introduce thinking about fractions on a number line. (I posted a blog about a recent experience with this approach: Fractions on a Number Line.) I introduce fifths, tenths, etc. when students can extend their understanding without relying on the strips. Yesterday, I talked to the class I wrote about in the blog about figuring out if 4/5 or 5/4 was closer to 1. None of them used circles. They figured out how far each was from 1 to decide that 4/5 was closer because 1/5 is less than 1/4. All this said, I’m most convinced that there is no one best sequence that meets all students’ needs, but I’m feeling successful with the approach I’ve been taking and its success with the students I’ve been teaching. I hope this is helpful. Please feel free to question me further.
I agree that it’s hard for students to draw fraction circles, but I’ve found it can be successful to have circles (paper plates etc) and have students fold them to find some easy fractions – 1/2 , 1/4, 1/8.
Generally I agree that circles are an overused model, and number lines are underused – I also like number lines for counting – easy to see how you go beyond 1 whole by just keeping adding more quarters or halves etc to the line.
Marilyn, I’d be interested in your take on some of Cathy Bruce’s work – one part I struggle with is her focus on using fractional notation to express part: part relationships.
For example if you have 2 cans of juice concentrate and 6 cans of water, this relationship can be expressed with the fraction 2/6.
To me, I’d always write that as a ratio. Ratios are flexible ways to express relationships – part: whole, part: part etc. and when people use a ratio they state what the ratio is representing “The ratio of oil to vinegar in the salad dressing is. . .”) On the other hand, fractions are not communicated this way, and so are assumed to be used for part/ whole relationships.
It would seem to add confusion to a topic that students already struggle with and I’m not sure what it adds as a mathematical representation.
I look forward to reading about Cathy Bruce’s work — thanks for the suggestion. The relationship between fractions and ratios is important, but can be difficult. I found it useful to refer to the Progressions written for the Common Core Progressions. The Common Core position is to use ratios both in stituations where units are the same (as in the example you gave above for 2 cans of concentrate and 6 cans of water) and also in situations where units are different (e.g., 3 meters and 2 seconds). The Progressions document states (and I substituted the example you gave above for the ones in the Progressions): A ratio associates two or more quantities. Ratios can be indicated in words as “2 to 6” and “2 for every 6” and “2 out of every 8” and “2 parts to 6 parts.” This use might include units, e.g., “2 cans of juice concentrate for every 6 cans of water.” Notation for ratios can include the use of a colon, as 2:6. The quotient 2/6 is sometimes called the value of the ratio 2:6. Ratios have associated rates. For example, the ratio of 2 cans of juice concentrate for every 6 cans of water has the associated rate 2/6 (or 1/3) cans of juice concentrate for every 1 can of water. Students in grade 6 are expected to use ratio and rate reasoning to solve problems. Prior to grade 6, students study fractions as parts of whole (where the whole could also be a set). I’m not sure this helps, but it was good for me to refer to the Progressions document and think more about this. Thanks for your comment.
Thank you for response. Makes total sense what you are saying. I myself have seen the most gain when students use number lines but also feel it needs to be what makes sense to the students as you stated. Giving them more choice in what works for them. I like the idea of simplifying how many models we use in North America and working on making sense and reasonableness instead of procedures. In the junior grades I work with in my schools (4-6) we have been having amazing gains using number talks out of Ruth Parker and Cathy H book making number talks matter. And it’s doing exactly what you were doing in the second lesson. Putting fractions up and asking if they are closer to 0, 1/2 or 1 and have them explain there reasoning and agree or disagree with each other. These seem to really increase the students conceptual understanding fast especially when anotated with a visual model that shows there thinking. Thanks for sharing Marilyn I really enjoy your blog posts.
For more ideas for number talks with fractions, see a free sampler of Number Talks: Fractions, Decimals, and Percentages by Sherry Parrish (the author of the K-5 Number Talks book) and Ann Dominick. And thanks for the feedback on my posts. It encourages me to finish the others I began during the year and just never completed. Maybe a summertime project when there seems to be more time to reflect.
To tell or not to tell…Thank you for posting this question. I often get it after I have teachers and/or students struggle with a problem during a session. I appreciate how you showed that more experiences and reflection can reveal even more about what a learning understands or doesn’t understand. What did you and Sara decide to do after the follow-up question? What were the next steps?
Thanks for your comment. See my response to Joe Schwartz’s comment for what I’m planning to do next and my uncertainties about doing so. Stay tuned for a follow-up report.
To expand on what Johua said, I think some of the problems students have with this topic is that the whole is an arbitrary figure. Recently I was trying to support students in a grade 4 class, a co- teaching group had challenged them to represent 1/2, 2/2, 3/2 and 4/2. This was a great question. As one student kept telling me that 3/4 and 4/2 don’t make sense, I tried going back to the familiar example of pizzas. I asked her to show me 1/2 of a pizza. She drew the standard cycle and shaded in 1/2. Then I drew 2 of the same size circles, and asked her how many 1/2 pizzas there were. She told me “4 halves” so I said that was correct, and that we could write 4 halves like this 4/2. This seemed to be an “ah-hah” moment.
I always try to make sure that teachers aren’t just using circles for fractions, many models are needed, but, as you commented, with a circle you can always tell what the whole is. Sometimes it makes a good starting point.
Thanks, Julia, for adding on to Joshua’s thoughtful comment. Yes, multiple models for sure, and understanding the importance of defining the whole. See my response to Mark Stamp’s comment about when I bring circles into students’ learning about fractions.
I really like how this teacher gave the students a problem and ask the students to show how they represent 5/4. Some students were able to understand and write what 5/4 is and some could not do it. By not telling the students the answer and asking each and everyone of them to find a representation of what it means will allow the students to understand the thinking process. It seems to be very important to understand what a whole is before students go into working with fractions. By having a deep understanding of what each whole means will allow the children to understand the fractions and why it works that way.
Thanks for the link to the Progressions – they look really interesting.
I work with adults and find that it’s really important to use different representations and to make sure they’re understanding the language for the ideas, too. Some of them have years of practice figuring out strategies to get answers if they’re from one model that have precious little to do with the math. The meaning of “whole” is so tricky!