When I visited Joe’s third-grade class, he was concerned about the results on his students’ homework from the day before. It was a computation 
practice assignment, and practically all of the children had either gotten the wrong answer to 503 – 398 or indicated that it was “too hard.” (Writing “too hard” was an option they had to let Joe know when they couldn’t figure out how to do a problem. This gave Joe useful feedback about what he needed to work on, either with the entire class or with specific students.) Joe asked if I could give some help.
The problems on the homework assignment were all written vertically, so that students could apply the standard algorithms they were learning. But to trigger their thinking without focusing on the procedure they had learned (which wasn’t serving most of them here), I brought them to the rug, made sure each student had a partner, and wrote the problem horizontally on the board:
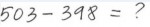
Making an Estimate First
“The numbers in this problem aren’t particularly friendly,” I said. “Let’s think about changing them to friendlier numbers and estimating the answer.” Making estimates is something I do regularly with students to give a ballpark solution against which they later can compare their answers. The students agreed that an easier problem to solve would be 500 – 400, which they knew was 100. I recorded this underneath.
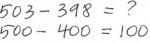
“So we know that the answer to 503 minus 398 is close to 100,” I said. “But how close? And do you think the answer is greater than 100 or less than 100?”
I asked them to turn and talk. Conversations broke out and the room got noisy. When I called them back to attention to share their ideas, there was a difference of opinion. Some thought that the exact answer would be greater than 100 and others thought it would be less than 100. Some thought it would be 5 more or less (adding the 3 and the 2 “left over” after rounding), and others thought it would be 1 more or less (the difference between the leftover 3 and 2).
About Open Number Lines
To help them resolve the issue, I introduced them to the idea of an open number line. I’ve found that using an open number line to subtract is enormously helpful and has several benefits:
- An open number line reinforces the idea that the answer to a subtraction problem is the difference between two numbers.
- It supports using the inverse relationship between addition and subtraction by counting up instead of back.
- It engages students in decomposing numbers and reasoning.
- It provides a visual model that’s a useful tool.
These are good reasons to use an open number line.
What I don’t like about the open number line is its name. First of all, I rarely draw a line. And when I locate numbers, I don’t make their locations proportionally correct, as they should be on number lines. In a way, this makes the math part of my brain screech. (That said, I do pay some attention to spacing—for example, I wrote 400 and 500 far apart with 398 next to 400 and 503 next to 500.)
But, despite the imperfect name, I do like how open number lines empower students.
How I Introduced Using an Open Number Line for 503 – 398
First I wrote 400 and 500 on the board:
Then I drew a “jump,” explaining that jumping from 400 to 500 shows the difference between the numbers and that 500 – 400 equals 100:
Next I wrote the numbers from the original problem:
I recorded small jumps to show those differences:
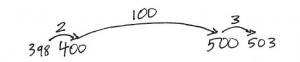
I then said to the class, “Turn and talk again and see if what I drew convinces you about what you thought or helps you to change your mind.”
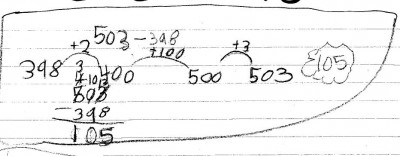
Conversations broke out again. When I called the students back to attention to share, they still had some differences of opinion, but more were convinced that the answer to 503 – 398 had to be 105. Fran explained it clearly: “There’s an extra 2 from 398 to 400 and an extra 3 from 500 to 503, so it’s 105 from 398 to 503.”
I then had them return to their desks. I showed other examples using simpler numbers and modeling various ways to make jumps, and I gave them practice with trying some on their own.
Students Solve 503 – 398 Again Using Two Different Ways
After a few days of practicing with other examples, I gave the students the assignment of solving 503 – 398 again. I asked them to figure out the answer in two different ways. What I loved about their work was the variety in their thinking. See the four examples below.
Camille used the open number line and then the standard algorithm.
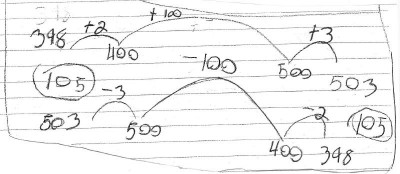
Laura used the open number line for both solutions but in two different ways. In her second solution, she reversed the order of 503 and 398 and subtracted instead of adding.
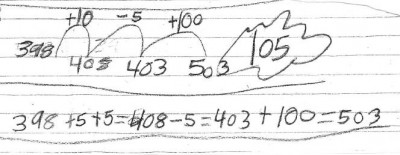
Fran used the open number line, but not as I had demonstrated. She made a first jump to add 10, then subtracted 5 (trying to get back to 403 for an easy last jump to 503), and then made a final jump of 100. After this, she represented her thinking in another way. Both methods are unconventional, but both show reasoning and number sense.
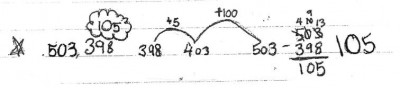
Zoe made only two jumps on her open number line, first a jump of 5 to get to 403 and then a jump of 100 to get to 503.
Classroom Suggestions
Try this lesson as I described it, adjusting the numbers to be appropriate for your students. For younger students, start with subtracting multiples of 10, like 60 – 20. (I find that while some students are comfortable making one leap of 40, others makes leaps of 10 or 20. All of these are fine methods.) Or use a two-digit problem like 82 – 57, encouraging students to use multiples of 10 as benchmarks. For older students, try a problem with greater numbers, like 5013 – 1998.
P.S. I’m obsessed about subtraction. See two more posts I wrote: Another Way to Subtract and Yet Another Way to Subtract.
I love how this post shows the benefits of the open number line without making it seem like another procedure to teach. I am a long time fan of your writing and math ideas and am very excited to see you have a blog!
Marilyn,
Good piece. I liked the overall lesson and the specific number line lead in to the estimation.
I just did a talk this past Sunday for the Unitarians on the Common Core Controversy here in SaddleBrooke, AZ. They were quite interested and, finally, saw that the controversy is not all over the mathematics, but all of the add ons in assessment, the rewriting of the NCLB legislation, and the lack of quality professional development for the teachers–as well as quality instructional materials.
So it goes, hopping along in 20 year increments 1970s, 1990s, 2010s,… what will come next?
Keep up the good work,
John and Anne Dossey
I love the use of a number line! The challenge is that parents probably are not on board. Sometimes
all our great teaching becomes confusing when parents interject their teaching thoughts.
I am such a fan of the number line especially for subtraction. I actually encourage it more now as I see less mistakes
I loved your explanation about how the open number line doesn’t fit the name. I’ve found that when I’m using the strategy with coaches and teachers. I also found the question about whether the answer was less than or more than 100 very thought-provoking.
I modeled using the number line with my parents at an Open House to help them understand that it is a visual way of building the concepts of add/subtraction. Once they saw it and practiced it with me, they were more on board. I have had to purposely show them math strategies the last few years and earn their support, but it can happen! We use the number line with elapsed time too. Great tool that the children can draw on their tests to prove their answers.
I teach high school math, and my students have no number sense (‘m not exaggerating). The only strategy they really know is counting up/down by ones. They don’t ‘know’ the number bonds for 5-10, 10 more or 10 less, and 20 is as friendly a number as 23.67! I’ve discovered they see numbers as the actual symbol and not as a quantity. Math facts for them are as arbitrary as if I gave you a list of letter equations like a x b = c and expected you to memorize them. Again, they are in high school so my efforts to remedy this is a hard sell. If we are adding 27 + 6, they don’t know the next multiple of 10 is 30 (not friendly), so it wouldn’t be helpful to add 3 from the 6 to get there (they would have to count that by ones) and then add the other 3 (counting by ones) to get 33 since they don’t readily know 6 = 3+3. They feel it is so much easier to just count up by one 6 times than all the foolishness I am trying to get them to do. They are very emotionally attached to counting up. If I up the ante and add 27 + 36, they will just line them up using the traditional algorithm. I am trying to use open number lines with this telling them they can ultimately manipulate these numbers in their head, but they will often do it off to the side the traditional way (even though I have asked them not to) and then fill in the number line. FYI, every day I do some smaller number sense routine where we investigate number bonds for 5-10, count up/down by 10, find the next multiple of 10, but I still find them counting by ones and they are frustrated with me for having them do this ‘second grade’ math even though they don’t know it and it holds them back from tackling bigger grade level problems. What am I doing wrong? How can I get them to see the value in this?
Your situation calls for the utmost in patience to find ways to address where the students are and encourage them to move forward. Last spring I tutored a sixth grader who could use algorithms to compute, but often wasn’t able to decide which algorithm to use when and mostly wasn’t sure what to do with the results. He did not reason mentally at all. The algorithm was always his go-to method and I didn’t discourage him from using them since that’s what he trusted. I’d coax him to try something mentally and then check with the method he knew. He didn’t often trust his own reasoning, just the algorithms. I spent my time using the open number line and giving him lots of practice with games. He made progress but, my, it was in baby steps. Your job is even tougher with older students are more of them. I wish I had a magic wand. I don’t. But I sense your caring and I encourage you to keep at it. Anyone else have a suggestion? Please post!
In my many years of teaching the use of the open number line, I often observe students subtracting by moving from left to right on the number line. They subtract in the same direction they would write or read an equation. In the past, I was unconcerned about this model–especially if students could explain their thinking. Now I wonder if, at least for some students, we’re glossing over potential issues with magnitude of numbers when students model 400, for example, to the right of 500 on a number line model. I notice in one of Marilyn’s examples of student work, one who modeled subtraction taking away while moving to the right on the number line. I would much appreciate others’ thoughts on this subject. After many years teaching and coaching math teachers, I’m conflicted.
I’m interested as well in others’ thoughts. It doesn’t bother me and the convention of greater numbers to the right seems to resolve itself as students mature. Still, the “open number line” is more of a tool than a number line representation as we ususally use it.