My grandson Jeffrey is a terrific kid―generous, cheerful, trustworthy, and kind. He does well in school, loves to read, and likes helping in the kitchen. (OK, enough bragging.)
Jeffrey’s true love is sports, especially baseball. For his 11th birthday, we bought him subscriptions to two baseball magazines and included information about them with his birthday card. It was going to be a while before the first issues of the magazines arrived, and we wanted to give him something he could enjoy at the party.
So I decided to make a book for him about the number 11. I researched facts and images online and created a Word document. I selected eleven entries, printed them, cut each page in half, and assembled and stapled them into the 5 ½ -by-8 ½-inch booklet in the photo above.
When I finished, I thought about how making a book like this would be a good classroom activity. I sent the Tweet below and included a photo of the book cover. There were several responses to the Tweet, asking for a peek inside and suggesting ways that I might share the pages. I couldn’t figure out how to share the book on Twitter, so I decided to write this blog.
When I started making the book, I thought that I was making a math book, but it turned out to be more. At his birthday party, Jeffrey opened the book and read it aloud to all of us.

There were four extra entries that I hadn’t included in the book. They were mathematical, and I couldn’t bear to exclude them. So I printed them on one full page, rolled it like a scroll, and gave it to him separately. He read those aloud, too.
Below are the eleven entries in the book, and the extra math page. If you try this with your students, for any number, I’m interested in learning about how you structured the activity and what resulted.
A Final Note
An inspiration for the book I made is the children’s book 12 Ways to Get to 11 by Eve Merriam. It’s a book that’s perfect for first graders. (The lesson is described in Math and Literature, Grades K–1, a book I wrote with Stephanie Sheffield.)






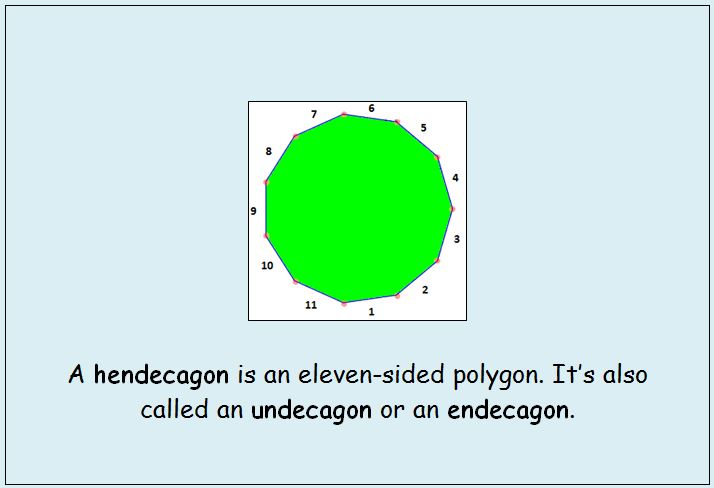


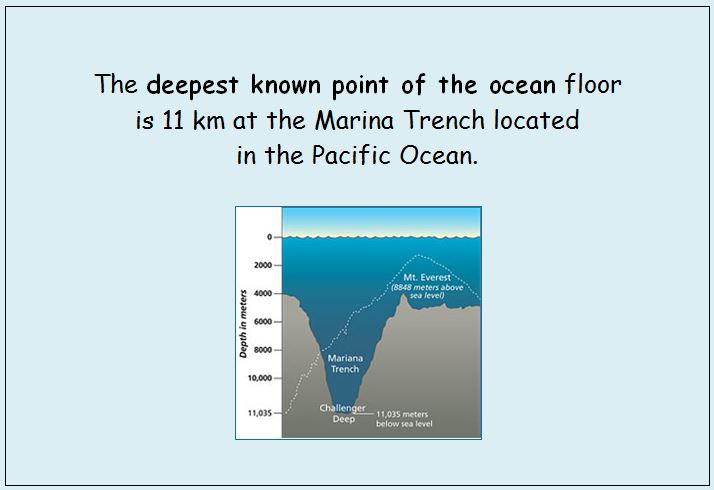

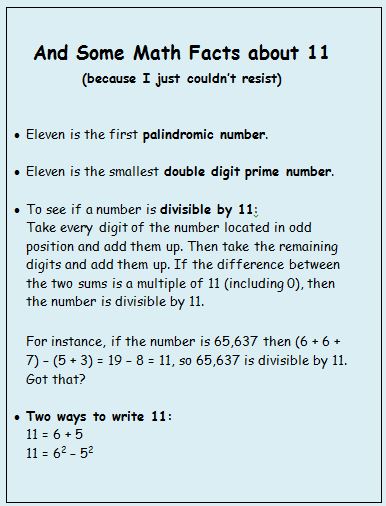
And the extra page of math ideas that I also included:


Marilyn, what a great and creative idea about numbers! I will now have to get started working on 12 book for my niece’s birthday! Thank you for sharing.
One of my favorite books to use in teaching mathematics is 12 Ways to Get to 11 . I love this math project idea!!! Your work continues to inspire me!
I love your book. I am particularly impressed with the wide variety of examples that you found. When I read your intro I thought you might include several sports connections as it seems to be a great interest of your grandson. I can picture students being very engaged in the research aspect of a project like this and the cross-discipline aspect is very appealing.
FYI- Field hockey also has 11 players. 🙂
Marilyn, you have inspired me and all of the students that I had in class while I was teaching elementary school for 30+ years. Now your 4th edition book and your blogs are inspiring my pre-service teachers. Like you, education is my passion and you have been my mentor for decades. Thank you.
Another inspirational book along this line is Numbers – Facts, Figures, and Fiction by Richard Phillips. The book includes detailed information for all numbers 1-156 as well as brief information for numbers 157-1000 and notable large numbers. His website by the same name – http://www.richardphillips.org.uk/number/ – includes wonderful information for numbers 1-31.
I immediately notice how many skills students can develop due to the nature of the task. This is creative and a great way to motivate children to want to learn. Students of varying ages would love to learn fascinating facts about any number (whether age, favorite number, etc). This could help them build some research skills from an early age. Also, by initiating the process of investigation, they will be invested in understanding concepts (like you promoted with terms like 11 being a dihedral prime), because they’ll want to understand what that term means. It is provides them with opportunities to develop technology skills from researching online to choosing a technological medium through which they can present their findings, as well as presentation skills if shared with the class afterwards. Thanks for sharing this fun, engaging, creative idea!
I love this idea so much…think I’ll go through family photos of the past year and see how many I can find that fit the bill, and then add some fun facts too. What a wonderful idea! Think 6…the number of baseball players in the infield…the possibilities are limitless!
I’m a former Math teacher and current blogger about my amazing son @ amazinglucas.com.
Your book is brilliantly done! What a great way to expose numbers to younger kids. Your book would even motivate the reluctant kids!
Great job!
Canan Harvell @ amazinglucas.com
11:11 is also the only time that a digital clock has the same digit in all four spots! I always make a wish when it is 11:11 ever since I was a kid. Now that I am an adult, google has informed me that 11:11 is special to more than just me. 🙂
Marilyn,
You are a peach, and I never stop learning from your generous offerings!
Thank you for who you are, what you do
and…
especially for…
being YOU!!!!
PS hope I have not misspelleed,
not wearing my gllassses!
I like the scroll finale! That is,
11 = 6+5 = 6^2 – 5^2.
Is this a coincidence?
Well, if we increase each addend, then we have 7 and 6:
13 = 7+6.
What about 7^2 – 6^2? That’s 49 – 36 = 13, as hoped!
And then there’s 8 and 7…
Yikes! I hadn’t thought about it, but it works for all pairs of numbers that are 1 apart. I figured it algebraically first and looked at it geometrically. Thanks so much for noticing this. You’ve inspired me to write a short blog about this. Stay tuned.
Great; I look forward to the post!
Meanwhile, I don’t know how you (personally) “look at it geometrically” — my guess is by taking a 6×6 array and removing a 5×5 array? [Though I’m sure there are other ways…]
Well, if that is the case, then you could consider continuing the pattern as follows:
What is 6^2 – 5^2 + 4^2 – 3^2 + 2^2 – 1^2?
That’s a lot of numbers to start with (for me, at least!).
Beginning with a smaller even number, 4, we find:
4^2 – 3^2 + 2^2 – 1^2 = 4 + 3 + 2 + 1.
Here’s a picture indicating as much: http://i.imgur.com/xnFzqh8.jpg
(The thinking is: take a 4×4 array, remove a 3×3 array; now put back a 2×2 array; now remove a 1×1 array; now color the blocks in to improve clarity!)
Other problems can be explored similarly (without invoking algebraic identities, though they can be used, if desirable, to connect representations between the pictorial and the symbolic).
One example is an observation like: from 3, 4, 5, let’s square the middle number and take the product of the other two; this leads us to 4*4=16 and 3*5=15. And 16=15+1.
So: 3*5 + 1 = 4*4.
Here is an image transforming 3*5 + 1 into 4*4: http://i.imgur.com/k9n68im.jpg
What about for other sequences of three consecutive counting numbers?
Say, 7, 8, 9 (punchline to the classic math joke).
Is 7*9 + 1 = 8*8? Yes, it looks to be so; but maybe this is another coincidence…
Anyway: Block-play can get into some deep explorations!
MQ
http://mathwater.wordpress.com
This is wonderful!!! It has so many possibilities for a wide range of learners. Many thanks for sharing this with us.
Thanks for the idea, Marilyn. I just finished a book, Now You Are Two, for my granddaughter, Layla.
I’ve begun thinking about 6 for next March!