Squeezing in time to work with Oscar after school wasn’t easy for him―baseball practice and games consumed many of his after-school afternoons. But his mom finally found a time to bring him to my house. It was the first time I had met Oscar.
I asked Oscar if I could ask him some math questions. I explained, “I’m interested in how you think about math. I’d like to give you some problems to solve in your head and listen to you talk about how you’re thinking. Then the two of us can learn about how we might work together.”
Oscar looked skeptical but agreed to answering questions. Well, honestly, what else could he do with his mom sitting nearby observing?
I asked, “So, which would you prefer we talk about—whole numbers, fractions, or decimals?” Giving Oscar the choice was a way for me to get a sense of his level of confidence. Also, it was a way to give Oscar the message that we were working together, that I was giving him some choice over what we’d do. Without hesitation, Oscar opted for whole numbers.
“OK,” I said, “and would you prefer to think about adding and subtracting, or about multiplying and dividing?” Again, without hesitation, Oscar answered, “Adding and subtracting.”
The Interview
I asked Oscar four questions from the MRI whole number interview which focuses on adding and subtracting numbers within 1000. I kept careful notes about how Oscar responded. The first question I asked was, “What is 99 plus 14?” I showed Oscar a card with the problem written horizontally.

Oscar thought for a long moment and then gave the correct answer of 113.
“How did you figure out the answer?” I asked.
Oscar explained, “I know 9 plus 4 is 13. So, I carried the 1, and then I added the tens.”
“Can you tell me more about how you added the tens?” I prompted. It seemed that Oscar was figuring out the answer in his head as if he were solving it with paper and pencil, but I wanted to be sure.
Oscar thought again for a moment, and now he visualized the problem, scribing with his finger in the air as if he were actually solving a written problem. “I did 9 plus 4, wrote down a 3, and then put the 1 on top of the 9. Then 90 and 10 is 100 and the extra 1 that I carried makes 11. So, the answer is 113.” “Got it,” I said.
In his explanation, Oscar used a mix of mathematical language, which I find typical. He knew that the digits in the tens place were worth 90 and 10, an indication that he understood the place value structure of two-digit numbers. But then he referred to the 1 that he carried as 1, not as 10. I didn’t learn if Oscar could solve the problem in another way, but I did see that he was able to apply the paper-and-pencil algorithm that he had learned.
The second question I asked Oscar was, “What is 180 minus 90?” Again, I showed the problem written horizontally.

Oscar gave the correct answer of 90. When I asked him how he figured out the answer, Oscar explained, “I’d do 180 minus 80, and that gets me to 100, so I have to take away 10 more, and that gets me to 90.” Here Oscar didn’t resort to an algorithm, but instead demonstrated his ability to break 90 into 80 and 10 in order to get to the friendly partial answer of 100. I took this as an indication that Oscar had the ability to make use of the important benchmark number 100.
“The next question is a missing number problem,” I said. I asked the question and showed it to him on a card, “Twenty-five plus what number equals 200?”

When I showed Oscar the problem on the card, he frowned, initially not sure what to do. Then he brightened and said, “Oh, I have to subtract. Can I use paper and pencil?”
“Can you try and solve in in your head?” I coaxed gently.
Oscar then proceeded to do what he had done when adding 99 + 14, scribing in the air. He visualized lining the numbers up and, with his finger, did an elaborate amount of crossing out and replacing digits in the 200, then subtracted the 5 in 25 and then the 20.” He reported the correct answer, “A hundred seventy-five?” The upturn at the end indicated to me that he wasn’t completely certain. I didn’t probe. I typically ask students to explain how they figure out answers, but here Oscar’s method was obvious. I merely said, “Got it,” and continued.
I showed Oscar another problem and asked, “How much is 1000 minus 998?”

Oscar gave an audible sigh. “What are you thinking?” I asked him.
“That’s a lot to do in my head,” he answered.
“I agree,” I said. “Is there another way you can think about the numbers so you don’t have to do all that crossing out and keeping track?”
“Well, maybe,” Oscar said. He sat quietly, thinking.
“Can you talk about what’s going on in your head?” I asked.
“I know there’s a 2 in the answer,” he said.
“How do you know that?” I said.
“Because 8 plus 2 is 10,” he said. He thought quietly a bit more, and then gave an answer, again with an upturn, “Maybe 112?” (The MRI data of responses from almost 35,000 students reveals that 112 is a common incorrect answer to this question.)
“Can you explain how you got 112?” I asked.
“I’m really not sure,” Oscar said. “I know it ends in a 2, but you also have to take away the two 9s, so I think that’s why there are two 1s, so I think 112.”
“Final answer?” I said. I often ask this to see if a student wants to think more about a problem. Oscar was OK with 112.
What I Learned about Oscar
When I interview students, I listen to learn about what they understand. Rather that focus on misconceptions and gaps, I think it’s extremely important to think about what students do understand and what they can do. Then I can make instructional decisions that acknowledge, incorporate, and build on their strengths instead of focusing on finding and fixing deficits.
Thinking about Oscar, I learned that he understands the place value structure of our number system. When adding 99 + 14, he seemed comfortable knowing 9 plus 4 was 13, similarly with 8 plus 2 when he was thinking about how to subtract 1000 minus 998. To solve the missing number problem, 25 + ___ = 200, he knew that he could figure out the answer  by subtracting, which showed that he applied the inverse relationship between addition and subtraction. With numbers that were accessible to him, as with the problem of 180 – 90, Oscar demonstrated his ability to decompose 90 into 80 + 10 and then use that information to get to the benchmark number of 100. Using paper and pencil was his preferred way to solve problems and he seemed neither comfortable nor experienced with reasoning in his head.
by subtracting, which showed that he applied the inverse relationship between addition and subtraction. With numbers that were accessible to him, as with the problem of 180 – 90, Oscar demonstrated his ability to decompose 90 into 80 + 10 and then use that information to get to the benchmark number of 100. Using paper and pencil was his preferred way to solve problems and he seemed neither comfortable nor experienced with reasoning in his head.
I next asked Oscar if he would solve the same four problems with paper and pencil. I typically don’t do this during interviews in classroom situations―it’s easy enough to give students a paper-and-pencil assessment and find out about their ability to use written procedures. But I was just learning about Oscar and wanted him to have the opportunity to show what he could do. Oscar was willing to solve the problems on paper, even eager, which was the first glimmer of confidence that Oscar displayed. He solved all four problems easily, quickly, and correctly, showing his comfort with paper-and-pencil procedures.
I then talked with Oscar and his mom, who had been observing carefully. I told them both about what I was pleased to learn about Oscar’s math understanding, explaining what I wrote in the paragraph above. I wasn’t sure that either Oscar or his mother knew what I meant by “place value structure,” “inverse relationship between addition and subtraction,” or “benchmark number of 100.” But they visibly relaxed as I described what I learned about what Oscar could do. I didn’t include the last part of the last sentence above, that Oscar was “neither comfortable nor experienced with reasoning in his head.” Instead, I turned to Oscar and told him, “It’s clear to me that you’re comfortable with paper-and-pencil math, and good at it, which indicates to me that you’ve practiced a lot.” For the first time, Oscar grinned and sat back in his chair.
“What’s also clear to me,” I said, “is that doing math in your head isn’t as easy for you as doing math with paper and pencil.” Oscar nodded and again grinned.
“So maybe that’s something we can work on together,” I said.
This was almost enough for our first session and I told Oscar that I was willing to see him again, if he was interested, and that I wanted to talk with him more about multiplication and division, also about fractions and decimals.
“Decimals are hard,” Oscar volunteered.
“Yes,” I agreed, “but you’re a baseball player, yes?” Now he really grinned.
“So, you know about batting averages?” I asked. He nodded.
“Well, they’re decimals,” I said.
I chatted a bit more with Oscar about baseball because I wanted him to feel that I was interested in him and in what he cared about. Also, I was trying to make Oscar feel comfortable enough to be willing to come for more sessions. I felt I had as much to learn about helping Oscar as he had to learn about reasoning numerically.
Revisiting an Earlier Problem
I worked with Oscar for about a dozen sessions. In one of our tutoring sessions, I returned to the missing number problem I gave Oscar when we first met: 25 + ___ = 200. By then we had spent time thinking about 25 as a benchmark number and how using money could be helpful. We also worked with open number lines as a tool for figuring out differences. For this missing number problem, Oscar was now able to add up in his head to figure out that the answer was 175. He explained, “I know that you need 75 more cents to get to a dollar, and then another 100 cents to get to 200 cents, so the answer is 175.” And while this seemed like a triumph to me, Oscar was still more comfortable with paper and pencil and asked if he could check. He subtracted correctly.
I then gave Oscar another problem, one he hadn’t seen before: 112 + ___ = 1000. “Can I use paper and pencil?” he asked? I agreed and here’s what Oscar wrote in the notebook we used during our sessions:
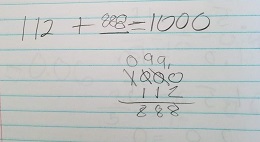
Underneath his work, I wrote the related subtraction problem:
1000 – 888 = ___
“Do you know the answer to this?” I asked him.
Oscar looked at me, surprised, and responded quickly, “It’s 112.” He pointed to the problem he had just solved in the notebook. I then completed the equation, and Oscar nodded in agreement.
1000 – 888 = 112
I then thumbed back in the notebook and showed Oscar a question I had asked him the first day we met: 1000 – 998. “I asked you to solve it in your head,” I said, “and here’s the answer you got.” I showed Oscar where I had recorded 112 next to the problem. Oscar nodded, seemingly recalling.
Then I returned to what I had just written and recorded this equation underneath.
1000 – 888 = 112
1000 – 998 = 112
“Something here doesn’t make sense to me as being possible,” I said. “Can you see what that is?” We had spent lots and lots of time in our sessions talking about the importance of things making sense and being able to explain why they did. Here I was asking Oscar to explain why something didn’t make sense.
“They can’t both be right,” he said. “You can’t subtract two different numbers from 1000 and get the same answer.”
I sat as Oscar quietly looked at the problems. Then he hit his head lightly with his hand and said, “If I just add up I know that 998 plus 2 is 1000. Duh. So, I was wrong back then.”
This exchange seemed to validate for Oscar that he was making progress. It was validation for me, too. It’s not that I felt that I had accomplished enough with Oscar, but I knew that I had shifted how he thought about math away from only following procedures to also reasoning in other ways and evaluating when answers made sense. I asked him to solve a few more problems mentally, which he was able to do by adding up each time:
1000 – 997 = ___
1000 – 993 = ___
1000 – 989 = ___
We had more to do, but we both left this session feeling a sense of progress.
Using Written Procedures as a Springboard to Reasoning Mentally
There are times when it’s not reasonable to solve a problem mentally. For example, in one session I asked Oscar to figure out the answer to 193 x 47. Of course, I allowed him to use paper and pencil. It would be ridiculous and inappropriate to expect him (or anyone?) to compute the answer mentally.
I asked Oscar to do this calculation for several  reasons. One was that I knew it was something he could do and that it would contribute to his feeling confident and accomplished. Another was that I would then be able to use the problem to engage Oscar in reasoning about multiplying decimals, something he was studying in school.
reasons. One was that I knew it was something he could do and that it would contribute to his feeling confident and accomplished. Another was that I would then be able to use the problem to engage Oscar in reasoning about multiplying decimals, something he was studying in school.
Here’s what Oscar wrote:
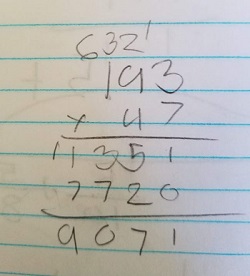
“So, what’s the answer?” I asked Oscar.
He answered, “Nine thousand and seventy-one.”
“Let’s check it on a calculator,” I said. Oscar did the problem on my calculator and verified the answer.
“Here’s another problem,” I said and wrote in his notebook: 19.3 x 47. When Oscar reached for his pencil, I put my hand gently on his and said, “I wonder if you could use the answer you figured out correctly for 193 times 47 to figure out the answer to this problem.”
Oscar was lost.
“What do you notice about the numbers in the two problems?” I prompted.
Oscar responded, “They’re the same numbers, sort of, but this one has a decimal point and this one doesn’t.” He pointed first to 19.3 and then to 193.
“Which of those two numbers is greater?” I asked. Oscar pointed to 193.
“How many times greater?” I asked.
“What do you mean?” Oscar asked. I wrote in his notebook:
200 20
201 20.1
I said, “I would say that 200 is 10 times greater than 20.” Oscar nodded his agreement and I continued, “and 201 is ten times greater than 20.1.”
Oscar didn’t nod readily but sat thinking. Then he asked, “Oh, so 193 is 10 times 19.3?”
“Do you think that’s right?” I asked.
“Yeah, well, I’m not really sure,” he answered.
From this questioning, I learned about Oscar’s rocky understanding and insecurity. Because I want to use his strengths, I asked Oscar to multiply, with paper and pencil, to check if 19.3 x 10 was equal to 193. He did this handily, and we also verified it with a calculator. But Oscar wasn’t able to use the information that 9071, the product of 193 x 47, was ten times greater than the product would be for 19.3 x 47. He didn’t deduce that the answer to 19.3 x 47 was 907.1. At least not yet. I still had my work cut out for me for helping Oscar build on his strengths to think about problems that were new and puzzling for him.
In a classroom setting that’s focused on building students’ ability to think and reason with numbers, problems like these are excellent choices for number talks, where students can explain their reasoning and hear explanations from others. Tutoring has the advantage of focusing on just one student and learning, in depth, how that student thinks, but doesn’t provide the student the opportunity to learn from his peers. In our sessions together, I circled around whole numbers, fractions, and decimals. I taught Oscar games to take home and play with his parents and his sister. I never devalued Oscar’s ability to do paper-and-pencil math and allowed him to solve or verify with paper and pencil when he wanted to, but I also regularly encouraged him to think mentally, make estimates, and try out different strategies.

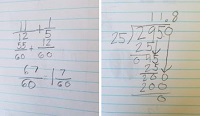
193 x 47 is easy to do mentally. 9071
Of course, I have to see the 193 and 47 written down, so it doesn’t really count as ‘mental’ arithmetic. You do it by a modified version of the Chinese (or Gelusia) method.
When I was young, I could do 4 digits times 4 digits, but that is now a huge effort for me.
And to do 19.3 x 47 I do whole numbers, and add in the 1 decimal place I ignored. I can’t multiply decimals.
The main thing when doing 19.3 x 47 is to recognise it is about 20 x 50 or 1000. If you want the exact number, use a calculator.
Agreed, both about estimating and then using a calculator, and also about things that used to be easy. I love this quote from Gail Godwin’s novel Grief Cottage: “These days, Marcus, I have to put in requests to my brain, as one does at the library, and then a little worker takes my slip and disappears into the stacks. It may take him a while, but he always comes back with the goods.”
Thanks so much for sharing your knowledge. I always enjoy digging into students knowledge and building from there.
I wondered if you could have asked Oscar to estimate how much 200 x 50 would be as a proxy for solving 193 x 47. Similarly, in the subsequent calculation with 19.3 x 47, you could have asked him to multiply 20 x 50. This might have helped him see that the result is (will be) approximately 10 times as large. Also, it leverages Oscar’s strength of multiplying to get him to possibly see the connection between the numbers. Just a thought.
Anyway I loved the story and your writing. I agree wholeheartedly with your idea of focusing on what the students do understand and can do rather than what needs to be remediated. And as a teacher who is involved in numerous IEP meetings, I also believe that some of these meetings focus too much on what the students cannot do or what deficits they have INSTEAD of what skills the students possess and what strengths they bring.
That would have been a good idea. Thanks for the suggestion. Next time, I hope.
I wonder if teachers will ever finally believe that teaching kids to think and reason about numbers is far more powerful than teaching them algorithms as their main go-to method of computing?
Hear, hear!
Yes please Lucy!
I loved how you showed specific examples of building off what Oscar knew about solving problems with algorithms to work with him on solving problems mentally. Thanks for sharing.
I was Oscar.
I’m convinced that all of us who had “Oscar” experiences are better teachers for it.
I appreciate that you built on Oscar’s existing strengths to help him to reason mathematically. The example of Oscar makes me think of how to utilize my students’ procedural knowledge to further their mathematical reasoning. As a fourth grade math teacher in a disadvantaged school, I find that most of my students have procedural knowledge of the addition algorithm. Most of them also have misconceptions about the subtraction algorithm. However, even when the procedural understanding is flawed, it can still be a good starting point for building on mathematical reasoning.