Four Strikes and You’re Out

Several issues come up regularly when teachers seeking help with their math instruction ask me questions. One is a general question: How can I motivate my students to enjoy math more? Another relates more to managing instruction in the classroom: What can I do during math lessons when some students finish their work quickly while others need more time? Another is a concern about practice: How can I give students the practice they need without relying on more worksheets?

One suggestion I offer to respond to all of these questions is: Make games an integral part of math instruction. One game that has become a standard in my teaching repertoire is Four Strikes and You’re Out. I’ve taught this game at different grade levels to give students practice with mental computation, adjusting the numbers, operations, and number of blanks to be appropriate for the particular class. The game is sort of a mathematical version of the word game Hangman. (If your students know how to play Hangman, it may be helpful to reference it when you teach this game.)

When I introduced the game to a class of third graders, I began as I typically do. I wrote Four Strikes and You’re Out on the board as I explained to the class that this is the name of a game I was going to teach them. Sammy’s hand shot up and asked me the question I typically get.

“Don’t you mean three strikes?” he asked, thinking about baseball.

And I gave my typical response, “We could play the game with three strikes, but let’s try it first with four strikes and then you can see which would be better.” Next I wrote a blank frame for a math problem that I knew the children could solve. And next to the problem frame, I listed the numbers from 0 to 9.

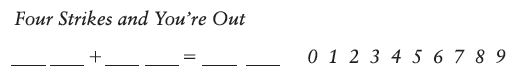
“One number goes in each blank,” I explained, pointing to the six blanks I had drawn,“ and your job is to figure out the numbers in the problem.” I showed the students a folded slip of paper and told them that inside I had written the problem they were to guess. (I didn’t tell them the problem: 35 + 10 = 45.)

“Here’s how we play,” I continued. “You guess a number, and if it’s in my problem, I’ll write the number in all the places it belongs. But, if you guess a number that’s not in my problem, you get a strike. To win, you have to figure out all of the numbers before you get four strikes.”

As with all new investigations, some students were confused. I’ve found that the best way to resolve confusion is to move forward. “There’s no way to know at this point in the game what my numbers may be—you just have to guess,” I said. “But after you make some correct guesses, you’ll have some clues that can help.”

I called on Celia to make the first guess. “Three,” she said. I referred to my “cheat sheet” with the problem written on it and said, “Yes, that’s one of the numbers in the problem.” I wrote 3 where it belonged in the problem and crossed it off the list to indicate that it had been guessed.

“Two,” Hiroshi guessed next. I referred to my cheat sheet and said, “No, there’s no 2 in my problem, so that counts as a strike.” I crossed out the 2 and wrote an X next to the title to indicate a strike.

“Nine,” Amanda guessed next. I referred to my cheat sheet again and said, “No, that’s strike two.” The students groaned.

“It’s good that we have four strikes,” Sammy commented. I wrote another X.

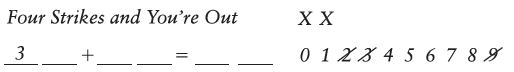
“Five,” Maria guessed. I again referred to my cheat sheet. Even though I had memorized the problem, I checked to model for the students what they were to do after each guess when they later played the game independently.

“That’s a useful guess,” I said, recording the 5 in the two places it appeared and crossing it out on the list.

A buzz of conversation broke out. A few students realized that the two 5s meant that there had to be a zero in the ones place of the second number. Others, however, didn’t notice this. I brought the class back to attention. “Now that you have some clues in the problem,” I said, “talk at your tables about what you now know, and what might be a good next guess. Raise your hand when you’re ready to make the next guess.” After a moment or so, about half the hands were raised. I called on Charlotte.

“There has to be a zero,” she said.

“Can you explain why?” I asked.

“Because you’re adding something to 35 and the answer ends in a 5. So the number you’re adding has to end in zero.” Some students nodded in agreement and others looked confused. I’ve learned that after playing the game several times, more students begin to reason numerically about how the clues can help. This thinking calls for computing mentally, thinking about numerical structures, and helps develop number sense. At this point, I focused on being sure they all at least knew how to play.

“So you’d like to guess a zero?” I asked. Charlotte nodded and I recorded.

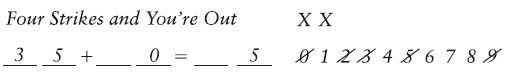
Conversation broke out again at their tables. After a few moments, I called the students back to attention and asked, “Who would like to share an idea about what you now know?” I called on Nelson.

“You can’t be sure about the missing numbers,” he said. “But if you guess one of the numbers right, then you’ll know the other.”

Anna had something else to add. “Eight won’t work in either place,” she said. “Can I come up to the board and show why?” I agreed. She came up and pointed at the first remaining blank. “Look,” she said, “if it’s 35 plus 80, you’d have three numbers in the answer because it would be more than 100.” She then pointed at the blank in the sum and continued, “If the answer is 85, the second number would have to be 50, but we already used up the five.” I could see that some students didn’t follow Anna’s explanation, so I asked the class again to turn and talk at their tables about Anna’s idea and what they might want to guess next.

After a moment I asked, “Who wants to make the next guess?” I called on Tom

“Lucky seven,” he guessed.

I checked the problem and responded, “Strike three.” The students groaned as I recorded a strike and crossed out the 7.

“Let’s see what choices are left,” I said. Together we read the numbers that weren’t crossed out—1, 4, 6, and 8. Max reminded us that eight wouldn’t work, but I didn’t cross it out since it hadn’t been guessed.

“Six won’t work either,” Angela said. “We already know nine is wrong, so you can’t have 35 plus 60, and if the answer was 65, there would have to be another 3, and we already guessed that number.”

“I guess a one,” Beatrice said.

“And the other number is a four. It has to be 35 plus 10 equals 45,” Sammy said.

I recorded the numbers and said, “Let’s check the addition to be sure it’s right.” Everyone agreed that it was correct.

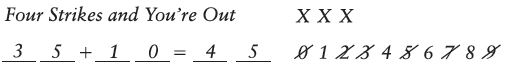
“You figured out the problem with only three strikes,” I said, “so you win.” The class cheered.

I repeated the game for two more problems (50 + 26 = 76 and 29 + 13 = 42). The next day, we played two more games, and this time I changed the number of blanks in one game and the operation from addition to subtraction in the other (37 + 87 = 124 and 70 – 12 = 58). Later in the year, I used problems that involved multiplication and division, choosing problems that related to what the students were learning and practicing (for example, 6 x 5 – 4 = 26 and 5 = 40 ÷ 8).

Tips from the Classroom
This is an example of a game that works well as a whole-class investigation, for the class to play as one team against the teacher. When the students understand how to play, they can learn to play the game independently. For this, I organize the class into partners so that each pair of students could play against another pair. I’ve found that having students work in pairs helps avoid errors in their responses and encourages communication about how they are reasoning.

First I had each pair think of a problem and, as I had modeled, write it on a slip of paper and fold it. On a half sheet of paper, they wrote the blanks for the problem and the list of the numbers from 0 to 9. Then they were ready to play. Pairs took turns, first one pair guessing and then switching roles. I added Four Strikes and You’re Out to the class list of Math Games and it became a favorite option.

I’ve learned that it’s important that students have the skills they need to play any game I teach, whether the game is intended to develop understanding, provide practice, and/or offer challenges. I was careful to introduce the game using an addition problem that I knew the students could solve. When the math is accessible, students can more easily focus on learning how to play.

I’ve also learned that when I first introduce a game, just as when I present anything new in the classroom, some confusion is typical. For that reason, I like to teach a new game to the entire class so that everyone receives the same information. I play sample games as many times as needed to resolve all issues of confusion before I expect students to be successful independently.

Competitive or Cooperative?
Four Strikes and You’re Out is an example of a competitive game where the goal is to win. From their experience playing games like Tic-Tac-Toe, children have learned to play competitive games where someone wins (or sometimes the game ends in a tie). Competitive games help students test their skills, take risks, and learn to be graceful winners and losers. However, it’s important to foster communication and cooperation among students. Having the students play in pairs allows for both cooperation and competition. Throughout the year, I like to teach a mix of games that can be played competitively, cooperatively, or either way.

Note: This blog is excerpted from the Fourth Edition of my book About Teaching Mathematics. The game originally appeared in Teaching Arithmetic: Lessons for Addition and Subtraction  by Bonnie Tank and Lynne Zolli.