What Is 5 Divided by 4? So Many Answers!

Sara Liebert’s fourth graders at John Muir Elementary School in San Francisco have been studying fractions and she was beginning a division unit with them. One of our goals for this lesson was to engage the students in a problem-solving experience that would connect their study of fractions with division, using the context of sharing cookies. Another was to present a division problem that would give the students the opportunity to express remainders as fractions. And a third goal was to give them a problem for which the answer could be expressed in different ways.

Setting up the Problem
We gave each student five paper “cookies” to share and made scissors, tape, and glue available. I wrote this on the board to help them set up their papers, and asked them to describe, both in words and in numbers, how much each person would get.

The Students Got to Work
The students chatted among themselves as they collected the materials they needed, organized their papers, and thought about how to share the “cookies.” Some students worked by themselves and some worked with others at their table. Some began cutting the circles into halves or fourths while others sat looking at them before doing any cutting. Some labeled the pieces they cut and others didn’t. What I love about a problem like this is that students have the freedom to approach solving it in a way that makes sense to them. And the physical nature of the problem, to give each person the same amount, makes it possible for them to have confidence in their solutions.

Sara and I had worried that the problem would be too easy and not interesting to them, that the solution would obvious. That wasn’t the case! Some puzzled for quite a while before they decided what to do. Sara and I circulated―helping, supervising, answering questions, pushing some to finish up, etc. (Ah, the luxury of having two of us in the classroom.)

Organizing a Gallery Walk
It’s difficult during the class period when students are working and we’re circulating to think deeply about what they’ve done and what might be useful for a class discussion. So, rather than summarize the lesson at this time, Sara used the time remaining to organize a gallery walk for students to look at each other’s solutions. This was the first time she had done a gallery walk with this class. She explained the logistics, “When I hold up one finger, clear your desk and have just your cookie paper on it for others to look at. When I hold up two fingers, stand up and push in your chair. When I hold up three fingers, you can begin to walk around the room to look at how other people solved the problem.” She held up one finger, then two, and then three, and the students began to circulate and look at each other’s work.

Examining the Students’ Work
After class, there was a break so Sara and I collected their papers and looked at their work. Some students had written one answer and some gave two. Some cut all the circles into fourths, some cut only one of them into fourths, some mixed halves and fourths. We found six different numerical solutions reported, all correct.

This student cut all of the five cookies into fourths and gave each person five of the fourths. She recorded two options for the answer―5/4 or 1  1/4.

This student cut four of the cookies into halves and gave each person two halves. Then he cut the remaining cookie into fourths. His solution to each person’s share: 2/2 + 1/4.

This solution is similar to the solution above, giving each person two halves and also one-fourth more. This student, however, recorded two numerical solutions: 2/2 + 1/4 and also 1/2 + 1/2 + 1/4.

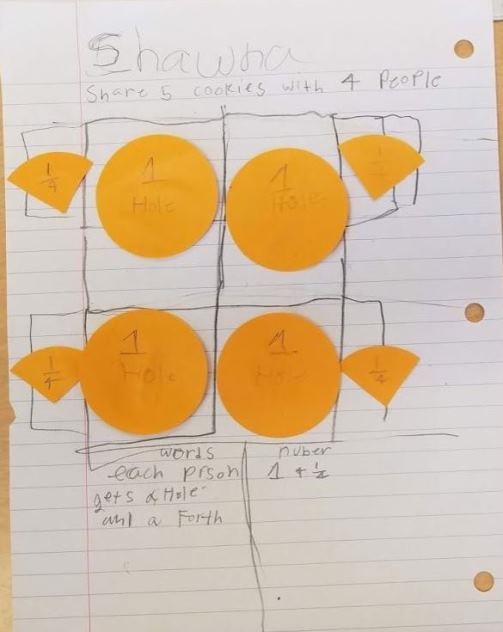
This student doled out four whole cookies and then cut the last circle into fourths. She reported her answer as 1 + 1/4.

I watched this student as she first cut two cookies in half and pasted half in each person’s share. Then, when I returned, she had cut each of the remaining three cookies into fourths and was taping them down. She recorded the solution for each person’s share as 1/2 + 3/4.

What We Did the Day Before the Lesson
In preparation, the day before the students had solved the problem of sharing three cookies among four people. We engaged them with the problem without making the connection to division. Here are samples of their work.

In the first paper, the student gave each person 1/2 and 1/4 of a cookie. The second paper shows each person’s share as three 1/4-pieces.

Before introducing the problem with five cookies, I talked with the students about what they had done the previous day and the different solutions and different representations I noticed. Now I connected the cookie sharing problem to the division work they had been doing and presented the problem as 3 ÷ 4. (See the board work below.) Sara and I think this was a useful discussion before they began the problem of sharing five cookies.

And the Next Day’s Lesson
Sara and I talked that evening. I wasn’t able to come to class the next day, but we thought together about what might make sense to do next. (Ah, the luxury of breaking the solitary aspect of planning.) Our decision was that Sara would list on chart paper the students’ solutions. Then, as she pointed to each one, she’d ask students who got that answer to raise a hand. And then she’d lead a discussion. Our goal was to help students see that all of the answers were correct, that although expressed differently they were all mathematically equivalent. She also wanted to reinforce the connection to division.

After the next day’s lesson, Sara sent me a photo of the chart she created. She had listed the students’ answers and, as they discussed each, she drew a representation of the solution. She also included Clifford’s comment about the solution he had reported.

There’s still lots of work to do with making the connection between this problem and division, with helping students become comfortable with multiple ways to express solutions mathematically, to help them become flexible with different models for thinking about fractions, and to help them understand that fractions are numbers that can answer questions like How many and How much.

An aside: When I was in elementary school, and also in my early years of teaching, I would never have considered the answer of 5/4 to be correct. I would have changed and expected my students to change the “improper fraction” to the “mixed number” of 1 ¼. I’ve long given that up! I hope we can lose the description of “improper” as a description of fractions, once and for ever.)

Where Cookie Sharing Can Go
Sara uses a Math Menu in her class, and the sharing cookies problem is ideal for repeat experiences that can be differentiated to meet the needs of students.

Share ___ cookies equally with ___ people.

Students can choose their own numbers and/or you can make assignments you think are most appropriate. In all cases, even when students seem comfortable, I’ve found it useful and supportive to make the paper circles available and ask for the solution with the “cookies,” in words, and in numbers.

About Planning This Lesson
The experience of sharing “cookies” has long been a standard part of my teaching repertoire. It’s an investigation that I return to year after year because it engages students, they learn, and I learn about how they’re thinking. Each time I prepare to teach a lesson with sharing cookies, I tweak the activity for the particular class I’m teaching. (Isn’t that the way with much of what we do?) This time, before coming to Sara’s class, I read through all that I’ve written previously, where sometimes I used sharing cookies as a fraction exploration and sometimes for division. (See the chronology below if you’re interested in descriptions of my previous classroom experiences.) Then I thought about the opportunity to connect the students’ fraction work with their new division work. And then I thought about the logistics for the day’s lesson. Yes, I’ve taught this lesson for more than 30 years, but I still have to plan each time. Just as there’s never just one way to solve a problem, there’s never just one way to teach a lesson.