I was really pleased to read Jo Boaler’s paper, “Seeing As Understanding: The Importance of Visual Mathematics for Our Brain and Learning.” You can download a copy at the youcubed website.
In her paper, Jo and her colleagues make the case for helping students “be strong with visuals, numbers, symbols and words.” The paper ends with a call to action “to expand the ways we think about mathematics, and to teach it as the visual and multidimensional subject that it is.” I think that the paper is an important read with a compelling message. And a bonus is that it includes specific suggestions for putting research ideas into practice.
The border problem is included in the paper (see page 9) as an example of how to teach students “visual algebra through pattern study and generalization.”
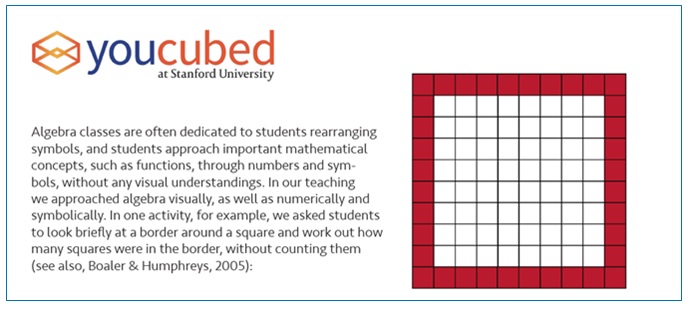
The border problem is one of my all-time favorites, an investigation that I’ve introduced to students many times in classrooms in grades five through 8, typically over a span of several days. If you’ve never tried it, I think that you and your students are in for a mathematical treat.
For a quick visual overview of the lesson, watch this video of me teaching the border problem to Cathy Humphrey’s middle school students. It’s an oldie (as you can see from my hair color). (The video clip is included in the three-DVD set, Mathematics for Middle School, and is included with permission from ETA hand2mind, Inc.)
Also, to provide you support for thinking through the investigation and planning instruction, I’ve made a five-day Border Problem lesson plan available. This is an excerpt from A Collection of Math Lessons, a Math Solutions resource that I wrote with Cathy Humphreys. The resource is also an oldie—first published in 1990—and the chapter presents a detailed vignette about the border problem that includes specific teaching suggestions along with samples of student work.
I hope this serves you and your students. I’m interested in hearing how the investigation goes in your classroom.


What fun to revisit this problem! Even though I’m long retired from the classroom I can still recall the effectiveness of this problem and student’s engagement and success with it in a high school Algebra 1 class. It’s one of my favorites too.
I love this problem – it’s so adaptable and extendible. I did a rectangular version of it (a growing pattern) as part of my NCTM 2016 workshop – so many ways to “see” the relationships; and it’s cool that the center tiles form a quadratic relation, while the border tile pattern is linear.
What a great problem! I was excited to see that the students had many different methods of reaching the solution. This problem really changes the way to think about algebra. I am guilty of thinking of algebra as the task of rearranging symbols and balancing equations, but it is really more multidimensional than that! Visual models can really change a students understanding! It certainly helped me think about algebra in a different way. Thank you for sharing.
I facilitate after school maths clubs in South Africa. I think I found this problem in one of your books and have used it many times with my Grade 4, 5 and 6 students as a ‘pre-algebra’ task – a way of introducing the students to the ideas of working with letters and equations. It is a wonderful problem and can be developed in so many ways – one of my favourites. I was also glad to see it in Jo Boaler’s visual maths article.
I have not used or seen this particular problem, but what a great way to introduce the students to visualizing and working with variables.
I love the multiple approaches to this problem. Your questions guided students to justify their own thinking. The number sense grew for the entire classrooms. I could tell that students felt confident in sharing their individual methods. I loved the connection to writing the algebraic formula from a geometry problem. This provided multiple modes of learning.
This is a great review for those who get stuck in a rut. They can incorporate the students in the lessons and have them work out how to share the process with others. Letting the student share their thought process may help someone else understand. I have found once I reached out 4 or 5 times with different ways to do a problem I let the student explain it. The other students then understand the process used and can incorporate it into their successful understanding. It also offers students a chance to use their understanding to become class helpers who can help others gain confidence in their skills.
very informative review! i glad that i bumped into this blog since I’ve never seen this kind of problem. thank you for these amazing lessons since this will help how students can mold their understanding of simplifying a problem to solve efficiently. thank you