My goal for the lesson was to see if students would agree or disagree with 13/20 as a number that represents how much of the grid was shaded. Also, I was interested in how students would explain their reasoning. To that end, I planned to give them this writing prompt:
Mr. Chubb said the shaded part of the 100 grid is 13/20. I agree/disagree because ______________________________________________________.
[An aside: Phil Daro and I have been thinking recently with Lucy Calkins and her reading/writing team about crosswalks between ELA and math instruction. One obvious crosswalk to investigate is the role of writing in both areas. For this lesson, I planned to have students write what might be similar to a persuasive essay that would support their thinking about fractions and decimals in the context of this image. However, I wasn’t aiming for students to prepare essays for publication, as they do in writing workshops, but rather giving them an experience to focus on what William Zinnser wrote in Writing to Learn: “Writing is a way to work yourself into a subject and make it your own.” I was more interested in their writing giving me insights to their understanding. As students wrote, I would encourage them to revise, clarify, rethink, and so on, many of the same ways I might intervene with students engaged in a writing workshop.]
Before I presented the writing assignment, I planned to lead a number talk. This would help me set up the problem, and would also give me the opportunity to assess whether the assignment was appropriate for the class. After an introductory number talk, I’d decide if the assignment could help to develop, cement, or extend students’ learning. My questions:
- Do all students have access to the problem, and does the assignment allow for different approaches?
- Do I need to do more scaffolding before giving students the problem of thinking about Mr. Chubb’s conjecture?
- Or should I plunge in, see what happens, and build from there?
[A second aside: I’ve thought often about what the difference is between a number talk and a lesson. Typically, number talks are shorter, and I planned for this introduction to take about 15 minutes. So I guess it fits into the category of “number talk” from that perspective. But it wasn’t a stand-alone experience, but rather would serve as the introduction to a longer lesson that would include the writing assignment and a discussion after that. I’m honestly struggling with what differences there are between number talks and lessons beside the time constraint. But I’m happy to call it whatever.]
My Lesson Plan
The teaching plan I came up with was to show the students five images, one by one, and discuss each.
Image#1

I’d present this image to establish that this was a 10-by-10 grid with 100 squares.
Image #2
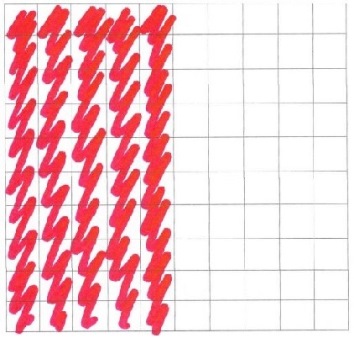
For this image, I planned to ask, “What number could I write to describe how much of the grid is shaded?” I would use students’ responses to test my assumption that they were familiar with the idea of one-half and would see that I had shaded in one-half of the grid. I was curious about their comfort with different numbers being possible; e.g., 1/2, 50/100, .5, and so on.
Image #3
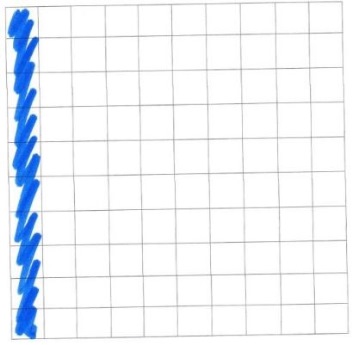
I planned to ask the same question for this image.
Image #4

And, again, I’d ask, “What number could I write to describe how much of the grid is shaded?”
Image #5
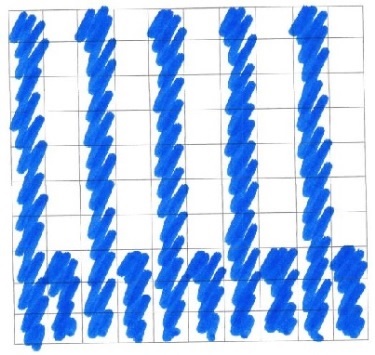
And, finally, I would ask the question for this image.
That would be the end of the “number talk” part of the lesson. Then I’d show the class to the image I saw on Twitter, with 13/20 written below the grid, and present the writing assignment I described above.
Results from the First Class
Using a document camera, I projected the images on the white board, one by one. For each, I asked students to turn and talk to a neighbor before I called on students to report to the class. Discussions for all of the images were lively. When students suggested numbers, I wrote them on the board and asked the students to explain their suggestion.
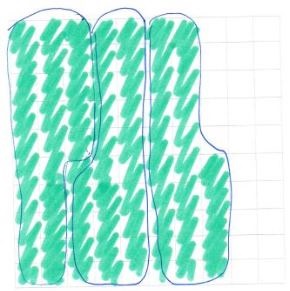
When I posted Image #4 with 75 of the 100 squares shaded, students suggested two numbers that I hadn’t anticipated: 7.5/100 and 75/102. For 7.5/100, the student explained, “There are seven and a half columns of the ten columns shaded.” For 75/102, the student explained it was the same as 75/100, but he gave an alternative, using an exponent, to represent 100. Another student suggested 3/4 as a fraction equivalent to 75/100, but wasn’t sure how to explain why. I told the class that thinking about money and quarters helped me connect 75/100 and three fourths or three quarters, that I could see three chunks of 25 each in the shaded part (I marked the image as shown below), and three 25s is 75, and 75 cents is equal to 3/4 of a dollar, which is 100 cents. I’m not sure the way I drew on the image was useful for all of the students.
I moved on and showed Image #5:

For this image, most students counted the number of squares shaded and suggested representing the shaded area as 65/100 and .65. One boy thought that it was about 2/3. That resulted in a discussion about decimal equivalents of 1/3 and 2/3, and also gave me the opportunity to introduce the symbol of two wavy lines instead of the two straight lines as in the equal sign to indicate that 65/100 was “about” equal to 2/3.
During the class discussion, I heard a variety of ideas from some but not all students in the class. This is typical, I’ve found. That’s why I think it’s important to ask students to produce individual work that can help me learn what individuals think and understand.
To introduce the individual assignment, I projected the image from the Tweet and wrote the starter for their writing below it:
Mr. Chubb said the shaded part of the 100 grid is 13/20. I agree/disagree because ______________________________________________________.
The students began writing. As they thought and wrote, I circulated and talked with students individually, prompting some to clarify an idea, answering questions for others, asking others to tell me about what they were thinking and then encouraging them to get their ideas on paper. In this class, 15 agreed with Mr. Chubb, 5 disagreed, and 3 disagreed initially but then changed their minds.
Rethinking before Teaching the Second Class
Before teaching the lesson again, I exchanged a few emails with the second class’s teacher. I sent him what I had written about my experience with the first class so that he had a sense of what I was planning to do. He was currently working on decimals with his class but hadn’t yet focused on fractions. He wrote: Keep in mind that I have not started any sort of fraction and equivalent fraction work with the class yet. Many of the kids will remember their work from 4th grade but there will be some who are very confused by the idea that 65/100 can be seen as 13/20. Will be interesting to see how they work through it.
After thinking about his message, and before teaching the lesson, I prepared two additional images.
Image #6
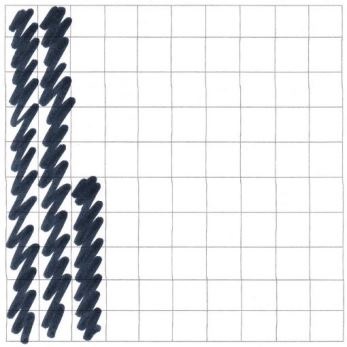
I thought that perhaps this might be a good substitute for Image #4 that had 75 squares shaded. I thought that perhaps 25 squares would be easier to relate to money and to the idea that 1/4 would be an appropriate number to represent what was shaded.
[A third aside: I think that helping students use benchmark numbers to reason numerically is important. We typically think of 10 and multiples of 10 as being especially useful for reasoning with whole numbers, and I agree. I also think that 25 and multiples of 25 are useful, numbers that our money system helps make accessible to students. I look for opportunities to engage students in using 25 as a benchmark.]
Image #7
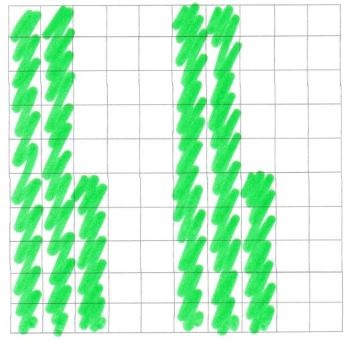
I wondered if this image would be helpful to scaffold to Mark Chubb’s image because I thought that students could more easily see that this image could be represented by 50/100 and ½, even though the squares shaded weren’t contiguous. I also wondered if this would be leading too much or if instead I should let students grapple.
When I drove to school to try the lesson, I hadn’t completely resolved what I’d do. For sure, this isn’t a lesson where I’ve polished the stone enough so that I’m confident about anticipating what students will do and deciding what might be the best teaching moves on my part.
Or are there “best” teaching moves?
And does teaching ever get easier?
Results from the Second Class
I felt more relaxed in this class, not because I had met these students previously, but because of my previous experience teaching the lesson. I felt better prepared to pay attention to students’ responses. I led the lesson in a similar manner.
An unplanned disruption was that after the students had looked at three images, a message appeared that the document camera was overheating and I had to turn it off immediately! I didn’t panic. Magnets were available for me to post the images on the whiteboard. And since the students were gathered on the rug as they as they usually are for math, each next to his or her pre-assigned math partner, they were close enough to see what I posted.
The students’ responses were similar to those for the images I had showed the first class and produced some interesting reasoning. For the new Image #6, students suggested both .25 and 1/4. When I showed Image #7 on the chalkboard (see below) students had a variety of suggestions.

One student suggested 5/10 and explained, “You have four full columns and they are one tenth each and then the two short columns make one more tenth, so it’s five tenths all together.” Another student pointed out that you could add 0.25 and 0.25 to get 0.50, which was the same. A third student said that 1/4 plus 1/4 was 1/2. Another offered 1/2 as a possibility since 50 of the 100 squares were shaded. Another said you could use 32/64 since it’s equivalent to 1/2. I recorded all of them on the board next to the image.
[A fourth aside: When the boy suggested 32/64, I acknowledged that he was mathematically correct, but that I couldn’t see how 32/64 related to what was shaded. He shrugged and held to his claim that 32/64 was possible. I’m not sure how much I should have pressed with this. Ah, decisions, decisions.]
Next I posted Image #5, from the previous lesson.

When we talked about this image, the students suggested equations that hadn’t come up in the other class, focusing on ways to figure out that 65 squares were shaded. After this, I presented the problem to them as I had done in the first class, posting the image from the Tweet, giving the writing prompt, and explaining that is was to be a first draft for a persuasive essay.

The students began writing. In this class, 10 agreed with Mr. Chubb, 11 disagreed, and 3 weren’t sure.
Results from the Third Class
When I taught the lesson in the third class, I decided to use the presentation as I did with the second class. From my experience with two other classes, I had a better sense about what to anticipate from students’ responses. Also, because I was more familiar with what I planned to do, I didn’t have to pay as much attention to my lesson plan. This enabled me to direct my energy into paying attention more to what the students were doing and thinking more than to what I had planned to do next. This resulted in my slowing down the conversations and hearing even more from students.

I slowed down our class discussion so much that I never got to the 13/20 image and the writing assignment I had planned and done with the other two classes. That would have to wait for another day. But this lesson was useful for setting the stage for the writing assignment and learning about what the students were thinking.
I’m Stopping Here
I could write more about the decisions I made in each class, but this blog has gone on long enough. If you have questions about how I arrived at any of the teaching decisions I made, or any suggestions, feel free to post your ideas and I’ll do my best to respond.
One Last Comment
I described my experience with this lesson in the talk I gave on December 2, 2017, at the California Mathematics Council North Conference at Asilomar. If you’ve never attended this conference, consider coming. The venue is beautiful and the conference never disappoints. I had a slew of conversations about the 13/20 problem, with people questioning, wondering, and searching with me. One friend said that she remained uncomfortable with 13/20 being a number to represent Mark’s image, in the same way I was uncomfortable with a boy’s suggestion of 32/64 for Image #7. Her suggestion for a way to show 13/20 was this:

She feels this image better helps relate 13/20 and 65/100. What do you think?
To view the original Tweet from @Mark Chubb3, click here.

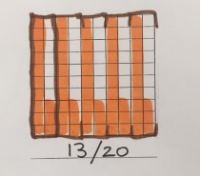
This is a perfect example of showing how to simplify fractions using a grid. Awesome!!
Hi Marilyn,
Thank you for sharing again. I love the focus on using the student work to guide and adapt further lessons. I think the conversation you had with the second teacher is super important. He said he has not done any fraction work this year with his students. Many resources are clear that students need to have been introduced to fractions first and have clear understanding of fractions as a number/quantity along with developing fraction sense before they are introduced to decimals. That decimals are just a base ten representation of fractions that allow us to work with fractions on more friendly terms. I often see teachers that I work with starting decimals before doing any work with fractions, especially operations. Our Ontario curriculum has adding/subtracting decimals two grades before adding/subtracting fractions. What do you think about that? Do you see teachers focusing on decimals too early before their students have strong fraction sense? Or do you think its ok to start decimals before fractions? Thanks!
Thanks, Mark, for your comment. About fractions, I agree with you completely that a firm foundation of fractions is essential before focusing on decimals with students. I have seen approaches where place value is used as the foundation for introducing decimals, but for me I’ve always taken the route first that decimal numerals are another way to represent a certain class of fractions. Actually, neither of the first two classes I taught had focused on fractions yet with their fifth graders, and this was in two different schools using two different programs. Both were teaching about decimals and relying on the students’ experiences with fractions in fourth grade. I hope to revisit both classes later in the year.
About adding/subtracting decimals: My worry is that the focus in on the algorithm, and the algorithm is introduced before adequate attention to developing understanding. If the goal is on the procedure, then I think that it’s easier to get kids to add and subtract decimals than it is to add fractions (as long as they can add and subtract whole numbers), and I worry that students can crank out answers without a robust understanding of what the numbers mean and why the method they’re using works. It’s sort of the same as teaching students to multiply fractions by multiplying the numerators and denominators, and they’re successful doing that without really understanding why it works.
Also, I’ve met many second graders whose understanding of place value with whole numbers is shaky, so focusing on adding and subtraction decimals doesn’t make sense to me. (About place value, you might be interested in the videos on a previous blog: )
Great conversation here Marilyn and Mark. I would tend to agree with Marilyn in that students’ understanding of topics like place value, (whole numbers and decimals) and fractions are often procedural, especially when students are expected to do an operation with those numbers. What I see as important is making sure students are gaining a relational understanding of these concepts. This would require various representations for each.
Knowing how to add decimal numbers without understanding the magnitude of those numbers doesn’t make sense to me. Work with number lines, and arrays help us visualize what is going on. Relating decimals and fractions is essential to a students’ overall understanding!
Hopefully, before we ever do any operations with numbers, students have a solid understanding of those numbers. Otherwise, students lose any ability to sense-make.
Hello Marilyn,
After seeing your blog link on twitter, I taught this same lesson to a 5th grade class in our district. Most elements of the lesson were successful, but my results did not reflect the amount of learning that was happening. Of the 24 students that participated, 24 disagreed with Mr. Chubb. One factor was the way we posed the conjecture. We used Google Classroom. There is a feature that will show responses from 3 other students once you submit your response. A few students responded quickly and students near them then saw that all of the students that responded so far had disagreed. Also, we overlooked a need to provide an option for students who were not sure.
Hindsight being 20/20 vision, we would definitely revise the way we have students respond. Also, we would try to address the equivalence of fractions that some students were missing.
Thanks for the work that you do and the insights that you share. 🙂 Caroline
I’ve had a similar experience in classes when a few students express an opinion and the rest jump on board. This is especially true when the students have status in the class as being “good in math,” and they typically are the ones who answer first. I’d be interested in learning about how you’d revise the lesson and how you had students respond. I’m still mulling. And, yes for sure about focusing on equivalence of fractions. If you have more experience, please let me know. Thanks for your comment.
I have recently retired from teaching, but I have always been inspired by your books and blog.
Here are some ways I like to deal with problems of less confident children ‘jumping on board’ with the ‘confident’ children. Instead of allowing the ‘good’ students to answer first, I use your technique of letting students talk with their neighbour or partner, then say ‘Hands down, I get to choose people to share their thinking.” This raises accountability greatly, and because my students know that mistakes in my class are always OK, they become quite comfortable with sharing their thinking. The ‘good students’ are not the focus, but rather THINKING and COMMUNICATING ideas are. I find that there is a lot more thinking going on, when they are communicating with their partner before the class discussion. I especially like to call on the quiet kids who might have some great ideas, too, even if not totally correct, and I’m able to get them to take chances in front of the class.
If I want to see whether they agree/disagree with a given statement or different ideas that are recorded on the board (as a quick formative assessment of how each child is doing), I number each option (eg., 1 = yes, 2 = no, 3 = unsure), then give 30-60 seconds of ‘Thinking Time’ for everyone to decide their own response. They must be able to justify their response, not just choose any answer. They are not to tell anyone their choice at this point, and when I say, “One…, two…, SHOW ME”, each child holds up that many fingers against the front of their chest, so that basically it is only me that can see their choice. Again, I can ask anyone to justify their choice and explain to the class.
Regarding your friend at Asilomar, I wonder if it might help to suggest that she try cutting up Mark’s image to see if it will fit over top of her image. Merry Christmas!
Thanks for your insights and tips. Enjoy being retired!
Thanks for sharing. I love the idea of a persuasive essay and would love to see examples, both successful and not so successful, of what the students wrote. I suspect that going in with the idea of writing a persuasive essay rather than merely explaining their thinking would increase the volume of writing and take it beyond a number sentence. Writing is indeed a learning tool.
As far as Mark’s image, I also love that. Showing values in unexpected ways increases flexibility in thinking. I am anxious to try this with students, but am confident that they will see that two columns of the grid include 20 squares and that 13 of those squares or 13/20 are shaded.
32/64 as a representation on a 100 grid is another interesting conundrum. Though it is indeed an equivalent relationship to .5. it is very difficult to see in the image. Am I correct in thinking that the least common denominator of 20 and 64 is 320? That would mean, in order to picture 32/64, each of the squares in the grid would need to be broken into 16 equal sections and then regrouped into sections of 4 original squares to get 64 little sections of which 32 would be shaded. So we are now showing in the entire grid, 25 sections of 32/64 similar to Mark’s representation 5 sets of 13/20. I think? So perhaps that would be the next step for Mr. “Think Ourside the Box.” As an enrichment opportunity, say to him. “I wonder how we could use this 100 grid to represent 32/64 in a way that we could count the sections? (1 inch grid paper may assist in this endeavor.) Maybe some of you could contemplate that and chat with me about it tomorrow before class.” I am guessing most of the students would be confused by the idea so to keep everyone engaged, I am thinking I would move on at that point. But I am grateful when students come up with their own enrichment activities.
I’d love to hear what you find out if you try this. Also, I’ve received a few requests curious about what students wrote. I’ll sift through and post some samples of what they wrote. But first I’m taking a break for the holiday and will do this early in the new year.
It’s fascinating that a student would mention 32/64. Perhaps in the past he noticed that a chessboard has 64 squares, and it seemed that half of them were white and half were black, so he counted them to investigate? He then realized it didn’t matter how you grouped them. As long as 32 out of 64 were white, that would always be equivalent to 1/2. Who knows? That the beauty of math, there are so many ways to approach a problem!
I hadn’t thought about that possibility. I agree — so many ways to look at a problem. Thanks for the comment.