17 Kings and 42 Elephants by Margaret Mahy is one of my favorite children’s books. It’s a poem that uses rhythmic and rhyming language to describe a royal procession through the jungle. I love the playful text and the vibrantly colored batiks that illustrate the journey.
I’ve never been particularly interested in memorizing poems, probably a result of being miserable when I had to memorize a Shakespearian sonnet and a Miltonian sonnet in high school. The assignment was supposed to make clear the difference between the two forms of sonnets. It didn’t work for me. All that’s left from that experience is that I can recite, on demand, even after two glasses of wine, the beginning of one of the two poems I had to memorize: When in disgrace with fortune and men’s eyes, I all alone beweep my outcast state. That clearly depressing start is all I recall about the poem, and I still have no idea about the two different sonnet forms.
But I digress.
I decided a while ago to memorize 17 Kings and 42 Elephants so that I could present the book to students without the distraction of fumbling as I turned the pages to show the illustrations. I didn’t want to break the rhythm of the journey and I wanted to be able to hold the book so students could examine the batiks as they listened to the poem. Here’s how the book begins:
Seventeen kings on forty-two elephants
Going on a journey through a wild wet night.
Baggy ears like big umbrellaphants,
Little eyes a-gleaming in the jungle light.
The text is full of made-up words like umbrellaphants that serve to preserve the rhymes ―rockodiles, hippopotomums, twangling trillicans, baboonsters, and more. These words offer a good way to talk with students about being playful with language and about the idea of poetic license.
I digress again. OK, now to the math.
Presenting a Math Problem
I’ve typically read this book to third graders but recently presented it to fourth and fifth grade classes. No matter the grade, I typically read the book through twice and allow time for students to express their thoughts about it before shifting to a math lesson. And no matter the grade, I begin by presenting the same math problem: How do you think seventeen kings can equally share the responsibility for taking care of forty-two elephants? Depending on where the students are with studying division, I may write 42 ÷ 17 on the board. Or, for classes beginning to learn about division, I may wait until later to connect the problem to the symbolism of division.
Sometimes I ask students to work on their own and at other times I ask them to work with a partner or small group. When they work collaboratively, sometimes I have pairs or groups produce one paper or poster that shows their solution and how they figured it out. At other times, I ask students to write their own paper on which they record the answer and explain how they reasoned. After students complete the assignment, I sometimes have a gallery walk so that students can look at each other’s solution strategies.
A Note about Division
Division is hard. It’s hard to teach and hard for students to learn. That’s true, I think, for a variety of reasons. Contexts for division can be confusing, either sharing situations (like this problem) or grouping situations. Interpreting remainders can be confusing. (For 6 ÷ 4, for example, when sharing 6 cookies among 4 people, 1½ makes sense as the answer of how many cookies each person gets, but when sharing 6 balloons among 4 people, 1R2 indicates that each person gets 1 balloon and there are 2 extras.) Depending on students’ number sense, figuring out answers can be confusing. Even when division is connected to multiplication (as I think is best when introducing division), figuring out the missing factor can be a numerical challenge, especially when problems involve two-digit divisors.
The Lesson in Grade 3
Division is typically introduced in third grade, and instruction focuses on representing and solving problems that involve division, relating division to multiplication to see division as an unknown factor problem, and dividing within 100. Problems with two-digit divisors aren’t typical for beginning division instruction, but the problem of figuring out how 17 kings share taking care of 42 elephants is a good challenge. Not only does the math provide a good problem-solving experience, figuring out what to do with the remaining elephants engages children’s imaginations. This lesson is described in Teaching Arithmetic: Lessons for Introducing Division, Grades 3–4 in Chapter Six, “17 Kings and 42 Elephants.” You can read the lesson here. Below are two samples of student work you’ll see in the chapter:
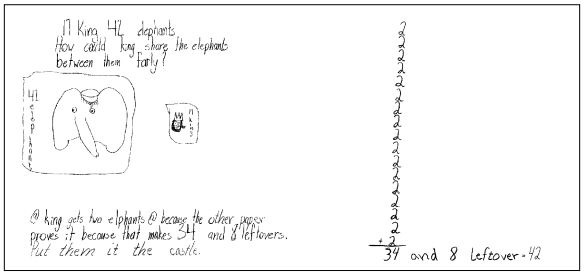
These students used addition to solve the problem and made the decision that the Kings could put the extra elephants in the castle.

These students solved the problem with tally marks and recommended sending the extra elephants to the zoo.
Extending the Problem in Grade 4
The problem is also appropriate for fourth graders and the lesson can be extended in a way that provides useful insights into students’ number sense. After students solve the problem of 17 kings sharing 42 elephants, they consider other numbers that could be used to make the kings and elephants problem either easier or harder to solve. Eliciting students’ opinions about other problems is an informal way to assess their numerical comfort.
I suggested this idea to Nina Sudnick who teaches math to two classes of fourth graders in Athens, Ohio. Nina and I “met” on Twitter a few years ago and since then have been communicating about her classroom teaching. For teaching division, Nina has been relying on the Teaching Arithmetic book mentioned above and also on its companion, Teaching Arithmetic: Lessons for Extending Division, Grades 4–5. I suggested to Nina that she present the problem about 17 kings sharing 42 elephants, and then try an extension. She and I had a telephone chat about how to structure an extension investigation. (Planning lessons is always easier to do with another teacher.)
Extension Ideas
After we talked I sent Nina a message summarizing our conversation and ideas.
- Ask the class: What numbers could we use for kings and elephants so that the problem would be easy to solve? List their suggestions on the board and ask why the numbers they chose would make the problem easy.
- Then ask: What numbers could we use so that the problem would be hard to solve? Again, list their suggestions on the board and ask why the numbers they chose would make the problem hard.
- Follow this with an assignment for them to do individually: Create six problems, three easy and three hard, and solve them. Maybe have them mark the problems E or H. (Ah, the details of planning.)
- As a further extension: Think of a problem that’s too hard for you to solve but that you’re curious about.
Nina Reported Results from Her Classes
A few days later, I received a message from Nina. Her students had solved the initial problem and she had tried the extension. Nina wrote:
In both classes I asked students to think by themselves about an easy kings and elephants problem to solve and about a hard problem. I then went around the room and asked each student to suggest a problem, asking certain students to give me their easy problem and others to give me their hard problem. (I tried to ask them for an easy or hard problem based upon their comfort level with math.) After we went around the room once, I asked if anyone wanted to add any other easy or hard problems and several students added on.
Next we talked about what made a problem easy or hard and I recorded their ideas on the whiteboard. They are wise beyond their years! Finally, I asked them to create three easy problems and three hard problems but they had to be able to solve all the problems. As you predicted, it was an excellent formative assessment and I got to see the variety of strategies they are now using to think about division. The students were focused on their work and very proud of it. I think they really liked the contrast of easy vs hard.
Nina took photos of the white board after each lesson. In the students’ reasons for why problems are easy or hard, the students use vocabulary that Nina has been using with them in class―known facts, landmark numbers, dividends, divisors, factors, and more.

Nina wrote “e” or “k” after the numbers in the problems so that students kept in mind the context of kings sharing elephants.

Some students enjoyed using large numbers (350 and 1,000,000) for their easy problems.
An Observation
It was interesting to me to notice that odd numbers came up in both classes as contributing to problems being hard. In the first class above, a student thought that “dividing even by odd” would make a problem hard. That made me think about problems like 6 ÷ 3 and 10 ÷ 5. I wonder if the student would rephrase that after thinking about examples like these. Discussing this conjecture with the class would be way to address Mathematical Practice Standard # 6: Attending to precision. I used to think that this standard was about getting exact answers, but I’ve come to understand otherwise. Two statements in the description of this standard particularly apply:
Mathematically proficient students try to communicate precisely to others. . . . In the elementary grades, students give carefully formulated explanations to each other.
From a Grade 5 Class
For the past several years, I’ve been collaborating with Sara Liebert in San Francisco, California. From time to time, Sara, Nina, and I share ideas, and this was one of those times. Sara also agreed to try the kings and elephants lesson with her students. She and I decided that Sara would present the book and the initial problem to her class, and I would visit the next day and introduce the extension activity. (Sara taught these same students as fourth graders and I’ve regularly visited this class over the past two school years.) To solve the problem with 17 kings and 42 elephants, students worked together and, as is typical, they solved the problem and presented their solutions in in different ways.

These students drew circles to represent the kings and dots to keep track as they shared elephants.
After the students had time to solve the problem, groups shared their ideas and Sara recorded them. She sent me a photo of the board at the end of class.

One group made a poster of their solution and Sara posted it during the class discussion. Also, look at the two equations at the bottom left. Unconventional for sure, but correct or incorrect?
Following up the Next Day
Sara and I talked about how I might extend the problem. After we reviewed the idea I had sent to Nina, we decided to take a slightly different direction. Sara uses a Math Menu in her class and we were trying to think of a way to extend the problem that would also lead to adding a new option to the menu. (You may be interested in “Using Math Menus,” an article I wrote for Educational Leadership in the October 2016 issue.) We decided that after students created and solved their own problems, they would use them to make problem cards for their classmates to solve.
To begin the lesson on the second day, I wrote on the board:
How can ___ kings equally share taking care of ___ elephants?
(17, 42)
I read the problem aloud, “How can blank kings equally share taking care of blank elephants?” Then I explained how the pair of numbers described the problem they solved yesterday. (This notation isn’t essential but it helped me avoid having to rewrite the entire problem each time for new numbers.)
I wrote (15, 42) underneath (17, 42) and reread the problem using these numbers, “How can 15 kings equally share taking care of 42 elephants?”
Then I elicited problems from the students. First I asked for an easier problem, referencing younger students, “What might be a reasonable kings and elephants problem for third graders?” After they talked at their tables, I called on one student to share. She suggested 6 kings and 24 elephants and explained, “It doesn’t have a remainder and the numbers are pretty small.” I recorded the suggestion on the board and the equation.
Then I asked, “What might be a reasonable kings and elephants problem for seventh graders?” After they talked at their tables, I wrote down one of their suggestions―2,327 kings and 56 elephants.
Then I asked them for problems that would be reasonable for fifth graders, and I listed three of their suggestions.

The fifth graders suggested kings and elephants problems that they thought would be appropriate for third, fifth, and seventh graders.
Sara and I decided that each student should each create and solve three problems that they thought were good for fifth graders, and that only one of their problems should have no remainder. We gave them the rest of the class to work on this in their notebooks. They worked hard but didn’t have time to complete the assignment.
Sara Reported after the Next Day’s Class
After class, Sara and I reviewed the students’ notebooks and talked about how she might continue the next day. I wasn’t able to return to class, but Sara sent a message to describe what happened:
Today’s lesson also went wonderfully. I decided to start class by showing a problem written with the divisor and dividend reversed. For 18 kings sharing 48 elephants, one girl had written 18 ÷ 48. Without using her name, I wrote the problem on the board and asked the class how they would solve this kings and elephants problem. They all assumed the problem was about 18 kings sharing 48 elephants and finally a student noticed that the problem was set up with the numbers reversed. We had a good conversation about why commutativity works for multiplication but not for division.
From there, I shifted into the plan for the day. I showed them pages from two students’ notebooks that showed problems so that the students who had completed the assignment could extend their work by either writing and solving a similar problem or finding a different strategy for solving the problems I showed. (Actually, only two girls were done with the assignment and this was because they hadn’t pushed themselves to create problems that were challenging for them. I know they are competitive, so once they saw that their classmates were solving more difficult problems, this intrigued them and they got back to work.)
Then I explained to the students how they were going to use their work to make problem cards. I created an example of how they could “publish” their problem to model for them what they were to do. (I purposely used the word “publish” to mirror the language we use when we publish in writers workshop.) On one card I had written two problems, and on the other card I wrote the solutions and explained my strategies. Then I gave students two cards and assigned them each a number to put at the top of both their card so that when the problem cards are a menu option, they can locate the corresponding answer cards. I’ll look over the students’ cards first to make sure their answers are correct.
I’m just getting a chance now to really dig into their work, but I just loved this lesson. Allowing students to create their own problems really set everyone up to have access to the lesson and experience some success and status. I think I’m really going to learn a lot from seeing the number they chose and how they are solving the equations.
Samples of Students’ Problem Cards for the Math Menu

This student used multiplication to figure out the answers to her division problems. It was interesting to me to see how she used a known fact, 7 x 8, to figure out 56÷ 14.

This student used a combination of multiplication and division to solve her problems.
Thoughts about the Two Different Extensions
I honestly don’t know why I didn’t try the same extension activity that Nina and I had planned with Sara’s class. Somehow I felt that the references to third, fifth, and seventh graders was a clearer way than “easy” or “hard” to define for the fifth graders what I meant by different sorts of problems. What will I do next time? I’m not sure―I like both options. Making decisions like these is truly at the heart of planning lessons and there isn’t one right or best way to structure the way students work. That said, I think it’s always valuable to include a whole class discussion afterwards about students’ reasoning and choices for presenting their ideas.
A Last Note: Dealing with the Extra Elephants.
When I’ve presented this problem, typically students come up with a variety of suggestions for how to deal with the extra elephants. In Sara’s class, after solving the problem, two girls wrote a story to describe their idea about how to deal with the problem of the extra eight elephants. Their story explains that a different king and queen across town had nine elephants. The last page of their story explains that those nine elephants escaped and came into the possession of the original 17 kings, giving them 51 elephants in all, which meant that each king could have three elephants to take care of. They presented their story which gave us the chance to talk about 51 being divisible by 17.

After figuring out that 17 x 3 = 51, the girls knew that if the Kings had nine more elephants, they could each take care of three elephants and there wouldn’t be a remainder.


Marilyn,
The is no “End” to any of the problems you present.
You always provide extensions which is the true art of investigating
a problem.
With much appreciation for continuing to challenge all of us!
Marsha
Hi Marilyn,
I have been doing a lot of work with teachers to help move their students from additive thinking into multiplicative thinking at the schools I work in. We are running two different PLC’s in this area. You mentioned it above about how we should relate division to multiplication when introducing it which I agree with. I was wondering your opinion about some research I have been reading in order to bring forward at the PLC’s I am running. The article is from Australia and its by Chris Hurst. The article’s title is called “The Multiplicative Situation.” I have found it to be fascinating. He is advising that we call it one situation “the multiplicative situation” instead of teaching it as two entities. He says it is easier to teach students one situation instead of two. He breaks it down to three key areas. 1) Understanding the three parts of the situation- total amount, number of equal groups, amount in each equal group. 2) Understanding the Array (it allows all three parts to be seen at once) 3) The language- factors, multiples etc. Then he speaks to understanding the properties thoroughly leading to the distributive property being a students friend forever. The article also connects the situation to fractions, area, rate and combinations. I have always advocated with the teachers I work with as a coach to not teach it as two different units but I thought this article even makes it clearer. I liked the idea of one situation. I would love to hear your thoughts and it just so happened your latest blog post is on division! I love how you lead your lessons from stories and look forward to hearing your thoughts. Thank you for sharing.
Thanks, Mark. Chris Hurst’s ideas are interesting. I know that there’s no best way to teach, but I lean towards giving students a grounding in multiplication — with equal groups, arrays, and contextual situations — and then introducing division as another way to look at similar situations. In division problems, the missing factor can be either the number of groups or the number in each group, and I find it best to have students use what they know about multiplication to make sense of division. Of course, the issue is how to make instructional choices that help develop understanding and skills while building number sense. I’m open to any classroom ideas to help with that.
Really like all of this. Some thoughts:
(1) I remember, as a student, getting the direction to create problems and then solve my own problems. The inescapable temptation was to create problems that are very easy, so solving requires no work. Also, I would never create a problem that was truly hard (beyond my ability to solve it). I was surprised that wasn’t an issue in either class. Maybe I’m just uniquely lazy?
(2) The equations you flagged in the 5th grade picture are intriguing:
42/ 17 = 2R8
42/2R8 = 17
Clearly, we would like to preserve equivalence of the equations a/b = c, a/c = b, and a = b*c (be careful about zeros!) The remainder notation (2R8) seems to interfere with that. Or, is there a sensible way to extend integer arithmetic to these new objects?
(3) Peter Liljedahl has some nice fair sharing activities (see Primary K-3 section). I’ve had great success using these. Even very young children seem to have a sophisticated sense of fairness and a deep interest in fair division problems. Their personal experience helpfully informs their interpretation of the problems and also helps them be quite creative in finding solutions to some tricky problems.
Which leads me to …
(4) I’m slightly surprised that none of the students came up with a solution to the 42 elephants for 17 kings problem involving time. Apologies if the reasons for this are obvious from the story in the book (which I haven’t read).
To explain with a similar example: at our house, we need to set the dinner table with five places. There are three kids who are responsible for this task. How do they divide it? They don’t calculate
5/3 = 1 R2 and scratch their heads. Instead, one of them says: you (first sibling) do it tonight, then you (second sibling) do it tomorrow, then I’ll do it, then we repeat.
The point is that this task needs to be done every day, presumably like taking care of the elephants, so an exact and equal division over time is possible.
Thanks for your comment and for the link to Peter Liljedahl’s site. I look forward to digging in. About the issue of solutions that involve time, one boy in the fifth grade class had an elaborate solution for kings rotating when they had responsibility for elephants. He got a grid for a calendar and worked hard to figure out a schedule that could work. Thanks again for your comment.
In my classroom we are working with reading strategies to help us solve story problems. I love your idea of having the students create easy and hard problems and then talking about why they are easy or hard. I am going to have my students write an easy and a hard story problem with a given equation with these directions, “write one easy and one hard story problem that uses the expression of 652 + 41.” Then we will talk about what makes it hard and what makes it hard. My goal is that students can understand there are key words in some story problems that make them easy but other story problems might be “hard” because they are not laid out for you. I am going to start this idea tomorrow and I am not sure how long it will take, sometimes writing story problems takes time.
I’m interested in how your plan unfolds with your students. And I agree that writing story problems can take time. Sometimes I have to remind students that their problems have to end with a math question that tells us what we have to figure out. Good luck with this.
This was a fun lesson to teach. After the students wrote their hard story problem they had to share it with the class and the other students had to guess what strategy the group was using to trick the reader. We had many great ideas that we wrote in the board after the class guessed. We had too much information, addition problems that looked like subtraction problems, unfamiliar words, the numbers written in word form and different form like twice instead of two times as many. The students learned they need to use reading skills to understand math story problems. Thank you for the start to a great lesson.