Involving students as creators of problems for others to solve provides an experience for them that’s different from the teacher always being the source of problems. Also, when students have opportunities to solve their classmates’ problems, I find that they invest in their learning in a different way, often with added interest. And a benefit to teachers is that reviewing the problems that students create is useful for assessing their progress.
Here’s what I did with fifth graders studying fractions.
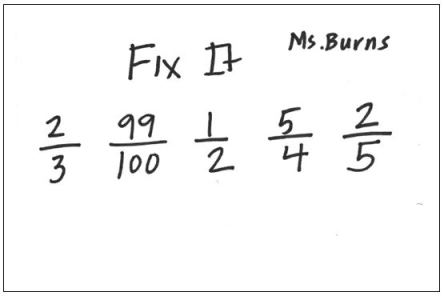
I wrote five fractions on the board, as shown above, purposely writing them out of order. I told the class, “Your job is to ‘fix’ what I wrote so that the fractions are in order from least to greatest.” Using think/pair/share, I asked students first to think on their own and then to confer with a partner. Next I called on students to share their ideas, each time asking them to explain their thinking. We repeated this for two other sets of fractions.
Then I explained to the students that they were going to create “Fix It” puzzle cards for their classmates to solve. I wrote directions on the board for what they needed to do before making their puzzle card. I explained as I wrote each direction.
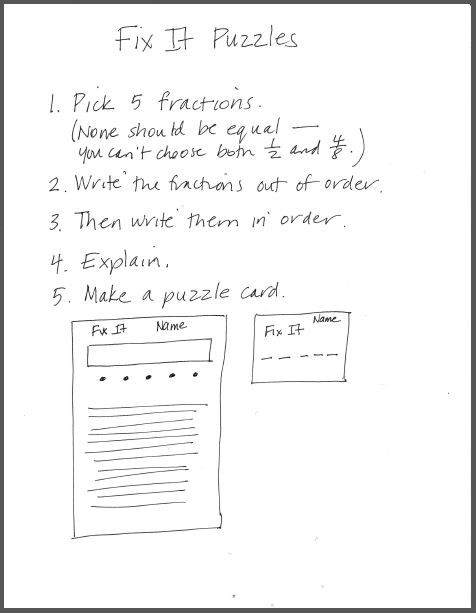
I modeled on the board how I would follow each direction. First I wrote my name and the title Fix It. I also wrote the number 1 after my name and explained, “That’s in case I decide to make another puzzle card.” Then I wrote five fractions purposely out of order—2/3, 99/100, 1/2, 5/4, 2/5. Underneath, I rewrote the fractions in order from least to greatest. As I thought out loud, I recorded below how I reasoned. (Later Jamie pointed out that I really didn’t write a sentence for 1/2.)

Finally I modeled making a puzzle card by copying onto a 4-by-6-inch index card the five fractions out of order from the top of my paper. I explained, “I put my name on the card so that anyone solving it can check the answer by looking at my Fix It explanations. After you’ve chosen your fractions, arranged them in order, and written your explanations, I’ll check your paper. Then I’ll give you a blank card so you can make a Fix It puzzle for others to solve.”
I gave one last direction. “When you solve someone else’s puzzle, you copy their fractions out of order, rearrange them underneath in order, and then write your explanations, just as you did for your own fractions.”
The students were interested in the project and began work eagerly. Meanwhile, I set up a folder for their papers so all of their solutions would be easily accessible.
As the class worked, I circulated and observed. When I approved students’ papers, I gave them an index card so they could make their puzzles. I asked those who finished first to exchange puzzle cards to solve, reminding them that they had to write their explanations.


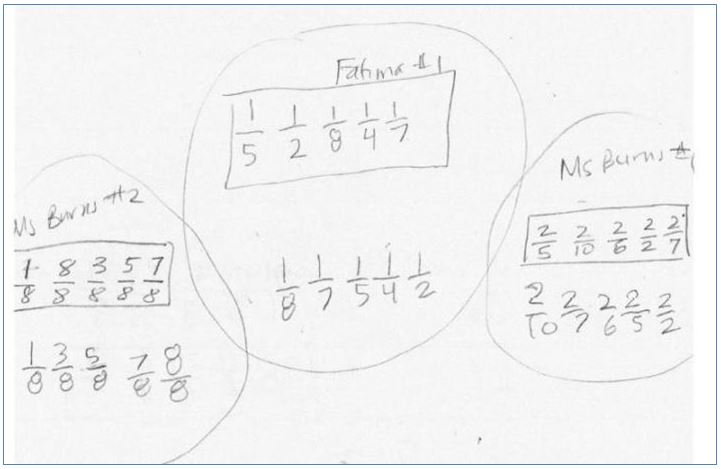
For a while as students were working, I sat with Fatima, a girl who had missed a good deal of school the year before and was struggling to keep up. She had written five fractions: 4/8, 1/3, 3/5, 3/6, 2/3. But she had no idea what to do next.
I suggested that she start with a different set of fractions. “Write five different fractions that all have a 1 on the top as the numerator.” Fatima drew a rectangle and in it wrote: 1/5, 1/2, 1/8, 1/4, 1/7.
“Can you put these fractions in order from least to greatest?” I asked. Fatima nodded that she could and reordered them correctly: 1/8, 1/7, 1/5, 1/4, 1/2. She explained, “The bigger number on the bottom means that the pieces are smaller.” I circled Fatima’s work and labeled it Fatima #1.
“Now I’ll write five fractions out of order and see if you can fix it,” I said. I drew a rectangle and in it wrote: 2/5, 2/10, 2/6, 2/2, 2/7. Fatima was able to reorder these correctly and explained, “It’s like the other one, but there are twos on top instead of ones. I circled this set and labeled it Ms. Burns #1.
“Want to try another?” I asked. Fatima agreed, feeling a bit more confident. For the next set, Ms. Burns #2, I wrote five fractions that all had the denominator of 8: 1/8, 8/8, 3/8, 5/8, 7/8. “I can do that,” Fatima said and reordered them correctly: 1/8, 3/8, 5/8, 7/8, 8/8.
My time with Fatima gave me information about what she understood so far. I relied on my long-time favorite—Fraction Kits—to help her move forward.
Solving “Fix It” puzzles became a choice on the math menu for fractions, and students solved each other’s puzzles over the next week.
Notes about This Post
I taught this lesson last spring in Sara Liebert’s fifth grade class in John Muir Elementary School in San Francisco. “Fix It” appears in the Do The Math intervention program, Fractions, Module B, Lesson 28. My colleague, Lynne Zolli, gave me the idea of having students make puzzle cards for the Fix It activity.


I appreciate so many things about this activity! There are multiple entry points and therefore so many assessment opportunities! I have not tried it with students. But I know I would have trouble in 4 areas: students waiting for me to okay their cards, students not understanding explanation cards to check their answer, and knowing when to abandon student beginnings in favor of stepping way back as you did with Fatima, and 4 orchestrating a whole class discussion about ordering.
I guess for the kid queue, I just need to have something else for students to work on while they wait.
If students have the same ordering but a different explanation, maybe they could add an alternative explanation sheet.
Thank you for the opportunity to think about Fix it.
Classroom management issues are always challenges. One option for Fixit is not to give OKs on that same day, but instead have students put their papers in the inbox for me to look at later. Then, after all students have completed their work, I can give them all cards at the same time to make puzzles. (Now why didn’t I think of that at the time?)Still, those students who finish more quickly need to be engaged in some other way. (That’s true for all class assignments, not just for Fixit.) That’s why I have an ongoing math menu of options. I’ve been meaning to blog about that. Math menus have made it possible for me to support students developing independent work skills, to differentiate experiences, to provide me time to work with students like Fatima. Hey, here’s a start of that blog. Stay tuned. And thanks for your comment.
Do kids learn fractions before percentages? Somehow, thinking back
long long ago when I was in grammar school, I recall turning fractions into percentages to see which was larger.
Some learn decimals first, some learn fractions first, some learn them both together. I go back and forth and have decided there’s no one “best” way.
I do a similar thing with 2 digit by 2 digit multiplication where students write their own question and do an answer key using one of the strategies. Then each day, three or four questions are given to the class to solve and the student who provided the question confirms the work of the other students. If the work is right, great, if not, the producer is responsible to work through the question with their classmate until they agree on the process and the answer. Even students who struggle are able to work through their own question and answer with support. There is no time limit for producing a question and answer. If the producer does not get the correct answer the first time, I ask questions until they find the problem spots and then we solve using another strategy so they are comfortable being the expert on that question. Students can submit questions when ever they want. I keep track of whose questions are being used and mix them up so all can be an expert. I like student generated questions because I find they are more engaged when they have initiated the problem.
As I was reading about Fatima I was wondering, did she have a fraction kit she could model with? Then, there it was at the end. Time and again modeling with fraction kits has proven to be an incredible sense-making tool for students.
I’m curious how the other 5th graders were making sense of the fractions in this activity. Was modeling prevalent or were they working abstractly? Perhaps a mix of both? If they were modeling, did any of them use a linear model to compare the fractions?
Thanks for sharing!
All of the students had access to their own fractions kits, and some liked to use the magnetic set on the board. Some students didn’t rely on the kits at this time in the year but were able to think about fractions abstractly; others needed the support of the pieces. We had worked with the students on a toolkit for comparing (from Do The Mathematics, Fractions, Module B) and they had been working with six different strategies for comparing fractions — comparing unit fractions, comparing fractions with common numerators, comparing fractions with common denominators, comparing fractions one unit fractions from 1 whole, comparing fractions to 1/2, and changing fractions to equivalent fractions. They justified their thinking about comparing two fractions with as many of the strategies as were useful for the particular fractions. With ordering, they’d start with two fractions, then insert a third fraction into the mix, then another, and so on until they had them all. There was a wide spread in the class, which is why I like this kind of activity that allows students to enter at a place that makes sense to them. And, yes, I couldn’t do it with the fraction kits. Thanks for your thoughts.
That sheds more light on the strategies you went to with Fatima. I agree wholeheartedly on the value of activities that are accessible to all students and that result in a range of strategies. Thanks for the extra context.
I’m glad the extra information was helpful. It’s hard for me to gauge how much to include in blog posts. I had actually thought about giving more context, but I worried it would be too long. I’ll be less heavy handed with editing myself next time. Maybe.
Isn’t that the truth? I see pros and cons to both more (longer posts) and less context (shorter posts). I’m usually worried about being misunderstood and so my writing skews towards the lengthy. I’ve noticed with some blogs, like Simon Gregg’s he gives you just enough and then his inquisitive readers usually ask good questions that pull out more context. I can tell you though that one thing I have enjoyed about your work (and other Math Solutions authors) is the vivid picture painted by the narratives that accompany the lessons.
Ultimately, I think it comes down to your reasons for blogging. My colleague Nina Sudnick decided her main reason was to blog as a reflective tool, which lends itself to expounding a bit more. Either way you go, I look forward to more of your insight.
Thanks again for your post. I’m a believer in William Zinsser’s view, whnich is something like writing is how you work your way into a subject and make it your own. With that, I hope to connect with others as we all work toward becoming better teachers. What a journey! Thanks for your interest and comments.