When Alfred, a sixth-grade teacher, explained the trouble-coming method of subtraction he learned as an elementary student, he recalled that his teacher had pointed out that you didn’t “make any cross-outs” or “write those little 1s.” He said that his teacher’s tone of voice made it sound as if doing subtraction without marks of any kind had to be superior. You can decide about that.
To describe the trouble-coming method for you, I made a short video (less than a minute and a half) to demonstrate with a sample problem. A warning first: You may find the video deadly dull. My husband, Jeffrey, listening to me record my explanation, wondered if perhaps I could use, well, a more conversational tone. I tried. Honestly, I tried.
Making the video reminded me of why teaching by telling can fall flat, especially when something calls for understanding and making sense. It also reminded me how difficult it is to teach the subtraction algorithm and to help students understand its logic. You may find my explanation confusing, but I think there’s something of value to discuss, both about the trouble-coming method of subtraction and about teaching a procedure (what to do) versus teaching for understanding (the why of what we do).
OK, enough disclaimers. I suggest that you take a look at the video. The sample problem is 6028 – 4352.
Discussion Questions
OK, now what? I think it would be helpful to talk about what I’ve shown with some colleagues. Here are questions that I’ve found useful for a discussion:
Question 1: How can you tell when trouble is coming?
Question 2: Does the method work for all subtraction problems? (Try it with some other problems.)
Question 3: Should we be teaching this method to students along with the standard algorithm?
Question 4: Why does the trouble-coming method work?
Some Responses to Discussion Questions
Question 1
You know that “trouble is coming” when you look at the column to the right and notice that the top number is less than the bottom number, making trouble for subtraction. (That is, if you stick to using only positive integers and zero, as we typically do when we first teach subtraction.)
Question 2
Question 2 is the easiest for me to answer. Yes, I’ve found that it works for all subtraction problems. A related question: Is it an effective and efficient algorithm? I think so. (I wrote about algorithms in my previous post, Another Way to Subtract.)
Question 3
I’m not interested in students becoming proficient with the trouble-coming algorithm. It seems to me that practicing with one algorithm to the point where it becomes automatic is enough. But I think showing the method, as I showed it to you, could lead to an interesting discussion with older students. It supports the ideas that algorithms are invented procedures that work and that there typically is more than one algorithm for a particular calculation.
Question 4
It’s been said that you know something best after you’ve had to teach it. That makes sense to me. To explain something, you first have to
make sense of it for yourself, and then think about how you might help someone else understand it. So to learn something—really learn it—you have to be able to explain it to yourself. For me, the trouble-coming method has to do with adjusting in a different way than we adjust when we regroup for subtraction, which we also do because we’ve run into trouble (with a digit in the top number that’s less than the digit underneath it). I’ll leave you with this challenge of explaining why this method works.
A Final Note: I wasn’t able to find anything when I did an Internet search for “trouble coming method for subtracting.” Nothing even remotely related came up. I searched a few other ways (e.g., “left to right subtraction”) but couldn’t find any reference to this method. But I did find something that reminded me of a method I learned a while ago for subtracting with only those dreaded zeros on top. See the second idea below in “Classroom Suggestions” for more on that.
Classroom Suggestions
Here are suggestions for engaging students with subtraction.
- If you choose to demonstrate the trouble-coming method with your class, do so with a light touch. Don’t make students feel that it’s a method they have to learn, but rather make the point that there are typically a variety of algorithms that work for any particular problem.
- Talk about subtraction problems with zeros in the top number. In my previous subtraction post, Another Way to Subtract, I wrote about Jesús’s method for solving 5000 – 328. Here’s another way to solve it. Since the zeros are typically what give students trouble, change the problem so there aren’t any zeros by subtracting 1 from the top number—that is, subtract 1 from 5000 to make it 4999. Then the subtraction will be easier. But since you reduced the top number by 1, you need to add it back; if you increase the answer by 1, you’ll be all set.
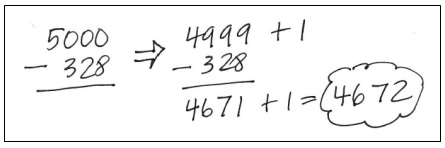
Have students in pairs try this method for other subtraction problems. While this works for problems when we subtract from a multiple of 10, it’s not effective and efficient for all subtraction problems. But it sure comes in handy for this one.
I was never formally taught the “trouble-coming” approach but it is what I find I use in eye-balling a subtraction calculation.
On the other hand, I have been trying to assist an older individual who was taught arithmetic very poorly, and very long time ago. I use the “Another Way to Subtract” approach and I could almost hear the click of the switch as the light bulb went on in this individual’s head! A delight for us both .
I’ve been told about that method but it included writing “those little ones.” This “trouble coming” stretches that working memory!
For 5000 – 328 I use a different method which “looks” to be very similar but conceptually is very diffrent. When I need to regroup to subtract the 8 ones, I take 1 ten from the 500 tens. Thus I cross out the 500 with a single horizontal stroke and write 499 above it. The one ten I write a 10 in the one’s column (i.e., exchanging the 1 ten for 10 ones).
Marilyn, this is mind blowing! I don’t think there’s any question but that it is superior in every respect. The main reason is that it gives results higher digits first so that you can stop at any point and have a good approximation (“it’s 16 and change”). As you showed you don’t need to use the little 1s or deal with more than one column ahead. Finally I think it’s ethically superior in that rather than making poor columns beg from the richer neighbors, the rich see that their brethren or sistren are in trouble and proactively help them. What really blows me away is how arithmetic could have evolved for hundreds of years without this method winning out.
Thanks.
Continually amazed that opening our minds to listen to our students explain their methods can only help us improve teaching others. Love “troubles coming” expression. Thank you.
One more thing I just realized. This algorithm is the “natural inverse” of the usual addition algorithm. Here’s what I mean. If you go from one state to another by a sequence of steps A then B then C then the natural way to undo that sequence of steps is “undo C” then “undo B” then “undo A”. For example if you put a present in a box and then wrap the box with paper then the natural way to get the present back is to unwrap the box and then take the present out of the box. The usual addition algorithm computes the sum by adding digits right to left. So the natural inverse (subtraction) should compute the digits left to right. In adding you obtained the top number by going through the columns right to left and adding some digit to the bottom digit to get the top digit. For each column, working right to left, we have to figure out what that digit was. If the top digit is bigger than the bottom digit then it’s easy, we just subtract the digits. But if the top digit is smaller than the bottom digit we must have “carried” and so to undo the operation we must undo the carry, i.e. borrow and then subtract.
I just keep wondering why that other right to left subtraction became the standard!
Thanks for pointing this out — I hadn’t thought about how this “trouble-coming” algorithm relates to the addition algorithm. And I have no idea how the algorithm typically taught today became the standard. It wasn’t the way I was taught when I attended PS 225 in Brooklyn as a child. I learned a right-to-left method that involved adding the same amount to the two numbers to keep the difference the same. I later learned that it is sometimes called the “equal addition method of subtraction.” I still subtract that way when I resort to paper & pencil. Old habits are hard to break.
Makes sense to me.
I shared this with our teachers and one of my colleagues (first grade teacher) was so fascinated that she kept trying numbers til she found one that stumped us.
9521 – 7585
Not sure what to do with 5-5 even though trouble is coming?
For 5-5, since there’s trouble coming, it seems that you have to change the zero to 9. Ah, me, another rule to learn!
Didn’t read this whole blog post, because I don’t care for Flash video (for security reasons I don’t run Flash). So, without the example video I didn’t follow the blog post. But, the comments are enlightening.
Without having a handle on this so called “trouble coming” I have to agree with Dave’s post of “natural inverse”… So, if I get to Marlo’s math problem of 9521 – 7585 in my head, the first two digits left to right easily break down to 20, but 21-85 doesn’t easily break down, so in my head I simply subtract 1 from 20, 19(00) and continue on 121-85, in which case my brain switches to the “natural inverse” of subtraction, which is addition (and all of this is an example of what is called “algebraic reasoning”). 85+15+21 = 121; 15+21 = (19)36. Hence 9521 – 7585 = 1936.
Funny, it takes me a long time to even do it in my head when I am trying to purposely explain the reasoning of what my logic circuits (brain) are doing in the background; but it is accurate nonetheless. It’s also why I got in trouble over and over in math class for not “showing my work” (I didn’t understand HOW to show the work that goes on in my brain until I was an adult and was able to put “arithmetic” and “algebra” together into one thing called “mathematics.” Or, as I posted in another comment on here, “thinking mathematically.”)
To answer the most obvious questions:
Why did I pick 85+15 and then add another 21? Just to make the place values quick on the one hundred, it’s quicker for me to recognize a 100-85 = 15, and 121-100 = 21 simply in my brain (again instantly crossing addition and subtraction, could have typed it 85+15 = 100 and 100+21 = 121). Or put it another way, to subtract 85 from 121, I figure in my mind the sum of the parts above 100 and below 100.
Two things to note with the way my brain comes up with the numbers
1) Note that there is ZERO difference between addition and subtraction, they are identical but inverse of each other. In fact, in digital electronics class I took in high school, I had to learn how digital computers make computations… And they NEVER subtract. They only inversely add. (They never multiply, rather they add multiple times, and they never divide, rather inversely add multiple times)…. [well these days digital circuits most likely include math tables, for more rapid calculations, but that is beside the point]
2) The great thing about place values, is that really it doesn’t matter where in the number sequence they are, each digit still represents values of 10 (0-9). So, subtracting 95123456 from 93123456 is just as easy as 95-93, 12-12, 34-34, 56-56, or 951-931, 234-234, 56-56 (how ever many digits your mind can quickly assess. Double digits for me is usually the easiest, though some numbers triple digits go fast in my head).
In fact, I would prefer teachers to not focus at all on “ones” “tens” “hundreds” “thousands” (other than for the purpose of naming or reading numbers aloud), but when teaching arithmetic focus only on “digits” or numbers 0 to 9. It is the only complaint I have with the Common Core Standards is that certain grade levels are still directed to be able to do calculations on a certain number of digits. But, then again, I am no longer a child, so I don’t remember if having too many digits becomes bogged down in the overwhelming factor, despite it all being the same thing over and over.
One additional comment on subtraction = inverse addition
I often don’t do calculations on paper either, most often, if it is going to take multiple steps, I use a calculator and my head. But, understanding that addition and subtraction (inverse) and negative and positive (inverse) numbers are all the same; even when a calculation I need to perform on a calculator may require addition or subtraction, and multiple steps where the steps themselves may not be apparent until I am in middle of the calculation, hence in my head I may rapidly switch between negative and positive, addition and subtraction where applicable.
For instance, I quite commonly find that I have a negative number showing on the calculator when I knew I needed a positive number (well its all the same). I wish I could think of a real world example off the top of my head.
However, I believe I can explain it without a real world problem. Perhaps I have many steps on a calculator to arrive at the number 150 which I then store in the calculator’s memory while I begin to perform other calculations necessary to arrive at another number. When I get done with those I have a number showing on the calculator as 100.
I may know in my head that the final result needs to be positive; that I need to subtract the 100 from the 150 (which may not have been apparent when I started the first set of calculations), but it is the 150 that is in the memory of the calculator, and the 100 that is the current number. I don’t want to have to remember the 100, and start over with the memory number; yet hitting “add” wouldn’t result in the proper calculation. Still hitting “subtract” will perform the opposite calculation needed. But, understanding that subtracting either of the two numbers from the other is the same result (only inversive).
Some calculators even have the “invert number” (or +/-) key on them already otherwise I either retype the number if more calculations are needed, or remember I am on the other side of the number line, or just use the positive version of what is showing if that was the final calculation.
In other words calculating 150 – 100 = x is the same as calculating 100 – 150 = inverse (or negative or -) x.
Speaking of subtracting a larger number and arriving an a negative number:
One of the things that bothered me in school was when I was told by a teacher that you can’t subtract a larger number from a smaller number. I had older siblings who told me that wasn’t true, and yet, it wasn’t until I was an adult that I realized it was “beyond the scope” of that math class, and hence why the teacher didn’t want to bother teaching it.
It could and should have been taught, because, it is important for children to recognize the inverse properties of mathematics including negative vs. positive, addition vs. subtraction; multiplication vs. division and how each of those are one in the same thing.
One more example to drive home the point why this is an important concept for children to understand:
It wasn’t that long ago my boss (an educator) explained to me she thought, for instance, that if a child sees the equation 1 + 5 = 6, should instantly be able to reason that 6 – 5 = 1 or 6 – 1 = 5. I agreed wholeheartedly, and typed up how that works with the Common Core’s institution of algebraic reasoning starting even in Kindergarten.
Why does it work? Because addition and subtraction are the same thing, only inverse, thus we change the symbol (+ to -) by changing (inverting) the order of items to be equaled (=). Hence 1 + x = 6, we easily determine the x represents the number 5, because in our mind we can reason that the equation is the exact same as calculating 6 – 1, hence x = 5.
Yes, even kindergartners can “do algebra” if they are taught to think that way. But tell a child, who already reasons in their mind that way, to “show your work” and you will get frustration every time.
When subtracting 5000-328, the difference between the two numbers is the same as the difference between 4999 and 327. It would look like this:
5000 (4999 + 1)
-328 -(327 + 1)
4672 4672 + 0
This is a horrible way to teach subtraction. The students may be able to memorize this and do it but, most will have no idea what they are really doing or how it works. It is no better than the standard algorithm and students cannot go on to do higher math it they don’t under stand the concept.
Thanks for your comment. I agree completely that it would be unfortunate and ridiculous to make this our teaching method for subtraction. My worry is that the “standard” algorithm that’s generally taught is just as unfortunate and ridiculous for students to learn. At least, for some students. My goal is for students to understand that subtraction is the inverse of addition, that they can subtract mentally when numbers are reasonable, and that they can estimate when answers don’t make sense (I was amazed a few weeks ago when a student defended the answer to 1000 – 998 as 112), and that they can use paper and pencil to keep track of their thinking. Algorithms are useful mathematical procedures, but without understanding and number sense, I worry when they are emphasized as the real way to do math.