Multiplication Bingo has long been one of my favorite games, and I recently introduced it to students who have been committing the multiplication facts to memory. (More about how the students are studying the multiplication facts appears at the end of the blog.) Also, several different versions of the game allow for varying and differentiating the experience, making it a good addition for the class Math Menu.
First a Caveat about Understanding vs Memorization
Years ago I had a conversation with Paul, a fourth grader. His class was studying multiplication and Paul’s teacher was concerned about him. She told me that he typically worked very slowly in math and “didn’t get much done.” I agreed to talk with Paul and see what I could learn. Here’s how our conversation began:

When I probed Paul’s thinking, I learned that he saw each multiplication fact as a separate piece of information to memorize. He lacked understanding of the meaning of multiplication and he wasn’t able to reason to figure out the answer to 6 x 9. Playing Multiplication Bingo or other similar games wasn’t going to serve Paul’s need. Games won’t do harm, I don’t think, but building and strengthening understanding is what’s essential for multiplication instruction. With that caveat, below I describe multiplication games that I’ve found to be useful to support learning of the multiplication facts―when appropriate.
Introducing Multiplication Bingo: Version 1
After talking with the students about playing Bingo, I told them I had a math version for them to play, Multiplication Bingo. I drew a 5-by-5 grid on the board and wrote FREE in the middle box.

I showed the class two 1–6 number cubes and explained, “I’m going to roll these two cubes and we’ll multiply the two numbers that come up. If you have that product on your Multiplication Bingo card, you can cross it out. If the number appears more than once on your card, you can only cross out one of them. The winner is the first person to cross out five in a row.”
Then I said, “I think that 1 is the smallest product that can come up from rolling the two cubes. Who can explain why?” Hands shot up and a student explained that 1 was the smallest number on the cubes and 1 times 1 was 1.
I asked them to talk with their partner about what the greatest possible product would be. After a moment, I had them say the product out loud. There was a chorus of 36s and I asked a student to explain. Then I listed the numbers from 1 to 36 on a sheet of chart paper.

I distributed a worksheet to each student that had four 5-by-5 grids on it, each with FREE written in the middle box.
“Ready to play?” I asked. Several students pointed out that there weren’t any numbers on their cards.
“Not yet,” I said, “but that’s your job. You can fill in the first card with any numbers you’d like from 1 to 36. It’s OK to write a number more than once, but you have to write a number in each box except for the middle free box.”
As is typical whenever I introduce something new and students aren’t sure what they are to do, confusion erupted. I reassured  the students that this was just a first trial game, that we’d play three more games afterwards. I coaxed them into writing numbers just to experience the game. I urged them to fill in their boards more quickly so we could play. Finally, everyone had written numbers. Here’s the first game board from one of the students.
the students that this was just a first trial game, that we’d play three more games afterwards. I coaxed them into writing numbers just to experience the game. I urged them to fill in their boards more quickly so we could play. Finally, everyone had written numbers. Here’s the first game board from one of the students.
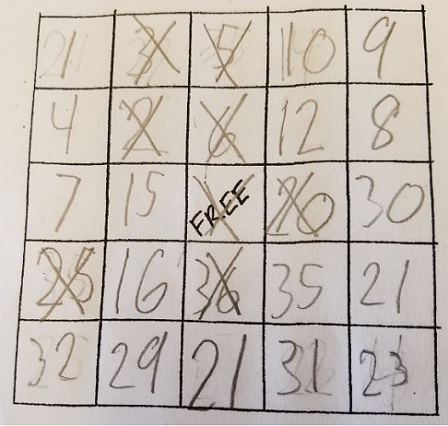
All of the products were in the 1–36 range but, as is typical when students first learn to play, some numbers weren’t possible products from rolling the 1–6 cubes―21, 23, 31, and 32.
To play, I went around the room, giving a different student each turn the two dice to roll and call out the numbers. Then we said the product together. And then students looked to see if they could cross out a number on their card. The room got quiet as they examined their cards. I reminded them that they could only cross out one number for each roll. Also, I drew a tally mark next to the product on the list of numbers from 1 to 36.
After 13 rolls, one student shouted, “Bingo!” I had her read the products she had crossed out to be sure they were products I had marked on the board. The products that came up during out first game were 2, 3, 5, 5, 5, 6, 15, 18, 18, 18, 18, 25, and 36. (They didn’t come up in this order.)
The students were eager to play again. I offered some advice, “You may want to change the numbers you write on your card for the next game.” Some did , some didn’t. I resisted the temptation to talk with the class at this point about which of the numbers between 1 and 36 were possible products . I also resisted the temptation to talk about some products being more likely than others. I’ve learned that giving students time just to play is important and valuable, and it also gives them time to begin to wonder about questions like these, and more.
, some didn’t. I resisted the temptation to talk with the class at this point about which of the numbers between 1 and 36 were possible products . I also resisted the temptation to talk about some products being more likely than others. I’ve learned that giving students time just to play is important and valuable, and it also gives them time to begin to wonder about questions like these, and more.
We played a second game, and I marked each product that came up on the 1–36 chart using a different color marker, light blue this time. This time, it took only 5 rolls for someone to get Bingo: 3, 12, 25, 25, 30. That’s not typical in my experience. But, hey, I don’t control the rolls, which is one aspect of the game that I like.
Then, before we played again, I asked them what they had learned about numbers to write on their cards, and if they had any strategy for filling in their cards. I had them look at the data I had marked on the on the 1–36 chart, then talk in pairs, and then report their ideas. They realized that 7, 11, and 13 couldn’t come up, providing me a way for me to talk later with them about prime numbers.
There was less confusion as they prepared for the third game and some students were beginning to think more strategically.
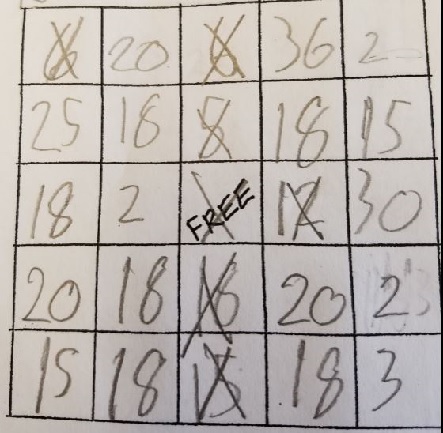
Here’s the third Multiplication Card for the student whose first card is shown above. She made more informed choices this time.
I captured the data on the chart, introducing the terminology of Frequency Distribution to describe it.
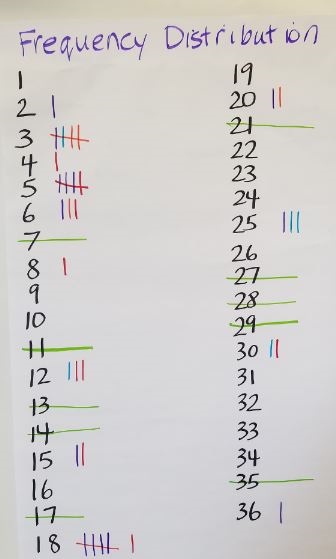
After recording more rolls, the chart gives more useful information.
At the end of class, students wrote reflections as they do each day.


As the students played on subsequent days, their strategies improved. Also, I left the frequency distribution posted for their reference and for us to add to and discuss again.
Baby Bingo Blackout: Version 2
This version is played on a smaller grid, 3 by 3, with the goal of crossing out all nine numbers. There’s no “free” space. The first player to mark all nine products wins.

Other Versions of Multiplication Bingo
Changing the number cubes gives students new ways to experience both games. Using a 1–6 cube and a 4–9 cube changes the products that students can enter, with the range of products of 4 to 54. Using 1–10 spinners provides another variation, now with the range of products from 1 to 100. Any change in the number cubes or spinners calls for again analyzing the products that are possible, and that provides an opportunity to think more about probability.
Communicating with Families
For a writing assignment linked to the math experience, ask students to write about the game so that they can teach someone at home how to play. Several years ago I gave this assignment to a class with three prompts for what to include:
- What do you need to play?
- What are the rules for playing?
- What strategies did you use?
Here are samples from three students after they had learned Multiplication Bingo and Baby Bingo Blackout and played the games on and off for about two weeks.

Emma explained her strategy based on products that were possible.

While Sam’s math thinking shows understanding, his spelling is atrocious. Out of 100 words in his paper, about 20 were misspelled. His struggle with writing didn’t interfere with his enthusiasm for the math.


Kelly included examples of filled-in Bingo boards that she felt were successful.
About Learning the Multiplication Facts
Years ago, a boy in my class said to me at the end of the day, “I hope tomorrow that I know my times tables.” I asked him what he was planning to do that night to help him learn them. He looked at me blankly―he had no ideas for how to study.
The next day, I initiated a discussion with the class about different ways that they could learn the times tables. Students had various ideas: write them down, use flash cards, say them out loud, use a computer game. I wrote all of their ideas on the board.
Then I asked them if they’d rather study alone or with a friend. Again, they had different ideas: some thought they’d rather study alone, some thought with a friend, some thought studying along and then have a friend test you. I added these ideas to the board.
Then I asked them each to choose a way that they’d like to try to learn the facts for multiplying by 4. I gave them time to choose and make whatever materials they needed, and I gave them time to try the methods they chose. I told them that when they thought they were ready, to let me know, and I’d test them. (I planned to do this by asking them three of the facts: 4 x 3, 4 x 7, 4 x 8. If they got those correct, I was satisfied.) My scheme was to give them the responsibility for learning and also the freedom to learn how. After the 4s, we repeated for the 6s, and they were free to use the same method or choose another. It worked for this class, and has been successful with others.


I love this idea! I’m going to try it out with my older groups this week and can already envision the “a-ha” moments around deciding which numbers work best. Thanks!
I really like this approach to multiplication bingo. It gives students more of a say by allowing them to place the products on the bingo card. Lots of opportunity for discussion, reasoning, and strategic thinking! Thanks for another helpful blog entry Marilyn! These entries are so accessible and thought provoking. There’s an interesting article by Mark Alcorn called “Reconciling Memorization of Basic Multiplication Facts with Teaching Efficient Strategies.” It provides some great ideas about helping students use the relationship between the facts in order to deepen understanding of multiplication (e.g. 7×6 is the same as 7 x 5 plus 7 more). The article is in the June 2008 issue of the California Mathematics Council Communicator.
Thanks for this Marilyn. I will try this out with my Grade 4 students in my after school maths clubs, as we have been doing a lot of work with understanding multiplication. I like the fact that the students can choose numbers and the process that may help them understand why some numbers work and others don’t.
“As is typical whenever I introduce something new and students aren’t sure what they are to do, confusion erupted.” This statement alone made this fantastic post worth reading, because this seems the “modis operandi” for students in my 4th grade math class! Thanks for the great ideas.
I used this lesson to teach some teachers about how you can take a simple lesson that covers 2 of the 8MP, make a little change and now it covers all 8 of the 8 mathematical practices. I also found that getting students to come up with the numbers to fill the squares is less work for the teacher and thus making it more effective and more efficient.
Thanks for this great and engaging lesson idea. I really like the way that you have incorporated a discussion in the lesson as a way to develop the students’ understanding of multiplication and ensure that students aren’t just rote learning answers. I also think the variations you have incorporated are a great way to differentiate the lesson to ensure that it caters fort he needs of all students.
Thanks for this innovative way to teach multiplication. I love the way the students have control over their learning. My son is great with problem solving but struggles with number facts, so I will definitely use a version of this idea at home; it’s bound to improve our dreaded multiplication homework battles!
I have played multiplication bingo with my students for years, but I always have had pre-created cards. I like the idea of them filling in their own cards and making the connection of which products will come up more often due to probability. Also, will probably try factor bingo. They can write a bunch of different factors. When I say the product, if they have one of the factors they can cross it off. Worth a shot.
I tried using this with my 3rd and 5th graders at home during our summer break. My girls love it. They like filling in the numbers and I enjoy watching their strategies. We played for about 2 hours because they were hooked on it. It is a fun way to practice their math facts. We also changed it up by playing a few rounds of multiplication, then addition, and subtraction. For each operation they use different strategies to fill in their numbers. Thank you for a great idea.
Thanks for the feedback. Puts a smile on my face.
Yessss! Thank you Marilyn! This is the perfect lesson for my students! We already play Multiplication BINGO, but most aren’t learning anything from it. I love this approach, and never would have thought of this.
Any way you could share your board templates with me?
I created two blank bingo templates, Multiplication Bingo and Baby Blackout Bingo. Sorry I don’t have them available — they were just rough sheets that I created fairly easily. See the templates in the blog post. Hope this helps.
he’s a smart kid! i definitely can imagine how his life will be in the future. btw, very informative blog. thank you for sharing these tips also!