Instruction about angles and how to measure them typically begins in fourth grade and continues in middle and high school. This blog describes the instruction about angles that Sara Liebert and I provided her fourth graders at John Muir Elementary School in San Francisco. We planned together, basing the lessons on ones I had taught before but usually to older students. We were thrilled that the experiences worked fine with the fourth graders. This mini-unit is a keeper.
To tell the story, following is a series of photos from the first three days of instruction, each annotated with information to describe what we did and what resulted. Information follows about the instruction that followed.
You’ll notice that the introduction to the unit was a number talk that focused on factors of the number 36. Sara regularly uses number talks with the class, and while this doesn’t seem to relate directly to learning about angles, please be patient as you read and you’ll find out about why we began this way.

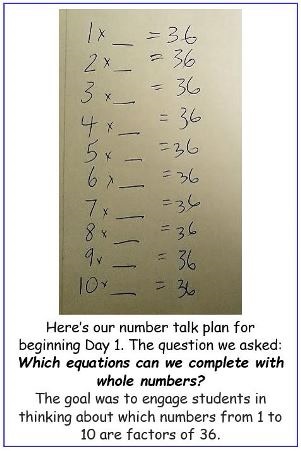















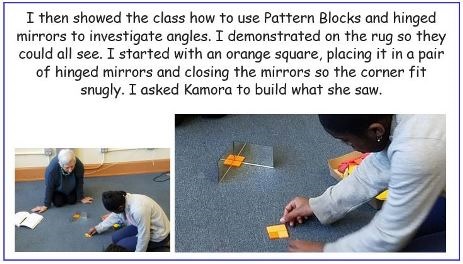
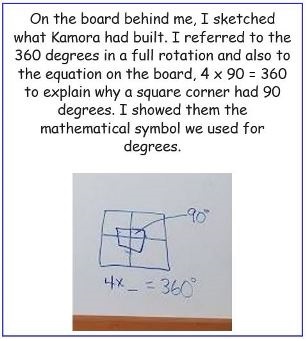





Reinforcing Angle Vocabulary
New vocabulary is best learned in the context of experiences. After the students had explored measuring the Pattern Block angles with hinged mirrors, we introduced the terminology of right, acute, obtuse, and straight angles. We wrote these on a Math Words chart and used the terminology regularly with the students.
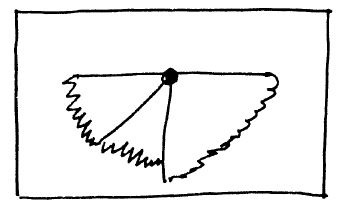
Constructing Weird Angles
Instruction continued over the next days with a variety of explorations. Students used what they knew about the angles of the Pattern Blocks to construct “weird angles,” combining angles from Pattern Blocks and paper folding to make new ones.

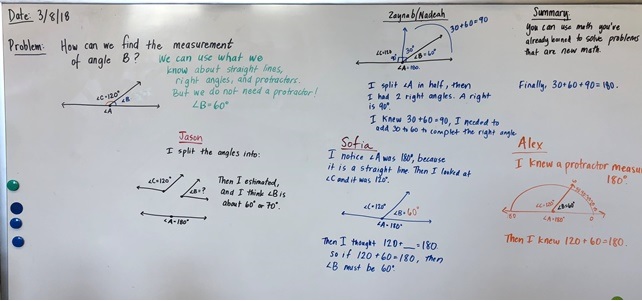
Investigating the Sum of the Angles in Triangles
From the investigation they had done with the Pattern Blocks, they were able to figure out that the sum of the three angles of the green triangle was 180 degrees. We engaged them in an investigation of other triangles, drawing different shape triangles, cutting them out, tearing off the corners, and arranging them touching the same point. The students were amazed that the angles of all triangles always made a straight line, or a straight angle.
Using Protractors
The students learned how to use protractors to measure angles. Sara showed the class a protractor and told the students that it was a measuring tool. She reminded them that they use rulers to measure lengths and scales to find out how much things weigh, and now they’ll explore how to use protractors to find out how many degrees there are in different size angles. She distributed protractors for students to examine. After a bit, she asked them to describe what they noticed. They reported a variety of observations: “It’s round.” “It’s half a circle.” “It has a hole.” “There are two rows of numbers.” “The number 90 is in the middle.” Sara showed them how to use the protractors, starting with a right angle which they already knew had 90 degrees. The students were intent on figuring out how to use the protractors to measure other angles.

Incorporating Angles into Number Talks
Sara continued to engage the class with number talks that presented angle measurement problems. Here’s the board work from one of these.
To prepare, Sara and I relied on Chapter 6 from A Collection of Math Lessons from Grades 6 Through 8, a book that Cathy Humphreys and I wrote in 1990. That’s the book by my side in the introductory photo of the blog with me on the floor with Pattern Blocks and hinged mirrors. An updated version appears in my 2020 book, Welcome to Math Class.



Brilliant and beautiful! Thank you for sharing 🙂 –Ana
Ever so refreshing to read through this hands-on mathematics/science/literacy lesson. To provide a thorough understanding of angles with manipulatives, writings that the children/teacher can hold/see/work with seems to provide a more in-depth understanding than simply scrolling around on a computer. Thank you for continuing your work, particularly with how many children learn best!
I am on the look out for a children’s book that talks about the origin of the 360 degrees rotational starting point, and, will certainly let you all know if/when I locate one.
Thanks for your comment. I hope you find a children’s book — I’d much appreciate.
Hi Marilyn,
Do you have any advice on getting my math story published? I have an original idea for a hilarious math story. I’m reaching out to you because I’ve used your stories to reinforce or introduce math concepts. I think using literature in math class is so important in reaching the creative learner. I’d love to hear any advice you may have.
Thanks
My email is laurakruger35@hotmail.com
Or feel free to call 7135623800
I haven’t written a children’s book in a while, but I suggest looking at publishers who have already published books that are similar and contact the children’s editor. They’re always looking for good books to support learning. Good Luck!
I enjoyed this Marilyn!
I like the way you set up the connected idea of factors of 36 and then factors of 360 that would later provide an aha! moment! I also like the way of using the mirrors to start from 360° when thinking about angles.
I approached it a differently – but again with pattern blocks – throwing my Gr 3s in at the deep end. Their starting point when the breakthrough came was that squares have a 90° angle, and then that three of the thin rhombuses’ acute angles fit into the square’s 90°.
http://followinglearning.blogspot.com/2018/11/hexagons.html
(There was a funny, and instructive, thing that happened later. I was asking them to estimate a slice of pie that was eaten. It was 72°, but students were estimating 90°. I couldn’t help blurting out that it was clearly not 90°! They then went to 60°. Then I realised, they were generalising from the pattern block angles, that are in multiples of 30°!)
Thanks for the comment and for the link to your blog. You’ve added to my file of what to do with pattern blocks and angles. So much.
My fourth-grade daughter was so excited about the “exploration” piece of this lesson that she asked me to email it to her teacher!!
C Rollins
1st grade teacher
I’m a retired teacher and not able to do replacement teaching (I’m temporarily living in a country where I don’t have a work visa), however, I wish I could still teach and love to read your blogs. Thanks for this one! What lovely investigations!
This is fantastic, Marilyn, both how you structured the investigation and its presentation in pictures and words. Easy to absorb and inspiring. Makes me wish I had a class of my own to explore with.
Vicki
I really love how you combined multiplication, division, hands on play, shape review and angle measurement into an engaging series of lessons. I think this will be perfect to do with my 3/4 combination class as the year comes to a close. Thanks!
I am a Mother of a boy who is in elementary school . I love to follow your blog, books and ideas to teach him Maths at home. You make it so simple yet interesting. Always conceptually clear for children. Thank you so much.
Where can you purchase affordable hinged mirrors?
I suggest searching online for “hinged mirrors math games.”