A Children’s Book, Differentiating Math Instruction, and More

One of my favorite books for supporting math lessons is Out for the Count: A Counting Adventure, written by Kathryn Cave and illustrated by Chris Riddell. I’ve read this book to classes from first through fifth grades and have found that students in all grades are always interested in the story and the illustrations. I’ve used the book in different ways, depending on the class, and here I describe several of my teaching experiences.

About the Story
The story is about a boy named Tom who sometimes finds it hard to get to sleep. One night, after three drinks of water from his dad and six hugs and four good-nights from his mom, he still can’t fall asleep. At 10 pm, his mother tells him that she’s going off to bed and advises that he try counting sheep. Snuggled into bed with his ten stuffed animals, Tom gives it a try.

The story then goes on to describe a wild night of dreams full of adventure . . . and full of math. For example, Tom finds himself deep in the woods terrified by a pack of sharp-toothed wolves on the prowl.

From the illustration, it’s hard to tell how many wolves are in the pack, but the illustration on the next page helps by organizing the wolves into a group of 10 to make counting them easier. There’s one group of 10 and two extras, 12 wolves in all, an introductory place-value experience.

After Tom comes down from the tree he had climbed to avoid the wolves, his nighttime adventure continues. Later on he finds himself in the midst of penguins.

Like the illustration of the wolves, it’s hard to tell how many penguins there are until they’re grouped into 10s. Then the students can count more easily—10, 20, 30, 40, 50, and four extras make 54. Again, place value to the rescue.

As the adventures progress, the numbers increase. Later on, Tom stops at a beach, hoping to relax and sunbathe. Hah! After seeing all those bears, Tom decides that it’s better to drive away. How many bears? Again, grouping by 10s reveals 61 bears—six 10s and one extra.

What I love about the book are the intricate illustrations, the rhyming text, the sense of adventure, and the marvelous way that our place-value system of numbers emerges. It’s a wonderful read-aloud that’s perfect for repeat visits.

Using the Book for a Math Lesson
Now to a math lesson built on the book. Here’s a price list for stuffed animals. These animals are the same stuffed animals from Out for the Count. And the prices? They provide practice with adding within 100. Students choose any two of the stuffed animals to buy and figure out how much they would cost together.

This lesson is included in Do The Math, in Addition and Subtraction Module A. Earlier in the module, the students learn several different ways to add—writing equations, applying an algorithm, and using open number lines. Now, after enjoying the story, students practice these methods, deciding which animals to buy and figuring out how much they cost.

In Do The Math, we structured the Workspace page for students to record, providing a page that’s more of an organizer than a worksheet.

One Class. Two Students. Different Approaches
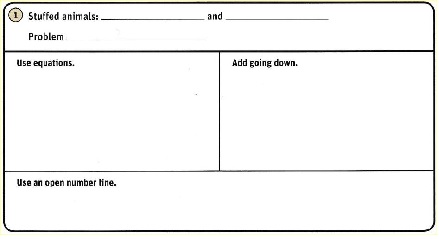
Will, a second grader, chose to figure out how much it would cost to buy a ghost, which costs $26 on the price list, and a bear, which costs $35. Will demonstrated the three ways we had taught and arrived at the same answer of $61 each time.

There are several interesting things to note. First, with all three ways, Will began by adding the 10s first and then added the 1s, different from the algorithm of adding the 1s first. (We find it very common for students to add the 10s first when they reason mentally to figure out sums.) When stacking the numbers to add them, he switched the ones digits in the two numbers, changing the problem from 35 + 26 to 25 + 36, and the answers were the same. (We don’t know if this was intentional, but it opens the door for investigating if and when this sort of switcharoo would always work.) When using the open number line, Will again solved 36 + 25, this time by adding 36 + 30 to get 66 and then subtracting 5.

My takeaway: What I love about this assignment is the choice it allows and the flexibility it supports. Yes, there’s a right answer for the problem the student selects, but there are different ways to arrive at the answer. I think it’s important to help students learn to reason numerically, not in one particular way, but in ways that make sense to them and are appropriate for the numbers at hand.

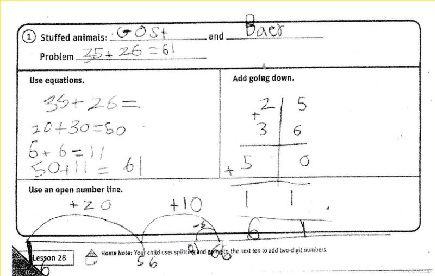
Here’s a solution from Andrew, another student in the same class. The students were asked to repeat the task three times, choosing different animals for each. For his third try, Andrew asked me if it was all right if he bought four stuffed animals, and I agreed. He chose a tiger, a penguin, a ghost, and a goat. When Andrew brought his paper to show me, he was grinning, pleased to show me how he made the answer end in a zero. Same class, same assignment, different problems, different approaches.

My takeaway: Correct answers are only the beginning. How students reason is the end goal. In this assignment, students weren’t expected to repeat one particular procedure they were taught, but instead to use the tools they had learned to reason. I believe this is an ideal way to support students’ math learning.

Differentiating the Assignment
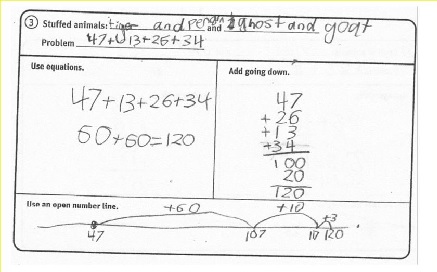
Assignments often need tweaking, even when they provide a variety of entry points and solution strategies, like this one. While Will and Andrew were successful, I knew that wouldn’t be the case for everyone in the class. For some of the students, the numbers on the price list would be just too hard. To support students for whom this assignment wasn’t accessible, I repeated it the next day, offering an alternative price list.

“The store is having a special sale,” I told the class. “If you’d like, use these prices instead.” Not only did I offer an alternative price chart, I told students that could buy eiether two animals or more than two. Also, I presented a challenge option of figuring out how much it would cost to buy one of each stuffed animal, using either price chart. Along with their calculations, what students choose to do gives me information about their confidence and interest.

My takeaway: Differentiating instruction so that experiences are accessible to all students while offering a challenge option is important.

Emphasizing Mental Math
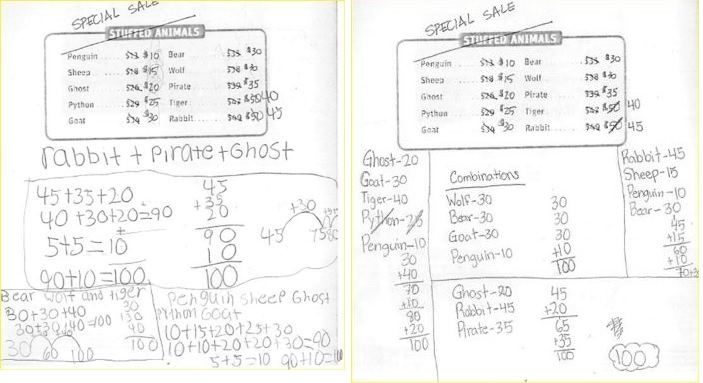
I think it’s important to encourage students to reason mentally, not only to rely on paper-and-pencil procedures. For a fourth-grade class, I changed the original price list to sale prices, but this time chose all prices that were multiples of 5. Students were to choose two stuffed animals to purchase, first figure the sums in their heads, then verify them with paper and pencil in the same three ways.

For a challenge, I told students that they might be interested in figuring out how to spend exactly $100. When I explained these options, one student noticed two prices that didn’t make sense for a sale. ‘You made the tiger and rabbit more expensive,” she announced. I realized that I had made an error and asked the class what I should do. After a short discussion, the students agreed on making the last two prices $40 and $45, as you see on the students’ papers below. Involving them in this decision was another way to give the students agency. One paper shows four solutions to the challenge problem, and the other shows three solutions.

Another Challenge
When I was reviewing these students’ papers, I thought some more about the original price list and wondered if it was possible to use the original prices and spend exactly $100. I fiddled with the problem a bit but didn’t come up with a solution. The next day, I offered it as another option. I told the students that I hadn’t figured out a solution yet and wasn’t sure that there even was a solution.

I included the option on the Math Menu. Not all students were interested in this problem. The beauty of using Math Menus or the Math Workshop model is that students can make choices about the work they do.

Some took on the $100 challenge and here are two examples of student work.

A Final Thought
These lesson ideas model several instructional practices that I think support students’ math learning:

• incorporating a children’s book into math instruction,
• adjusting a lesson to be appropriate in different grades,
• differentiating instruction within a class,
• giving students agency over their work,
• providing practice in several ways.

I strive to incorporate these qualities into all lessons, so that all students have access to the experiences, are motivated to engage, and can be successful.

Another Experience with Will’s Switcharoo Strategy
This Listening to Learn video clip of me asking Tova during an interview to mentally add 36 + 9 was the second time I’ve experienced a student switching the digits in the ones place when adding. This makes me think it would be interesting to create a lesson to engage students in thinking about when it’s OK and when it’s not to switch digits. What about when subtracting? Multiplying? Dividing?

 

Visit Do The Math for more information about this supplemental program.

Visit Listening to Learn for more information about math interviews.