A friend who is a house painter told me that he spends most of the time getting ready before painting―cleaning, sanding, taping, spackling, priming, etc. If you do all the preparation with care, he explained, then the actual painting goes smoothly.
I think the same is true for teaching.
For many years, I’ve written vignettes that describe how lessons I’ve taught have unfolded in classrooms. My goal has been to bring lessons to life so others could try them. But the vignettes typically haven’t revealed all that I had to do before teaching the lesson. I’ve begun to think recently that it might also be beneficial, or perhaps even more useful, to write about what I do when getting ready to teach.
This is my first attempt at writing from this perspective.
Preparing and Planning―Both Essential Before a Lesson
The thinking I do when teaching something new falls into two categories: preparing and planning. I have to prepare for dealing with the math content―what math goals I have in mind, why this a good time for this specific lesson, how I might engage the students in thinking about the math, what I need to do to give students access to the experience, how I’ll encourage them to think about the mathematics involved, and more.
Then I have to plan for dealing with logistical issues―where in the classroom I’ll gather the students, how I’ll organize the students if they are to do partner or group work, how I’ll manage transitions during the lesson, what individual work I might want students to do, and more.
These issues are essential for lessons to go smoothly.
A Lesson about Estimating
All of these thoughts about preparing and planning kicked in for me after I read an idea for a lesson in Chapter 5 of Tracy Zager’s new book, Becoming the Math Teacher You Wish You’d Had. The chapter includes a section on strategies for teaching students to check for errors. One suggestion is from Jennifer Clerkin Muhammad, who teaches fourth grade in Boston. Tracy writes that a dominant theme in Jen’s room is that she pushes students to think about whether answers make sense. Jen has instituted a routine: “I think ___ is unreasonable because __________.”
Tracy describes the routine in action: Jen had a student, Ethan, post a problem: 6739 ÷ 47. He gave his classmates time to think about what the answer might be and write their estimates on their mini-whiteboards. Then Ethan projected the estimates so everyone could see them: 130, 150, 155, 33, 597, 500, 1524, 2350, 2600, 750, 6700, and 372. He gave his classmates time to write about estimates they thought were unreasonable, and then he led a class discussion.
Tracy points out that an important aspect of this routine is that figuring out the exact answer is not the goal, but rather the focus is on making estimates. In a way, the estimates are the answers, rather than preludes to the “real” answers. The section includes information about what Jen did next to help her students see the value of estimates when they’re working on problems where getting accurate answers is the goal.
After reading this section, I put the book down and thought, “Whoa!”
How I Prepared and Planned to Use the Lesson
Before I could even begin to think about having a student lead a discussion like this, I wondered how I might lead this routine. My mind stumbled about as I thought about how I might prepare and plan to try this lesson, or some version of it, with the fifth graders I’ve been working with. They were learning about division and were having difficulty with greater numbers, especially with two-digit divisors. I wondered how this idea might help.
Then I thought about the range of the estimates Jen’s students came up with, from 33 to 6700. I wondered if it was typical to get this sort of spread and I wondered what happens when students don’t come up with such a range of estimates for discussion. I wondered how Jen first introduced the routine to the students and if, for example, she discussed with them how close an estimate needed to be in order for it to be reasonable.
I wished that I had read about this routine when we were working on two-digit multiplication. Then I thought that perhaps I should try a similar lesson with multiplication, so that the students would be on more solid mathematical footing. This could also be useful for emphasizing for students the relationship between multiplication and division and how multiplication can be useful when thinking about division.
I wondered what multiplication problem might be a good entry point, something the students couldn’t figure out mentally but could engage with.
Then I thought how I might introduce the activity. I know that when we try something new in class, there’s typically a period of confusion until students become clear about what’s expected. I try and plan carefully to avoid or at least minimize confusion, not with the mathematical thinking but with the routine of what’s expected. Here I wondered about what sort of scaffolding with the routine would provide them access. It was clear from the description in Tracy’s book about Jen’s class that this wasn’t the first time the class had engaged with this routine. How did it come to be a successful routine that could actually be led by a student?
What I Decided to Do
OK, here’s what I decided. I’d choose the problem, provide a collection of estimates, and then give the class the challenge of deciding which of the estimates were unreasonable. In a way, this seemed more teacher-directed than what Jen had done, where Ethan chose the problem and asked his classmates for estimates. But it seemed like a good beginning experience―for me and for the students.
I tinkered with problems and decided on 19 x 36. My thinking: The factor of 19 is close to 20, which could help the students use their understanding of multiplying by 10 and multiples of 10. They could relate the factor of 36 either to 35 or 40, also useful. While a few of them could figure out the exact answer mentally, it would be enough of a stretch for most of the class.
Then I figured out that the product of 19 x 36 was 684. (Yes, I used my calculator.) I jotted down possible estimates.
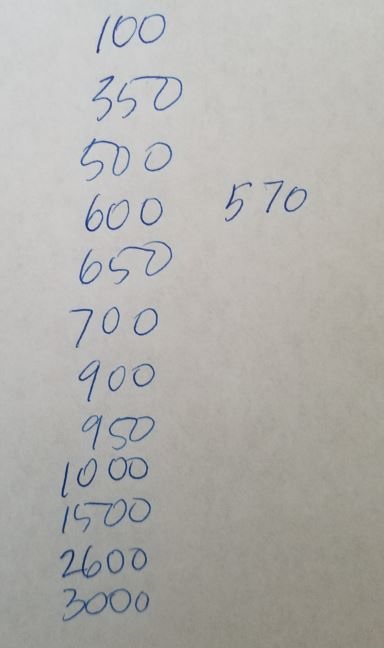
These estimates were multiples of 10 from 350 to 2600, about half too small and half too big, some close and some not at all close.
I was feeling pretty good about this plan and curious about how the students would respond and interact.
Planning Logistical Details
Then I went on to think about the specifics of presenting this to the class. I decided that I’d write on the board 19 x 36 = and above it I think ___ is unreasonable because . . .. I’d check to be sure they knew what unreasonable meant. (Hmm, I thought, I’d better have an example at the ready to illustrate the meaning of unreasonable. Maybe something like writing the number 1 in the blank as a possible product.)
I’d tell the students that I was going to write numbers on the board and ask them to think about which of them would be unreasonable estimates for the product of 19 x 36. Then I’d ask them to choose one estimate that they thought was unreasonable and think about how they’d explain why. I’d have them talk about their ideas with partners, and then I’d lead a discussion.
OK, I was set.
Or was I?
More Preparing and Planning
I began to think more about the students. I knew that a handful would dig in immediately and most likely be interested and successful. (They would be the same handful that usually is.) But what about the students who have weaker number sense, or are more timid about taking risks? How could I give them support so they also would have access?
Maybe I should start the lesson with a number talk that I thought they could all engage with. Maybe multiplication problems that they could solve mentally and explain their reasoning, similar to other number talks we’ve had. I could choose problems that could be useful for evaluating the possible estimates I planned to present for 19 x 36. So . . . what problems should I give them? I made a list.
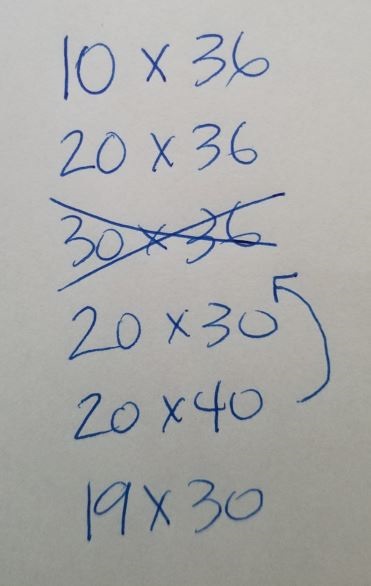
Here’s the list I jotted down. I crossed out 30 x 36 because I didn’t see its value for the problem of 19 x 36. I moved 20 x 40 before 20 x 30 because it seemed that 19 x 30 following 20 x 30 could encourage using the distributive property.
Reviewing My Plan
I reviewed my thinking, sort of a last run-through before teaching.
(1) I’d begin with a warm-up number talk using the above string of five multiplication problems.
(2) Next I’d pose the activity of identifying unreasonable estimates. After writing on the board 19 x 36 = and the prompt I think ___ is unreasonable because . . ., as the students watched, I’d write a dozen estimates. (I wrote possibilities on a list, choosing numbers that were less than and greater than the actual product.)
(3) Then, with the Think-Pair-Share routine that the students were familiar with, I’d have them first think by themselves about the possible estimates, next talk with their partner (with their “shoulder” partners not their “across” partners), and then I’d have them share ideas in a class discussion. I’d record the reasons they shared for labeling estimates as unreasonable, similar to the way I recorded their ideas during the number talk.
(4) If there was still time after this, I’d lead another number talk using a string of division problems. I thought that would help with preparing them for a similar experience with estimates for a division problem. (Oops, this made me realize that I’d better think about division problems that would make sense here.)
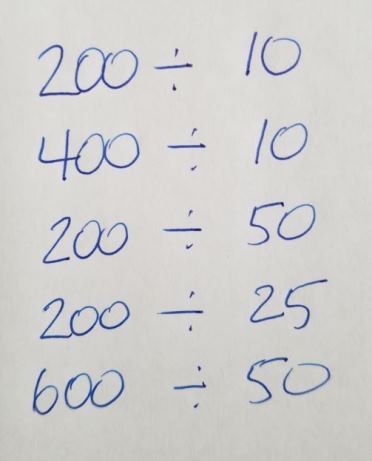
I brainstormed with Sara, their regular teacher, and we selected a string of division problems that we thought would be useful for later having them decide on quotients that were unreasonable.
How the Lesson Went
I was pleased. Here are the results from the multiplication number talk.

For the string of problems I presented in the number talk, I recorded the explanations students gave for how they figured out the products.
Then I presented the lesson as I had planned, writing on the board the problem (19 x 36), the prompt (I think ___ is unreasonable because . . .), and a dozen estimates.

I intentionally wrote the estimates on the board so that they were out of order.
After giving the students time to think and then talk in pairs, I led a discussion. As students presented, I wrote their explanations next to the product they identified as unreasonable. At the end, two boys announced that they had figured out the exact answer, and I recorded the answer and their explanation at the bottom. Here’s the board after the discussion.
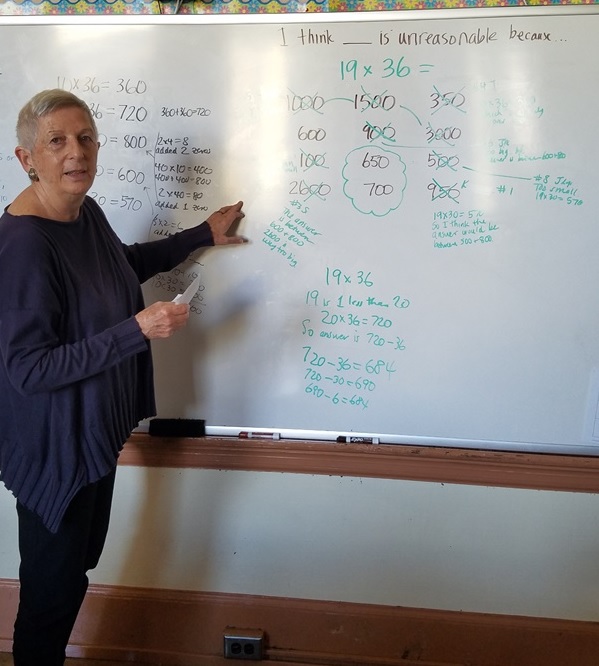
At the end of the discussion, the agreement was that 650 and 700 were the two most reasonable estimates.
To End Class
We still had about 10 minutes left in class, so I launched the other number talk with the string of division problems Sara and I had talked about.

As with the previous number talk with multiplication problems, for this string of five division problems, students figured out answers and explained their reasoning.
What Next?
After class, Sara and I talked about where to go next. We had several goals in mind. One was for students to record their reasoning in writing. We’ve learned that it’s important to have students do individual work that can help cement their understanding and reveal their thinking to us. We decided that it would be good to use the same routine of deciding on unreasonable estimates in the context of division. We tinkered with possible problems and decided on 3739 ÷ 47 and we identified a dozen possible quotients: 5, 10, 20, 25, 30, 40, 75, 80, 100, 150, 200, 500.
The next day, Sara presented the lesson, repeating the routine I had used for the investigation of the multiplication estimates. Well, she changed the routine just a bit, but it was a variation that students had experienced in other lessons. After presenting the problem, Sara told the students that they had three minutes of quiet time to think on their own. (Sara timed three minutes on her phone.) Next, she had one student share an idea about why one of the possible quotients was unreasonable. Then, she asked students to work with their partners to analyze the other estimates, but to record their ideas individually.
The experience took the rest of the class period. See the work below from two students’ notebooks. The next day, Sara planned to have them do a gallery walk to look at each other’s work.
Sara’s sent me a message after the lesson: The students like to think through things when they feel they can be successful. These lessons were the perfect combination of them having to think on their own but also knowing they at least had a way to start. It was the perfect balance of using the tools they’ve learned in a new challenge.
Samples of Student Work

As most of the students did, this girl relied on multiplication to make decisions about the quotients.
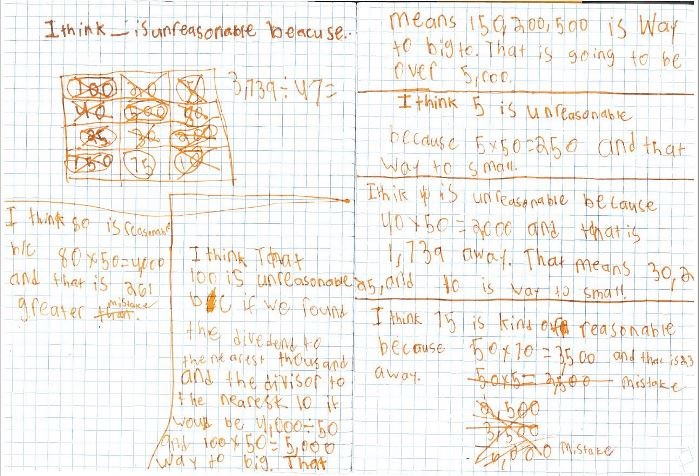
This student wrote “mistake” when he wanted to change his mind and revise his thinking, a protocol Sara has them use so that their work gives her as much information as possible about their thinking.


Do you use Estimation180 in class at all? I’ve just recently heard about it and am wondering about its value from teachers who implement it?
I have learned to value number talks greatly! As a math coach, I demonstrated a number talk in a 5th grade classroom (Is the fraction larger than 1/2?). In 10 minutes, the teacher looked at me and said that she had learned more about her students in that time than she had in days. She had 2 students who clearly still had misconceptions about the concept of a fraction; she had no idea!
Love the reordering to try to enhance the concept of using the distributive property!
I haven’t used Estimation 180 . . . yet. Thanks for your comment.
Marilyn, you’ve aways been so generous about allowing us to learn from your thought processes. I remember reading years ago a vignette you wrote about a lesson that did not go well and what you learned from it. I thought “wow! How brave and helpful!” This is another powerful story. I will use it with teachers I work with. Thanks for keeping our learning going.
That is a whole lot of work and love!
Dear Marilyn:
Interesting article about planning and preparing to teach math but also an important primer for leading a productive life.
Thank you!!!!
I love the idea of using number talks to help introduce a concept for a lesson. I did this for teaching estimation. I also found it really helpful when working with mathematical practices, such as looking to see if an answer is reasonable, during math talks. It gives students the chance to engage in math discourse and explain their thinking.
Marilyn,
Wow! Your dedication and thoughtfulness into your lesson planning is amazing and inspiring. You are so right — we spend so much time describing our lessons, but rarely share the thought process that goes into the planning. I’m excited to follow your blog, because I want my students to enjoy math class — something I never did. Thank you for sharing your planning! I’m going to try your number talks with my class.
Dianne Kiedrowski
Marilyn,
This blogpost is wonderful and generous and I loved reading it. (Sorry it’s taken me a few days to comment–sick kids the week before NCTM makes for a maxed-out Tracy!) I think your new stance–of making your thinking transparent during the preparation and planning–is so helpful for readers. I hope to hear more!
You’ve made me realize one thing I didn’t make clear enough in the book is that Jen had taught the routine many times herself before having a student lead it. For sure. And like you, her earlier ventures were with mathematics on surer ground for her students, and more scaffolded. I don’t know if she did any number strings. That’s a great idea and I was glad to see it work out.
One possible direction these conversations go is into empathetic error analysis, if that phrase makes sense. For example, in Ethan’s example, a student said, “I think I know what the student who got 6700 did. I think they didn’t see the little dots on the division sign and they thought it was subtraction.” I think they’re probably right! I saw that exact phenomenon happen in an 8th grade last week when a student hadn’t put her glasses on yet. Anyway, it can become helpful to try to figure out what went wrong, and Jen encourages that sort of thinking. She maintains an uber-focus on reasonableness, however, so rather than going down the subtraction road too far, she’ll take a comment like that and redirect it back to reasonableness: “Oh! So if the person thought it was subtraction instead of division, something around 6,700 would have been a reasonable estimate, right? That wouldn’t raise any red flags. That makes sense.”
My favorite aspect of this routine is that she stops when she gets to a reasonable range of estimates, and students never are asked to calculate it exactly. She’ll say, “So if I found an answer somewhere in this ballpark, I’d think, yeah, that’s reasonable.” And then she moves on. I love Number Talks very much, but I think occasionally including this variant–where we estimate instead of calculate exactly and stop at the range–builds a wonderful habit with students. As you mentioned, Jen explicitly guides students back to reasonableness later on, when they are calculating exactly, so they connect and transfer this way of thinking. I wouldn’t want the habit to live only within the instructional routine.
One thing I noticed in the second piece of student work is good use of the word “because.” That’s a component of this routine to emphasize. With the first student, I’d ask for a “because” statement justifying “I think 500 is unreasonable” and “I think 10 is unreasonable.” In this discussion, every answer needs to have two parts. I like this little video about getting a second sentence: As you and Sara build students’ familiarity with this routine, you could make that second sentence a habit.
Thank you for taking these ideas and running with them and sharing your thinking with all of us. I’m honored and grateful!
Tracy
Thanks for your response and the encouragement to share more of this kind of thinking. I won’t be at NCTM this year (my first to miss in as long as I can remember, due to a family wedding). But in a way, writing the blog was the kind of preparation I would have made for a presentation. (Next year in Washington, DC>)
About your thinking that you didn’t make it clear in the book that Jen had taught the routine many times herself — I had assumed that, and also assumed from the report about Ethan that she had done it effectively to make the routine so accessible for her students. And, in a way, the thinking that I had to do to figure out how to use this idea with the class I’m teaching was so very valuable for me and for Sara as well. Even if you had included specifics about Jen’s preparation for the lesson you described, I still would have had to think through how to do it myself and for my class. So, I’m grateful for the opportunity it gave me.
When I taught the lesson, I had planned to stop at the range, as Jen did. But the two students who had figured out the exact answer were excited about sharing their strategies. Recording their thinking for others to see was one of those teaching decisions that we all have to make all the time, without knowing at the time if they’re the best or right choices.
About the word “because,” I no longer have to prompt students to offer what Phil Daro describes in the video clip you included as”getting a second sentence.” In the second piece of student work, the student use the “b/c” abbreviation that a student had come up with last year (I worked with them as fourth graders, too) as a shortcut when writing and many use it consistently.
Again, thanks for your comment. And for your book.
Thank you for this great post. I have been an admirer of yours for so long, and it is so interesting to see all the steps of your preparation. Thank you for sharing it.
The question that came up for me as I thought along side your preparation was what would I do for those students who always want to give me the exact answer right away? My 4th grade students have heard me say (at least a thousand times!) I am not really interested in hearing the right answer, but more interested in how you got there. However, I know if I gave this lesson today 4 hands would shoot up and try to tell me the exact answer and use that as an explanation for which answer was reasonable. Did you encounter this in your lesson? How would you address this in your class?
Thank you so much for this post. I know I will be reflecting upon it for sometime as I prepare and work with my class.
Kim
I have experienced the same problem you describe, of students who quickly want to give the right answer. Sometimes I sidestep that by asking the class to say the answer softly on the count of 3. I count, they say the answer (sometimes I hear more than one answer), I acknowledge the correct answer, and then we move on to having them share all of the different ways we could figure it out. In this way, I let them know that I’m interested both in the exact answer and in their ways of thinking. I wrote a blog post a few years ago where I did that. But the goal of that lesson was to focus on the exact answer and how we might figure it out. With this lesson, I chose a problem for which I thought that getting the exact answer wasn’t reasonable, hopefully to deter those four hands shooting up. The problem worked in that way for my class, until near the end when getting the exact answer was fine with me. If four hands had shot up immediately, then would have realized that I didn’t choose a problem that was too challenging to figure mentally and I’d have to go back to the drawing board. The lesson in Tracy’s book used a division problem that I think would solve the problem you describe. Good luck with your class, Kim, and keep me posted.
Thank you so much for your response.
Hello Marilyn and Kim-
One thing I have tried with Number Talks is after the 3 minute or so period in which everyone is thinking, I then ask and record all of the answers that students got. Some of them are correct, some are incorrect. At this point, the students don’t know which is the correct answer. After we discuss the strategies, the students begin to realize what the correct answer, and often, the students who have an incorrect answer, realize their mistake. Furthermore, the students who are correct are encouraged to continue thinking because they aren’t sure if their original answer was correct. I really like this technique because it encourages continued thinking and emphasis on process.
Hi Josh, This is a fantastic idea and solution to the students racing to share the right answer. I intend to use it. Thanks for sharing!
Hi Marilyn,
My name is Anuj Agarwal. I’m Founder of Feedspot.
I would like to personally congratulate you as your Marilyn Burns Math Blog has been selected by our panelist as one of the Top 100 Elementary Teacher Blogs on the web.
http://blog.feedspot.com/elementary_teacher_blogs/
I personally give you a high-five and want to thank you for your contribution to this world. This is the most comprehensive list of Top 100 Elementary Teacher Blogs on the internet and I’m honored to have you as part of this!
Also, you have the honor of displaying the badge on your blog.
Best,
Anuj
great post