What Was Rebecca Thinking?

This blog describes a lesson I recently taught that has my head spinning (in a good way). I realize that the blog is longer than most I’ve posted, but I wanted to include all the juicy details that help describe what actually happened during the lesson.

Allison teaches second grade in Mill Valley, CA. She’s participating in the district’s project to use Listening to Learn interviews to help inform math instruction. From interviewing her entire class, Allison learned that almost half of the students did not demonstrate applying the inverse relationship between addition and subtraction. (Read more specifics about this at the end of the blog.) Lynne Zolli and I used that information to plan a lesson. (Lynne and I developed Listening to Learn together.) We decided that I would teach the lesson, which would include showing a video clip of Lynne interviewing Rebecca, a student who applied the inverse relationship.

The Lesson Plan
We had a little less than an hour to spend with the class. Here’s what we planned:

1. Lead a number talk using the problem 7 – 3.
2. Show the video clip of Rebecca solving the same problem.
3. Discuss with the class Rebecca’s strategy of using addition to solve a subtraction problem.
4. Ask the students to use addition to solve two other subtraction problems: 31 – 15 and 52 – 25.
5. Give a written assignment: Use addition to figure out the answer to 100 – 45. We gave a second problem for those who had time: 102 – 53.

Step 1. Leading a Number Talk for 7 – 3
After Allison settled the children on the rug, I introduced Lynne and me, writing Ms. Burns and Ms. Zolli on the board.

Then I wrote 7 – 3, the problem I wanted the students to consider first.

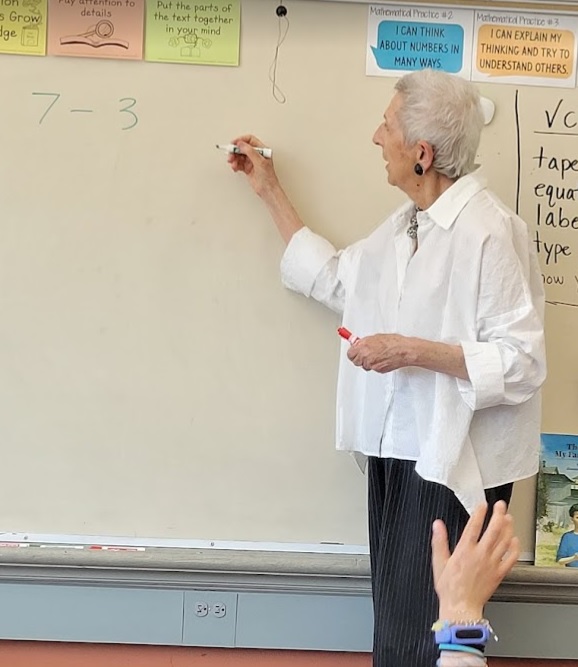
Hands immediately shot up. “I see that many of you know the answer to this problem,” I said. “Turn to your partner and check that you agree.”

Allison checked to be sure that each child had a partner, which was helpful as I planned to have them turn and talk several more times during the lesson. After the children had a moment to talk, I asked for their attention and told them that I was going to count to 3. “When I say ‘three,’” I said, “please say the answer in a soft voice.” I counted and heard a chorus of 4s. Having the students respond in unison established that 4 was the correct answer. Then we could move on to how they reasoned.

I told the class that I noticed that some of them knew the answer right away, without having to figure. I told them that now I was interested in learning how they could explain why 4 is the correct answer. I added, “Suppose a first grader was here who hadn’t yet learned that 7 minus 3 is 4. How could you explain how to figure it out? Raise a hand when you have an idea to share.”

I gave the children a few moments to think and then began to collect their ideas. I called on Ollie first. He said, “I know that 3 equals 2 plus 1. Then I do 7 minus 2 is 5 and then I do 5 minus 1 is 4.” I recorded Ollie’s idea on the board.

Ryan reported next. She used addition and said, “I know that 4 + 3 = 7 so 7 – 3 has to be 4.” I wrote on the board.

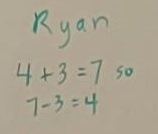
Leo shared next. “I did 3 plus 7 to get 10, then 3 + 3 is 6, and 10 – 6 equals 4.” I recorded the three equations. I realized, as I was writing, that I had no idea about how Leo was reasoning. Yes, he got the correct answer, but I wasn’t sure why he added 3 plus 7. Did he do this because these were the two numbers in the problem 7 – 3? Did it have something to do with making a 10? Why did he subtract 10 – 6? (It’s hard to teach and think at the same time.) I made the decision not to probe Leo for more information at this time and see what else I could learn during the lesson. Making this decision is one of the many decisions we have to make regularly as we teach. (See an addendum at the end of the blog for more about Leo.)

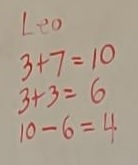
Next, I called on Jack. “I did it in my head,” he said. I asked him how he could explain to help a first grader know what to do. Jack answered, “I’d say that you can subtract 1 to get 6, then 1 to get 5, then 1 to get 4.” I recorded on the board and then posed a general question to the class about Jack’s idea, “How would the first grader know when to stop subtracting 1s?” Luke offered an answer, “You stop when you get to three 1s.” I recorded Luke’s idea.

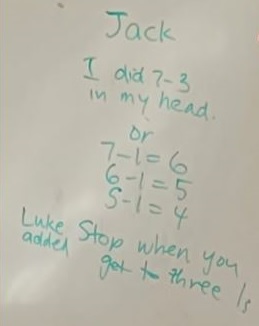
Andre was eager to share next. He said, “Four plus 3 is 7 so 7 minus 3 equals 4.” I recorded his idea, acknowledged that it made sense, and then pointed out that I thought he had the same idea as Ryan. Ryan nodded her agreement and so did Andre.

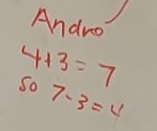
With ideas from six students on the board and no one else volunteering to share, I moved on to the next step in the lesson plan.

My Reflection We chose this problem from Interview 2 for two reasons. First, we’ve learned that answers are only the starting place and it’s important to move beyond them to also focus on how students reason. For that reason, we chose a problem with numbers within 10 so that the answer would not be a challenge or barrier. Also, we have a video clip of a student using addition to figure out the answer of 4 and we planned to show the video to the class to engage students with the inverse relationship between addition and subtraction. As I look back over what I had recorded on the board, I notice that five of the six children I called on were boys. That was a reminder to me to be sure to give girls adequate time to share their ideas. Also, a bit more about my reaction to Andre’s idea. Even though Andre expressed an idea that Ryan had already expressed, what came to mind for me is what I learned years ago from Eleanor Duckworth in her book, The Having of Wonderful Ideas. Andrew had expressed his wonderful idea. Eleanor’s claim is that when a child expresses an idea, even if it’s the same idea as someone else’s, it’s important to acknowledge the idea and let the student feel good about having it. She wrote, “The having of wonderful ideas is what I consider to be the essence of intellectual development.”

Step 2. Showing a Video of Rebecca Solving 7 – 3
Next, I told the class that I would be showing them a short video of a student named Rebecca being interviewed by Ms. Zolli, just as their teacher had interviewed each of them. I said, “You’ll see Ms. Zolli ask Rebecca, ‘What is 7 minus 3?’ Then you’ll see Rebecca think for a while to be sure she has the answer. And then you’ll hear her explain her reasoning. Play close attention because I’d like your help when I record Rebecca’s thinking on the board, just as I did for your ideas.” I pulled down the screen and brought up the video.

You can watch the video here.

The video is from before Covid and now, three years later, Lynne’s hair is longer and grayer. The children were fascinated and burst into chatter. They had lots to say. “Ms. Zolli looks different.” “Look, her hair is longer.” “It’s a different color.” “It’s gray now.” “She got older.” “Hey, she’s wearing the same watch.” “Her earrings are different.” “She’s wearing a different sweater.”

I called them back to attention, waited for them to get setttled, and then played the video. After Lynne asked Rebecca the question on the video, Rebecca thought for a while, gave the answer of 4, and then explained that she added 3 + 3 and then added on 1 more. The video is short, just over 30 seconds. I played it a second time to give students another opportunity to listen to Rebecca’s reasoning. They were now less distracted by Lynne’s appearance.

Next, I raised the screen so the students could see the board and asked, “What should I write to describe how Rebecca reasoned?” I called on Miriam. She said, “Rebecca added 3 plus 3 and then 6 plus 1. That’s how she knew it was 4.” Others nodded and I recorded.

My Reflection Listening to Learn includes an extensive video library that includes full interviews for each of the ten interviews and, in addition, almost 500 video clips of students answering individual questions. It’s a video treasure trove. Lynne Zolli and I are creating a series of suggestions for using LTL video clips in class instruction. We’re calling the lessons What Were They Thinking? In this case, we engaged students with What Was Rebecca Thinking?

Step 3. Discussing Rebecca’s Strategy
I then asked the class, “Did Rebecca reason in the same way as any of the ideas I recorded on the board? Turn and talk about this with your partner.” When I brought them back to attention, several students shared. The conclusion was that Rebecca’s idea didn’t exactly match any of theirs, but they noticed some things that were similar. Both Rebecca and Leo had added 3 + 3, but Leo didn’t add on a 1. Ryan and Andre also added, but they added 4 + 3, not 3 + 3.

I then told the students what I noticed. “Even though 7 – 3 is a subtraction problem,” I said, “I noticed that Rebecca solved it by adding. Leo used a combination of addition and subtraction. Ryan and Andre solved the subtraction problem only by adding, but they added 4 + 3 instead of 3 + 3.” By sharing this idea, I was preparing the students for solving other subtraction problems by using addition.

My Reflection Rebecca’s explanation revealed her ability to apply the inverse relationship between addition and subtraction. This is an important and powerful reasoning strategy. When students first encounter subtraction, they typically learn that subtraction means “take away.” This interpretation of subtraction relates to a problem like this: If you had $100 and you spent $86, how much money is left? However, when figuring out the answer, it’s important for students to know that they can reason either by adding or subtracting. That is, a student might think, “How much is 100 minus 86?” This can be recorded as 100 – 86 = __. But a student might also think, “What can I add on to 86 to get 100?” This can be recorded as 86 + __ = 100. The choice of when to subtract or add depends upon the numbers. For example, when mentally figuring out the answer to 100 – 98, it makes sense to add up from 98 to 100. For a problem like 100 – 2, however, it makes sense to subtract 2 from 100. The numbers matter! In  Interviews 2 through 5 from Listening to Learn, students have multiple opportunities to demonstrate applying the inverse relationship between addition and subtraction.

Step 4. Using Rebecca’s Strategy to Solve Two Other Problems
When planning the next part of the lesson, Lynne and I were interested in subtraction problems that would lend themselves to using addition to figure out the answer. We wanted problems that involved greater numbers but that still were accessible for second graders to solve mentally. We talked about Rebecca’s choice to add 3 + 3. We’ve found that adding “doubles” like 3 + 3 is typically easier for students, so we thought about problems that might suggest adding “doubles.” We decided on two problems: 31 – 15 and 52 – 25. Again, the numbers matter! Here we made the choice to include two numbers that we thought were easy for students to double: 15 + 15 and 25 + 25. (We thought that adding 25 + 25 would be accessible to the class because of their work on money.)

I wrote 31 – 15 on the board and said, “Turn and talk with your partner about how you could figure out the answer to this subtraction problem by only using addition.” After giving the students time to talk in pairs, I called them back to attention and called on Alexandra to share. She said, “I know that 15 + 15 is 30, and then you just add 1 to get to 31. So the answer is 16.” I recorded.

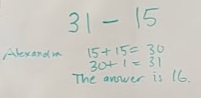
No one else volunteered to suggest another way, so I wrote the second problem on the board: 52 – 25. I asked the students to turn and talk with their partner about how to solve it using only addition. When I brought the class back to attention, I called on Stanley first. He began, “I added 25 + 7 and that gives me 32.” I wasn’t sure how Stanley was reasoning, but I recorded and listened. As I wrote before, it’s sometimes hard to teach and think at the same time. The good news is that in number talks I can focus on scribing what students say, which often gives me time (as well as giving time for the other students) to think about the reasoning. I recorded 25 + 7 = 32 and Stanley continued, “And 32 + 20 makes 52.” “And the answer?” I asked. “It’s 27,” he said.

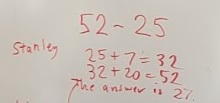
After Stanley explained, others put their hands up. More students were willing to share now than for the previous problem. This might have been because Stanley showed a different way to use addition, without relying on a “double.” Or maybe it was because the students were now more comfortable with a problem like this after their initial experience with 31 – 15.

Wren explained next and she did what I had expected—she added 25 + 25 and then added 2 more. Amelia said that was what she did, too, and I added her name to Wren’s explanation.

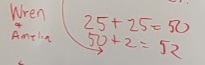
Andre offered a different idea. “I did 25 + 2 is 27, and I know that 25 + 27 equals 52.” I didn’t probe Andre about how he knew that 25 + 27 equaled 52. Ah, me, another missed opportunity.

Isla also volunteered to share. She said, “I know that 50 + 25 is 75.” She added, “And I know that 75 + 2 is 77.” Isla then scrunched up her face, not sure what to do next. I commented, “I see that you’re adding, as I asked you to do, but you’re not sure how these equations help with solving 52 – 25. Take some more time to do that. Remember, you can always change your mind to another explanation.”

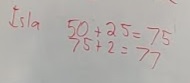
Leo volunteered next and explained that he “thought of jumps” on a number line. He began by adding 25 + 20 to get 45, next 45 + 5 to get 50, and then 50 + 2 to get 52. Finally, he added 20 + 5 + 2 to get the answer of 27.

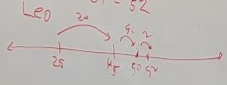
My Reflection There’s much to reflect on here. First, back to my being surprised, really startled, when Stanley started with 25 + 7 to figure out the answer to 52 – 25. I intentionally hadn’t mentioned anything to the class about Rebecca’s using “doubles,” but I confess that I had anticipated that was what they were going to do. I was so dialed into thinking about starting with 25 + 25 that I wasn’t sure where Stanley might be going in his thinking. Ah, the danger of preconceived ideas. This was a good reminder for me to listen not for what I expect to hear, but instead to listen to how the student is thinking. Also, about Isla, I wasn’t sure where she was going when she added 50 + 25 and then 75 + 2, I couldn’t see how these would help with figuring out the answer to 52 – 25. Neither could Isla, it turns out, so I accepted her thinking and encouraged her to revisit the problem.

Step 5. Giving an Individual Assignment
I felt that the students were now prepared to try an individual assignment. I was interested learning how each of them would tackle another similar problem—solving a subtraction problem only by using addition. Individual assignments are another valuable and important way to assess students’ learning. Again, Lynne and I had thought about problems ahead of time. We settled on one problem for all of them to do, and an additdional problem for students who had extra time. I explained this and wrote the two problems on the board.

I left all that we had done on the board so students could refer to anything that might be of help.

There was the typical transition time as students went from the rug to their desks, found pencils, and settled into the work. Some dug into the problem, others needed help getting started, some needed encouragement, others needed a jump start.

Analyzing the Student Work
Of the 17 students in class, nine solved the problem and successfully recorded addition equations to explain their reasoning. Another student solved the problem first by subtracting and then by adding, using an open number line both times. One student solved the problem by using 100 – 50, which he knew was 50 because 50 + 50 = 100 (which is how he used addition), and then by reasoning that 100 – 45 would have to be 5 more, or 55. Six tried but were unsuccessful or didn’t write anything on their papers.

Of the eight students who successfully added to figure out that 100 – 45 = 55, their papers revealed four different ways they reasoned. Four students started by adding 45 + 45. Then they added 90 + 10 and finally added 45 + 10 to get the answer of 55. I included two examples below to show two ways that I’ve typically seen students record. Both represent their reasoning, but Leo’s representation isn’t mathematically accurate because 45 + 45 is equal to 90, not to 90 + 10, as he wrote. This is a common error that doesn’t trouble me with second graders. That said, I think it’s good to encourage students to write a separate equation for each step of their thinking, as Ollie did in the second example.

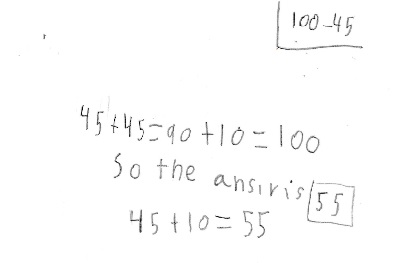
Two students started by adding 45 + 5 and next added 50 + 50. Then they added 50 + 5 to get the answer of 55.

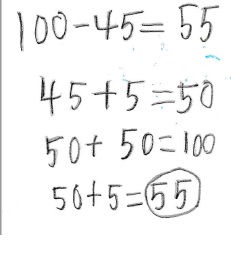
Max began in a different way, first adding 45 + 50. Then he added 95 + 5 and finally combined 50 + 5 to get the answer of 55.

Miriam did four additions—45 + 20, 65 + 20, 85 + 10, and 95 + 5. Then she combined the four numbers she added, 20 + 20 + 10 + 5, to get the answer of 55.

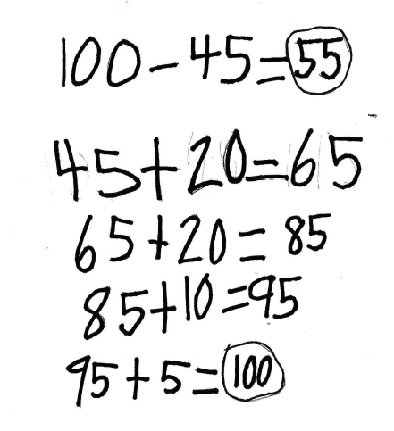
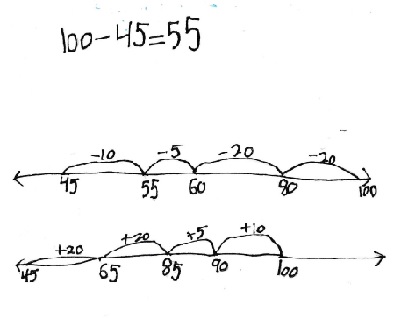
Mae used an open number line. She solved the problem twice, first by subtracting and then by adding (+20, +20, +5, and +10). She added the same numbers that Miriam did, but in a slightly different order.

Jack used addition in a roundabout way. First, he subtracted 100 – 50, which he knew was 50 because he knew that 50 + 50 is 100. He then reasoned that 100 – 45 would have to be 5 more, or 55.

Back to Leo
We have evidence of Leo’s thinking for solving three different problems during the lesson. First for 7 – 3, here’s how Leo reasoned:

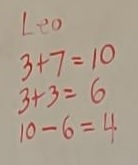
To solve 100 – 45, here’s how he reasoned:

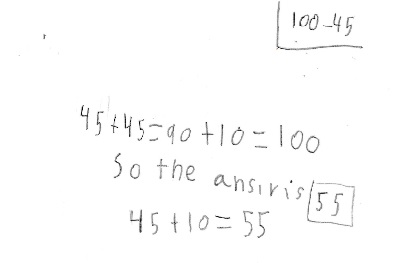
To solve 102 – 53, Leo provided two different ways to reason:

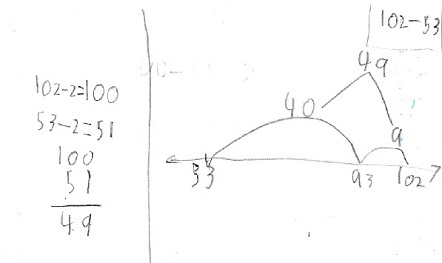
Leo’s work is testimony to his impressive flexibility with computing. For 7 – 3, Leo added 3 to each of the numbers in the problem, changing the problem to 10 – 6. This method is sometimes called “constant difference” and sometimes called “compensation.” It’s a useful strategy. For 100 – 45, however, Leo followed the instructions for the assignment and added to figure out the difference. Then, for 102 – 53, he showed two solutions. It’s not clear which he did first. On the left, he compensated as he did for 7 – 3, here subtracting 2 from each number in the problem to change it to 100 – 51. (It seems that 10 and 100 are “go-to” numbers for Leo.) On the right, he also added up on a number line, adding 53 + 40, 93 + 9, and then adding 40 + 9 to get the answer. To Lynne and me, Leo’s work was a big WOW. It’s a reminder of how important it is to give students a variety of experiences so that they have the opportunity to develop the kind of flexibility that Leo demonstrated.

Final Reflections
There’s rarely (if ever) only one way to reason to solve a problem. For this assignment, we gave the constraint that the students use addition to solve a subtraction problem. The goal was to help students see that addition and subtraction are related and to have experience applying the inverse relationship between the two operations. At the same time, we keep in mind that we want to accept and encourage students to tackle problems in ways that make sense to them and that are appropriate for the numbers at hand. Here’s another quote from Eleanor Duckworth that continues to inspire me:

“Teachers are often, and understandably, impatient for their students to develop clear and adequate ideas. But putting ideas in relation to each other isn’t a simple job. It’s confusing and this confusion does take time. All of us need time for our confusion if we are to build the breadth and depth that give significance to our knowledge.” ― Eleanor Duckworth, The Having of Wonderful Ideas and Other Essays on Teaching and Learning

Lynne and I spent a good deal of time revisiting the lesson, reviewing what was recorded on the board, poring over the students’ papers, and thinking about what we might do next with the class. (More about that in a future blog.) Neither of us currently is a full-time classroom teacher. We have the luxury of time that we know classroom teachers don’t typically have, and we know that analyzing lessons in this way is time-consuming and neither reasonable or doable on a regular basis. However, we offer our investigation to share how we approach thinking about a particular experience and also to provide encouragement for doing so whenever there’s time. We’ve found that digging in as we have, especially doing so with a colleague, can be revealing and rewarding.

The Back Story for This Lesson
As I wrote at the beginning of the blog, Allison teaches second grade in Mill Valley, CA, and participates in the district’s project to use Listening to Learn interviews to help inform math instruction. Allison gave Interview 3 to her class, which focuses on adding and subtracting within 20. Here’s the Class Report that the Listening to Learn digital tool generated:

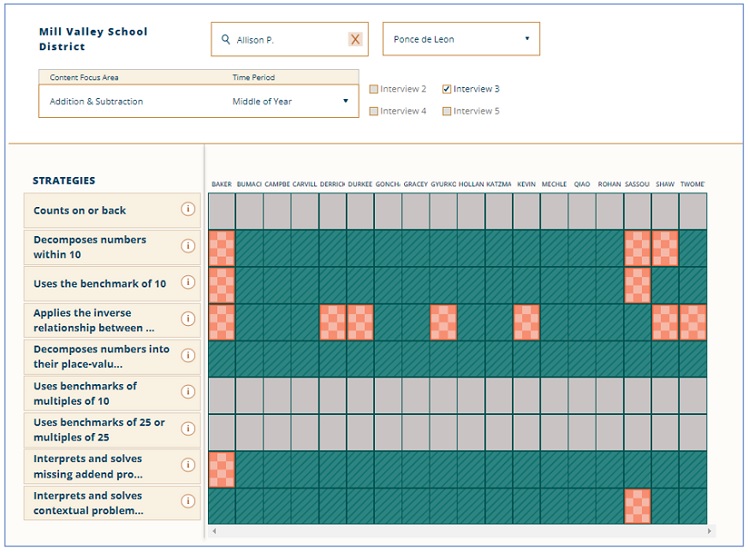
The reasoning strategies we want students to develop and use are listed on the left side of the report. Green indicates that students demonstrated the strategy and orange indicates that students did not demonstrate it. (The rows with grey squares are for strategies that relate to addition and subtraction but don’t pertain to this particular interview that focuses on numbers within 20.)

What Were They Thinking? Lessons
Lynne and I have been creating lessons which we’re calling What Were They Thinking? In these lessons, we show a video clip from Listening to Learn to the class. When we interview students to learn how they reason, we’re often surprised, intrigued, and delighted by their explanations. Not only does their thinking stretch our thinking and inform our instruction, we think the videos help students learn to listen to how their classmates reason. We believe that understanding how others reason can expand students’ own thinking. For other similer blogs with fourth and fifth grade classes, check out What Was Nathan Thinking? and What Was Jonah Thinking?