A long-standing instructional practice has been to teach students how to add, subtract, multiply, and divide and then, after students have learned to compute, give them word problems to solve. I think of this instructional practice as putting the cart before the horse.
Instead of teaching computation first and then assigning word problems, word problems are effective vehicles for helping students 
develop understanding of the meanings of operations. Word problems build understanding of when and how operations are useful. This focus on understanding is important before students focus on learning and practicing procedures for calculating.
As an example, here I focus on multiplication and present “Multiplication Stories,” a lesson that I’ve taught many times to third graders. Also, in this post I’m experimenting with a format that’s new for me, a video that’s basically a Power Point presentation that I’ve narrated to describe how the lesson can unfold in a classroom. The video runs 7:36 minutes, and I’m interested in learning if this format is useful for helping communicate a teaching idea.
Here’s some background information. Before I teach the lesson in the video, I usually teach three other lessons to the class―”Things That Come in Groups,” “Circles and Stars” (I don’t know how I ever taught multiplication without this game), and a lesson based on the children’s book, Amanda Bean’s Amazing Dream. (These lessons and the one on the video all appear in Teaching Arithmetic: Lessons for Introducing Multiplication, Grade 3.)
Take a look. And then please let me know if you find a video like this useful for describing instruction. If so, I’m interested in hearing why and/or what suggestions you have for me to improve it.
Note: This is the lesson that sparked an astonishing discovery from Lydia, a third grader, that I described in my previous post, Where’s the Math?
I like your video. I am now retired but I think it would have been a great illustration when I was trying to coach 3rd grade teachers with good teaching methods. It would also be an excellent tool for my pre service students to watch and listen to.
I suggest you do more of these.
Good morning! I LOVE this way of professional development! I am an instructional coach for math/science and have been a user of your methods since (gulp) 1990ish. All of the problem solving and common core and all the rest that people call “new”, I know it is what you have been trying to get changed since “Problem-Solving: the Math Challenge of the 80s” (I still have that book). I can’t afford to send my teachers to the week long training I took back in 1995, but this format will give them a chance to understand some of the replacement units you have written in a PDR format. This is POWERFUL stuff. Thank you for continuing to support math teachers!
This could be an amazing tool of empowerment Marilyn!
Many times I find teachers shy away from reading a lesson plan due to time constraints and the ability to connect it to their class. Your video allows teachers to visualize what’s happening, which is difficult to do with a typed lesson. By offering this as a planning tool, teachers are able to identify and uncover the explicit teacher moves you made during the task. (I like when…, Notice how…, I wouldn’t have done that when…, etc…) And because it’s not someone they know personally, they will be more transparent in their feedback.
I’m specifically interested to see if your transition between the tasks will be emphasized during collaboration. There were a lot of layers embedded within the 1st task that teachers may not have recognized without the use of the 2nd task. Nice move! By purposefully modifying the 1st and 2nd task, it ensured that the lesson was still centered on the conceptual development of multiplication and not just regurgitating what they previously did. The practice comes later:-)
Going forward: This seemed like a manageable task to capture in a quick video and I’m wondering if it’s doable with larger tasks. The video is short, clear, and concise but I’m afraid if it gets any longer it will lose its appeal. I’m looking forward to see how you flesh this idea out and in the meantime, I’ll give it a whirl myself.
Really enjoyed how clear and concise the lesson (your video) was. It’s much like your books, but WAY quicker and easier to access. I can’t think of how to improve it at the moment 🙂 thanks a lot!
I think the video is a great addition to your blog. Listening to your dialogue refreshes my memory of great teaching and it helped clarify your lesson. Thanks for your work to include that.
I really enjoyed your video. I would definitely like to watch some more! Thank you 🙂 Tales from a Fourth Grade MathNut
Brooke
I found the video an enormous help. I can never seem to generate that kind of discussion without seeing and/or hearing it modeled first. I especially liked seeing the student answers – exemplars always help me to see what I’m aiming for – and my students too. I liked to see what you were recording on the board – and how you labelled the sections to highlight the vocabulary. I can see how to apply these ideas in my lessons.
I will share this with my staff, because I feel it is very informative. I like the inclusion of the student work and I think the format works well. I agree with Connie that the concise and clear commentary are perfect for this day and age!
Thanks for this great video. I appreciate this format and am anxious to share it with my third grade colleagues!
Thank you….this was great…as a visual learner my self I would like to see some videos at younger year levels
Th video does good job of modeling all of the different approaches that students might take. The only part that seemed extraneous was the estimation. There was no dialogue about why this might be helpful nor any questions as to how students make their estimations. These seemed to be numbers just pulled out of the air. I noticed that this was not referenced at the end either. If this is part of a bigger picture that is addressed in another video or segment of the learning progression then a mention about this would be helpful. If not, then why is it included?
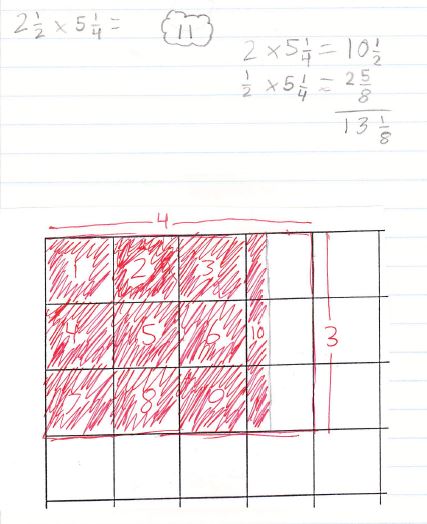
I just received a Tweet (@mburnsmath) asking about estimating and whether I always use this structure. I’ve changed my mind many times about when and how to ask for estimates, and what to do with the estimates that students suggest. I honestly can’t remember when I’ve ever listed the numbers as I did here with the multiples of 10. The best purpose it served was to spark Lydia’s thinking for her fascinating reasoning about 7 x 3 = 21 and 8 x 4 = 32 in my previous post. My current practice is to encourage estimates, have students write them in “wavy” clouds, and then be sure to discuss afterward how the estimates and answers compared. Here’s a sample of student work that led to an interesting class discussion about why 11 seemed reasonable but was, as Sam said, “Way off.”
I love the video format and think this is a great vehicle for sharing your expertise with us. Thanks for sharing actual student work as well. Keep it coming, Marilyn! I love this!!!
I very much appreciate the way you described situating this lesson after the other three lessons. I am wondering, for professional learning purposes, whether you might describe your thinking with respect to the students who shared their thinking. I know that the order of sharing was intentional on your part, and that means that you were observing the students at work, rather than “fixing the math”. I think this aspect of math instruction is the least visible to teachers and without it, the conversation about the problem falls into a giant share without organizational structure and the math is lost.
Good point. I intentionally look for a variety of ways that students reason and ask them to report. I often look for a mix of reasoning that does and doesn’t make sense. This is where teaching judgment is so very important, so that errors can be see as helps to learning. I’m clear in class that it’s OK to change your mind at any time, but it’s important to explain why.
Thank you for the video. If a teacher has not been trained to have students show their thinking in this way, watching a video with several representations can be very powerful.
Very nice! Thank you for sharing. I liked the format. It helped me to understand how I can use the lesson in my classroom.
Sweet package Marilyn. I’m sending it out as “summer reading” for incoming pre-service students to think about. Thank you.
High school math needs a Marilyn Burns.
“Oh, it’s fine for little children to reason their understanding, but by high school we have so much to cover. There’s not time for that.”
This leads to too little understanding and too many dropping out of math.
Oh, my! I absolutely love & appreciate the video! I’ve been a huge fan of yours for years so after I overcame my geekout (“Oh my gosh, it’s THE Marilyn Burns”), I was so delighted to see AND hear you talk out & demonstrate the lesson. For such a short video, I came away with a number of insights on ways to improve my instruction (i.e., how to reinforce vocabulary, summarize, etc.)! I believe pre-service and veteran teachers alike can benefit from these types of videos. Thank you so much. I look forward to future videos.
In your May 20 th blog, you mention the game “Circles and Stars”. Could you share how to play the game? Thank you!
The game of Circles and Stars is one of my all-time favorites. There are lots of references online. Here’s a link — http://teacher.scholastic.com/products/dothemath/pdfs/Sample_Teacher_Guide_Pt1.pdf — to a PDF that describes how I used the game in the Do The Math program:
Let me know if you have any other questions.
Hi Marilyn – I have just been viewing this video and found the concept behind it really thought provoking.. I too was interested in the circles and stars but the link is no longer accessible. Do you another way to access this resource.. Many Thanks
Circles and Stars is a game that appears in various places, both in books I’ve written and elsewhere. Here’s a link to a PDF. If you have questions, don’t hesitate to ask.
Loved it! Keep it coming!
I am a parent and stumbled upon your blog. I was never good at Math. Wish I had a teacher like you and probably then I would have fallen in love with Math. Thanks for sharing your knowledge and experience. It is an eye opener for me.