Charlotte’s Algebra 2 assignment was about rational functions. I don’t always remember the mathematics required for Charlotte’s homework, which was the situation this time. (My excuse is that, hey, I took Algebra 2 almost 60 years ago.) But when I’m initially stuck, I’m able to help by doing what I always did with math assignments—read the pages before the problems, look at the examples, and mirror what they did in them. I worried that this is resorting to “doing the page” not “doing the math,” but it helped jump-start my thinking.
The problems on this assignment asked students to find the domains of rational functions, identify the asymptotes and holes in their graphs, and then graph them.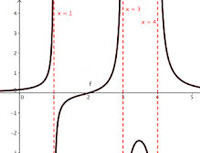 
I figured out the domains, asymptotes, and holes. Charlotte had already figured them out as well. But graphing? Oy. A note in the textbook’s margin suggested using a graphing calculator, which she doesn’t have. But then I remembered that Desmos has a free graphing calculator online. (Thank you, Desmos!) She looked it up and there it was. The online calculator helped her complete her work.
Why did I ever major in math? Well, my mother urged me to become a teacher and my grandmother urged me to become an accountant. 
When I announced that I was going to major in mathematics, both of them were satisfied. I think that math seemed to be a logical path to both of their career choices. Helping Charlotte reminded me of how I struggled in my college math courses, night after night, keeping in mind that I was pleasing my mother and grandmother, and reminding me that at least I didn’t have to write papers. (And look at me now, a writing fool!)
The last problem on Charlotte’s assignment had nothing to do with rational functions. It was a geometry problem to figure out the cost of materials for a tuna fish can, given its diameter and height, and the cost per square centimeter of the material. That I could do, and so could she. As we typically do, we worked on the problem separately, each with our own calculator, and then checked to see if we got the same answer. We did. I felt, well, triumphant. But rational functions? Not yet. Still, I experienced a weird kind of pleasure in helping her get the assignment done. It’s as if working on these assignments will eventually make me a better math person, that more math will seep into my brain. In a way, I think that’s true.
I was reminded of all the math I do understand—subtraction, fractions, multiplication, and much more—and how elementary students often feel about these topics the way Charlotte and I were feeling about rational functions. This experience strengthened my resolve to keep learning, just as we hope our students will. And to be sure that my teaching helped students do the math, not just the page. The next morning I went online and read more about rational functions. I’m on my way.
Charlotte had other homework, too. Her English assignment was to write a letter to a parent asking for something she really wanted, using ethos, pathos, and logos in the letter. The topic she chose: Please, May I Get a Dog? I wonder how many of those I-want-a-dog letters the teacher had to read the next day.
Hi Marilyn,
This post reminded me of helping my children with math as they entered high school. I went to the tutor with my oldest son – who really only needed a bit of support – but I decided to go with him so I could brush up on Alg to help the three others behind him! At that time, the school was using CPM -so I got a bit of a head start with a new approach. I remember several other parent friends who were completely at a loss with CPM and trying to hep their kids.
When my son took Alg 2 he struggled – at that time I was middle school principal and we had received an HP grant to provide ALG 2 to 8th graders through Academic Learning Systems – one of the first online curriculum for math. It was so successful at the middle school ( due to the outstanding teacher who had to differentiate for the 6 adv students he had his alg class). Anyway I used it with my highschooler and it was just what he needed. Ironically, his high school teacher had a really tough time accepting that I was helping my son pass his class using this curriculum…
Never a dull moment in our world but isn’t it great that we can still learn and support??
I enjoyed reading your blog!
Working with my adult students, we are still learning when it makes sense to say “use a graphing calculator to graph it.” If all a student does is punch it in and copy, then … well, I suppose at least it’s knowing how to use a tool (which they may or may not ever use again like this), but it feels too much like “doing the page. If they actually look at the graph and ponder its ups and downs and asymptotes and holes… that’s learning.
With the advent of the online graphing calculator (Yes, I use DESMOS too), I’ve decided that it’s more important to get good at using the graphing calculator than it is to graph manually. Graphing manually is important only to the extent that students realize the x -y relationship. Using the calculator, it’s much easier to alter the parabola and move it around the plane.
I’ve been hosting a series of parent workshops at an elementary school. On the first night the parents told me, “Without a textbook, how am I supposed to know how to help my child? I have no idea what these new terms are that my child is coming home with. I don’t know how to help them!” Common Core has added challenges for parents to support their children. To tell a parent, “Just trust the teacher” would be ideal but is unrealistic. This post reminds me to give credit to these parents for seeking out new learning. Even if some parents’ mentality is, if you can’t beat ’em, join ’em. Thanks Marilyn!